Tree Diagrams

What is a tree diagram?

A tree diagram is used to
- show the (combined) outcomes of more than one event that happen one after the other
- help calculate probabilities when AND and/or OR’s are involved
Tree diagrams are mostly used when there are only two mutually exclusive outcomes of interest
- e.g. “Rolling a 6 on a die” and “Not rolling a 6 on a die”
More than three outcomes per event can be shown on a tree diagram but they soon become difficult to draw and so lose their effectiveness
Tree diagrams are very helpful when probabilities for a second event change depending on the first event

How do I draw and label a tree diagram?

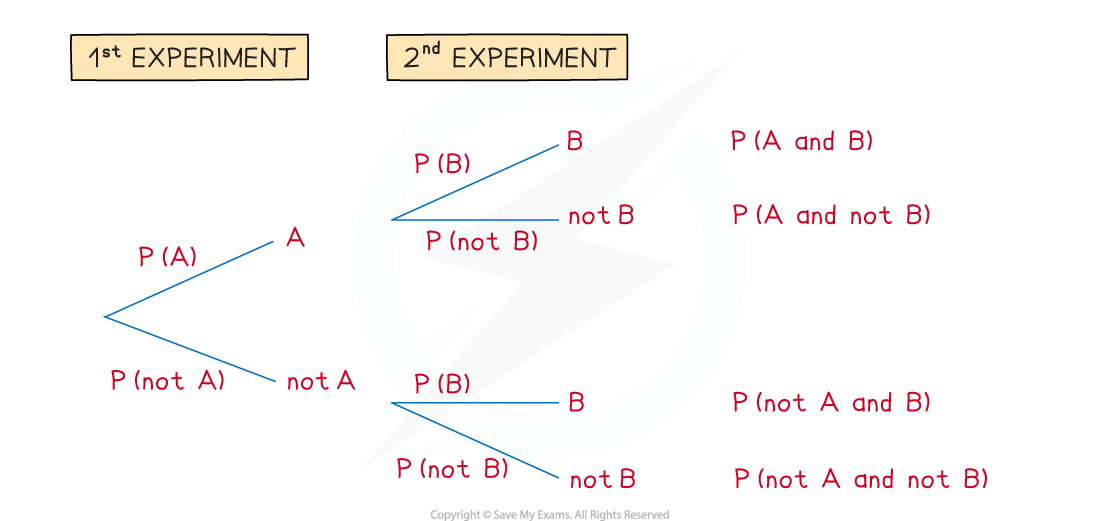
3-1-3-fig1-tree-setup

In the second experiment, P(B) may be different on the top set of branches than the bottom set
- this is because the top set of branches follow on from event A but the bottom set of branches follow on from event “ $n o t A$ ”
- e.g. This is most commonly seen in drawing one item at random, not replacing it, then drawing another
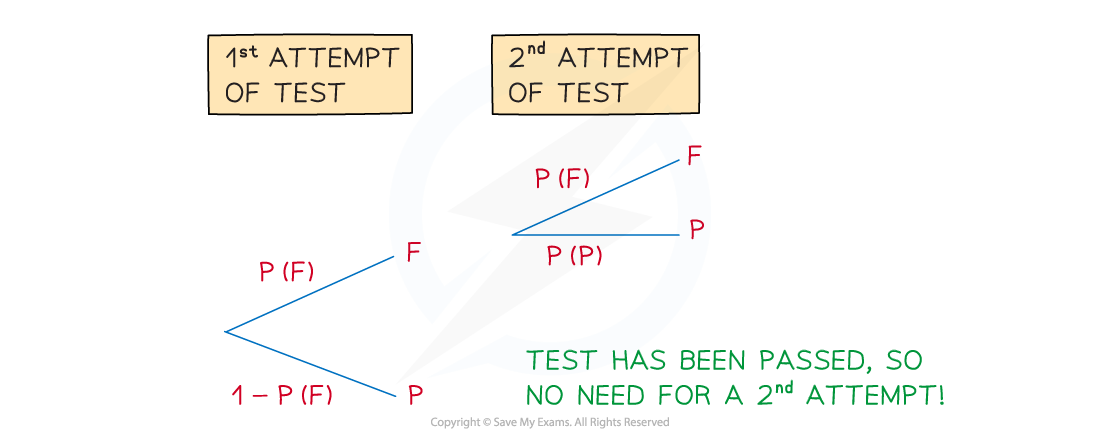
Sometimes a second branch may not be needed following a first event
- e.g. In aiming to pass a test (experiment) the event fail on the first attempt would require a second attempt but the event pass on the first attempt would not

3-1-3-fig2-pass-fail-tree

How do I solve probability problems involving tree diagrams?

Interpret questions in terms of AND and/or OR (See 1 Basic Probability)
Draw, or complete a given, tree diagram
Determine any missing probabilities; often using $1 - P (A)$ and considering
if probabilities change depending on the outcome from the 1^st experiment
Write down the (final) outcome of the combined events and work out their probabilities – these are AND statements
$P (A AND B) = P (A) \times P (B)$ (“Multiply along branches”)
- Do not simplify fractions yet – it’ll be easier to calculate with them later
  - you can of course use your calculator
If more than one (final) outcome is required to answer a question then add their probabilities – these are OR statements
$P (A B O R " n o t A " " n o t B ") = P (A B) + P (" n o t A " " n o t B ")$ (“Add outcomes”)
- This applies since all the (final) outcomes are mutually exclusive
- Note that $A B, " n o t A " " n o t B "$ are implied AND statements (for example AB means A and B)
When you are confident with tree diagrams you can just pull out the (final) outcome(s) you need to answer a question rather than routinely list all of them

Worked example

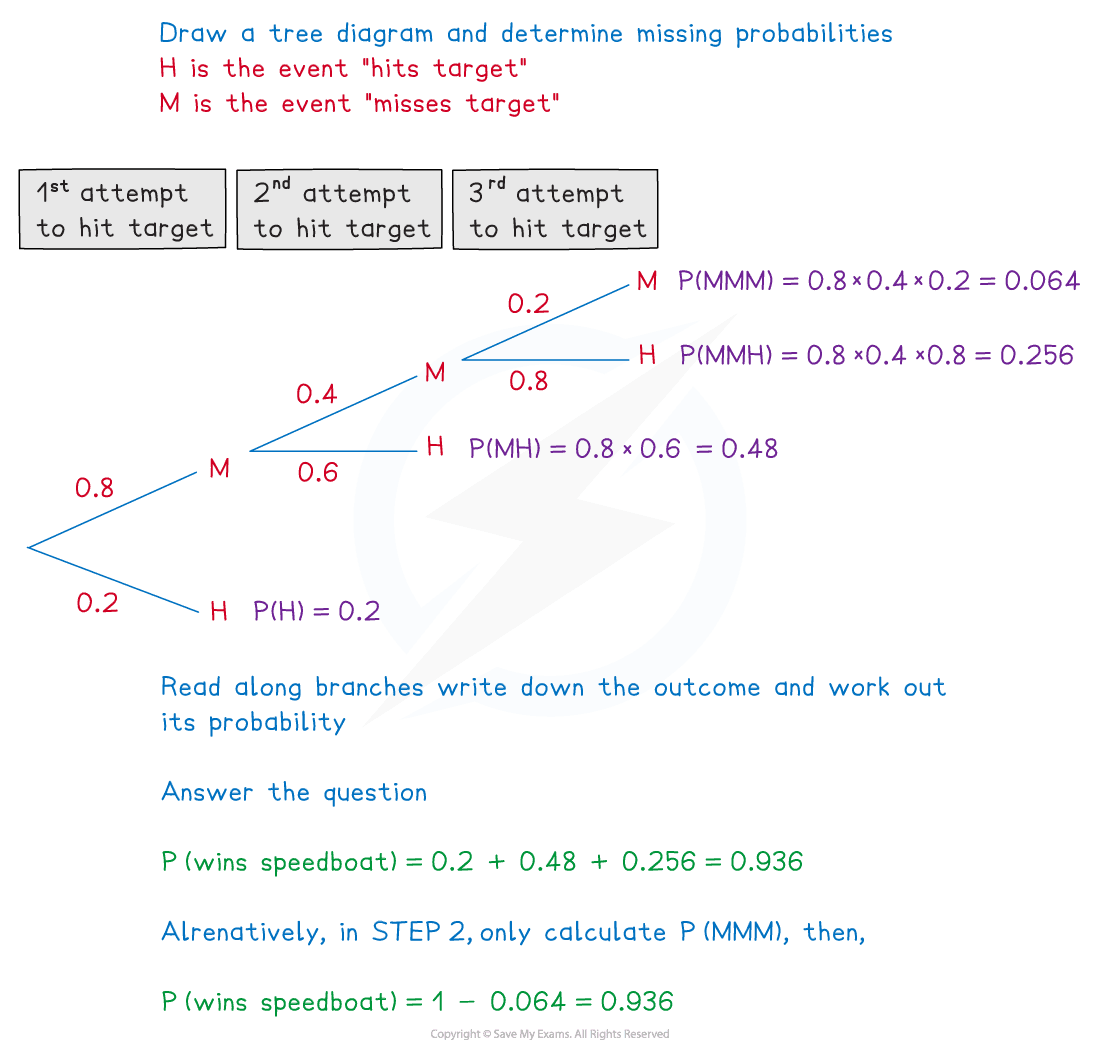
A contestant on a game show has three attempts to hit a target in a shooting game.
They have a maximum of three attempts to hit the target in order to win the star prize – a speedboat. If they do not hit the target within three attempts, they do not win anything.

The probability of them hitting the target first time is 0.2. With each successive attempt the probability of them failing to hit the target is halved.

Find the probability that a contestant wins the star prize of a speedboat.

3-1-3-fig3-we-solution

Examiner Tip

It can be tricky to get a tree diagram looking neat and clear first attempt – it can be worth drawing a rough one first, especially if there are more than two outcomes or more than two events; do keep an eye on the exam clock though!
Always worth another mention – tree diagrams make particularly frequent use of the result $P (not A) = 1 - P (A)$
Tree diagrams have built-in checks
- the probabilities for each pair of branches should add up to 1
- the probabilities for each outcome of combined events should add up to 1

Tree Diagrams (CIE A Level Maths: Probability & Statistics 1)

Revision Note

Tree Diagrams

What is a tree diagram?

How do I draw and label a tree diagram?

How do I solve probability problems involving tree diagrams?

Worked example

Examiner Tip

You've read 0 of your 10 free revision notes

Unlock more, it's free!

Join the 100,000+ Students that ❤️ Save My Exams

Author: Paul