Coefficient of Friction (OCR A Level Maths: Mechanics)

What is friction?

• Friction is a force between a surface and an object that acts to resist motion of the object across the surface
• On a horizontal surface with no forces acting on a stationary object other than gravity and the surface's normal reaction force, there is no force of friction
• If an additional force acts on the object parallel to the surface then friction manifests as a force in the opposite direction
  • Friction always acts in a direction parallel to the surface
  • Friction acts in the opposite direction to the resultant force that would cause the object to move parallel to the surface
  • Up to a certain limiting value (see next section) the force of friction prevents motion entirely
  • Beyond that limiting value friction is a force of constant magnitude in the opposite direction to the motion of the object

What Is the coefficient of friction?

• The magnitude of the frictional force satisfies the inequality:

F ≤ μR

where F is the force of friction, R is the normal reaction force between the object and the surface, and μ is the coefficient of friction between the object and the surface

• • If there is no friction then μ= 0 and the surface is smooth
  • Otherwise μ > 0

• The maximum possible frictional force is given by the equation

F_MAX = μR

where F_MAX is the maximum possible force of friction, and R and μ are as above

• F_MAX serves as a limiting value for mechanics problems involving friction
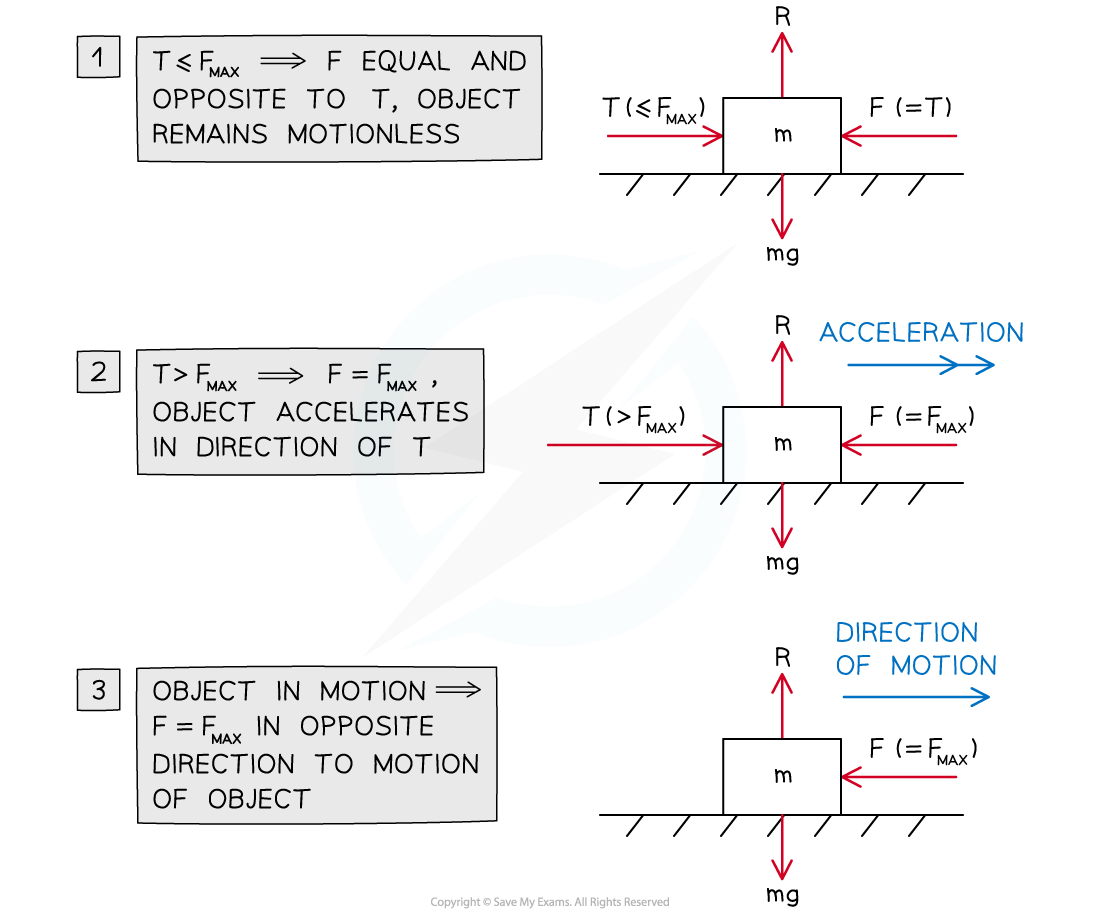
  1. If the object is stationary and the resultant of other parallel forces to the surface is less than or equal to F_MAX then
     • the object remains stationary
     • the frictional force is always equal and opposite to the resultant of the other horizontal forces
     • if the resultant of other horizontal forces is equal to F_MAX then the object is said to be in limiting equilibrium

  2. If the object is stationary and the resultant of other horizontal forces becomes greater than F_MAX then
     • the object begins to accelerate in the direction of the resultant force
     • the frictional force is equal to F_MAX with a direction always opposite to that of the object's motion

  3. If the object is in motion parallel to the surface then (regardless of any other forces) the frictional force will always be equal to F_MAX with a direction opposite to that of the object's motion

A box with a mass of 12 kg is at rest on a horizontal floor.  The coefficient of friction between the box and the floor is 0.7.  A horizontal force of 80 N is applied to the box.  Describe the force of friction in this situation and determine whether or not the box will begin to move.