Did this video help you?
Acceleration due to Gravity (CIE A Level Maths: Mechanics): Revision Note
Acceleration due to Gravity
What is acceleration due to gravity?

- If motion is vertical and no other forces are acting on a particle, the acceleration of the particle will be due to gravity
- Acceleration due to gravity is denoted by the letter g
- The value for gravity on Earth varies depending on location (the average value is 9.80665)
- Take g = 10 m s-2 unless told otherwise
- It is often easier to leave working in terms of g as it sometimes cancels out or it can be calculated at the end
- Some questions may ask for the answer to be left in terms of g
- Gravity will always act in the downwards direction towards the earth
- If you decide upwards is the positive direction, then a = -g
- If you decide downwards is the positive direction then a = g
- It is important to be clear about which direction you have decided is positive
Displacement or Distance?
- There is a difference between displacement and distance
- It is more pronounced in vertical motion problems
- Particles typically travel in both directions (up and down)
- What goes up must come down!
- Displacement, s, is a vector
- It is the position of a particle (at time t) relative to its starting position
- It only takes into account where the particle started and ended
- If a particle has returned to its starting position then its displacement will be zero
- Distance could be …
- … the distance from the start
(in which case distance is the magnitude of displacement)
-
- … the distance travelled
- … the distance from the origin
(the origin is not necessarily the starting position)
-
- … the distance from another particle
- Read the question carefully to be clear about which of these you are finding
How do I solve suvat problems involving gravity?
- Virtually the same as suvat in 1D for horizontal motion
- Follow the same steps and be careful with negatives
- You might have to spot that gravity is the acceleration by seeing the phrases:
- "falling freely"
- "projected"
- "thrown/dropped"
- A diagram is important to help make clear …
- … the positive direction of motion
- … the way in which acceleration relates to the positive direction
- … differences between displacement and distance
- Particles moving upwards will reach a maximum height
- At the maximum height, velocity is instantaneously zero, v = 0
Worked example
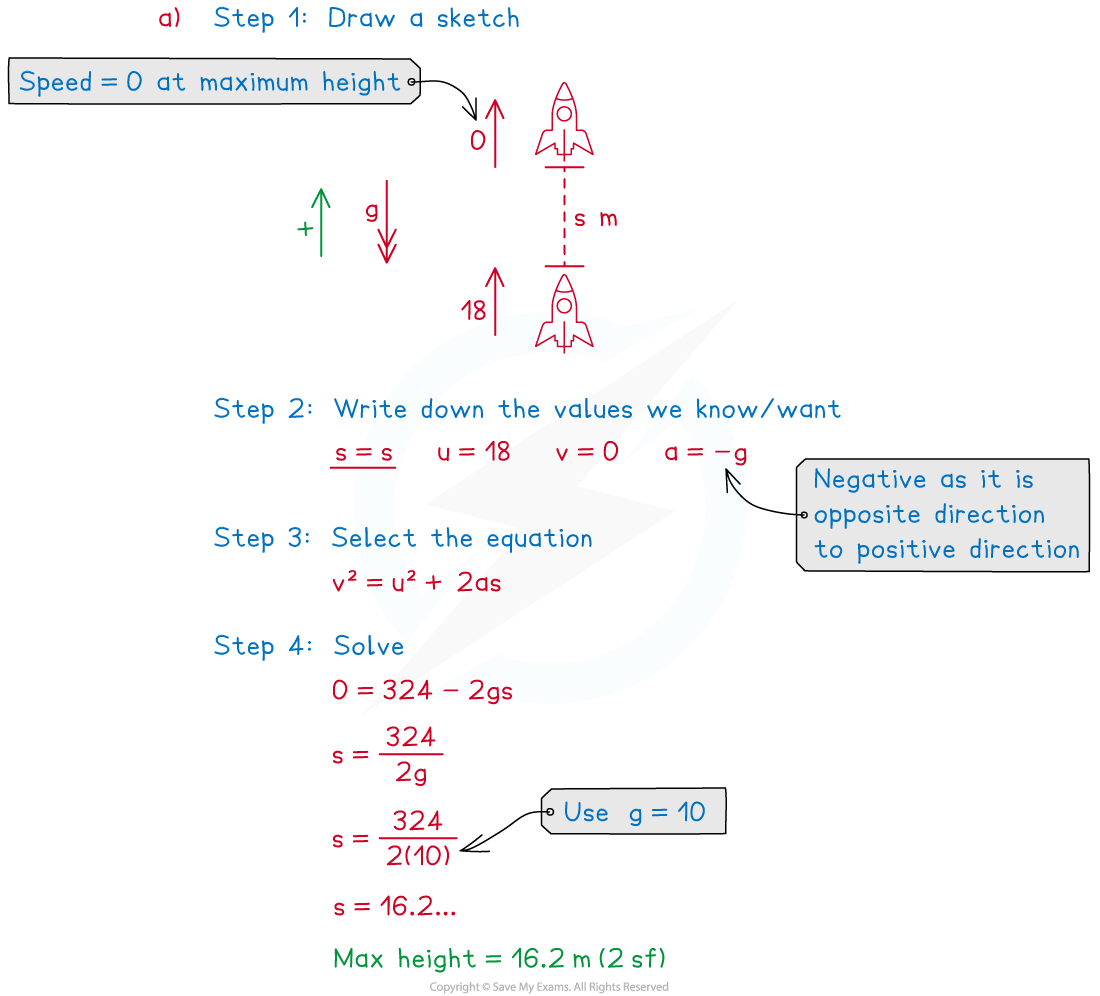
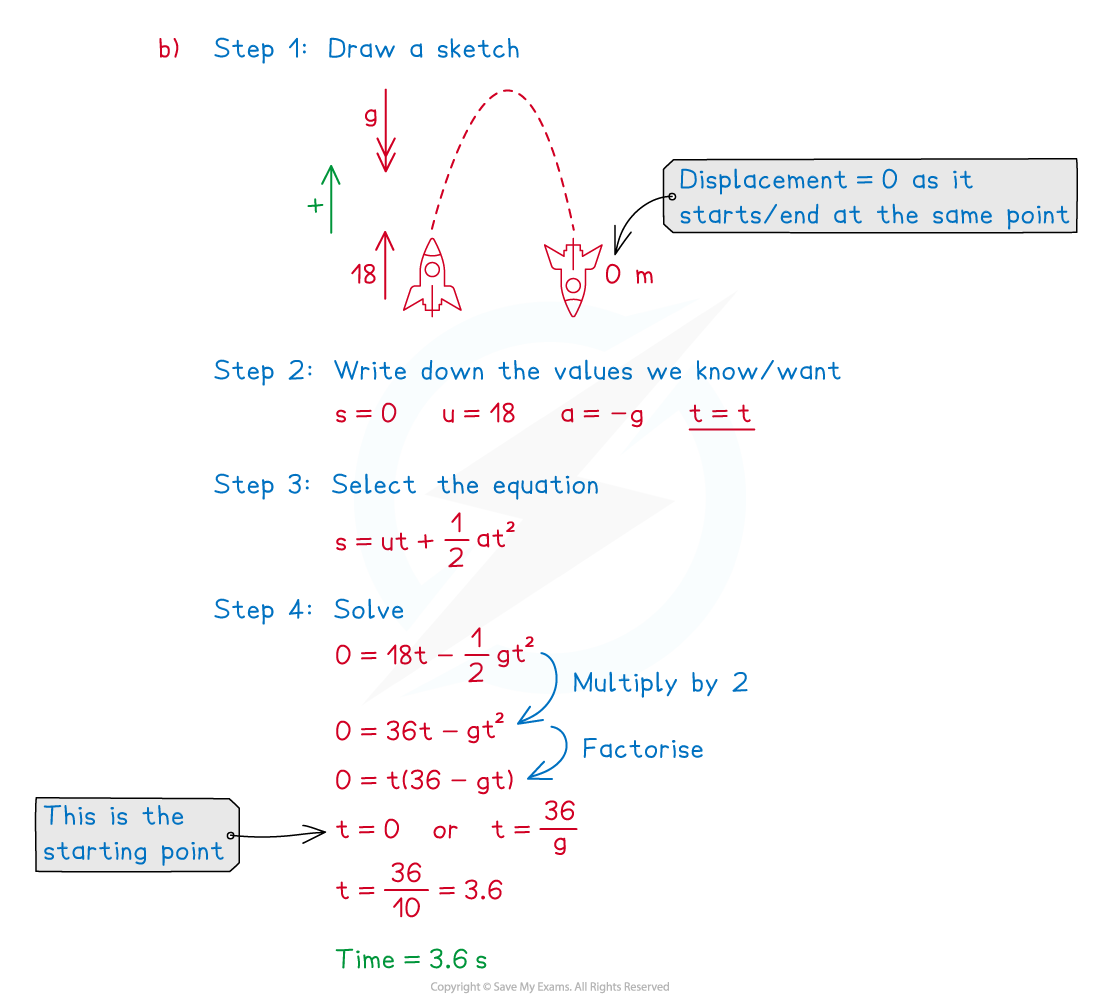
A toy rocket is projected vertically upwards from ground level with initial speed 18 m s-1.
(a)
Find the maximum height the toy rocket reaches.
(b)
Find the time taken from the instant the toy rocket is projected to the instant it returns to the ground.
(a)
Find the maximum height the toy rocket reaches.
(b)
Find the time taken from the instant the toy rocket is projected to the instant it returns to the ground.
Examiner Tip
- If you are asked to find how long it takes before a particle returns to the ground, you can simply use suvat with
- s = 0 if the particle started from the ground
- s = -h if the particle started a height of h m above the ground
- A common mistake is thinking that the speed of the object when it hits the ground is zero. This is incorrect! The object will be travelling with a speed at the instant it hits the ground. It is the impact that causes the speed to go to zero. For an object travelling vertically under gravity, the only time the speed is zero is when it is at its maximum height.

You've read 0 of your 5 free revision notes this week
Sign up now. It’s free!
Did this page help you?
