Revision NotesExam QuestionsPast Papers
A LevelMaths: MechanicsCIERevision Notes2. Kinematics (Straight Line Motion)2.3 Constant Acceleration2.3.1 Deriving the suvat Equations

Deriving the suvat Equations (CIE A Level Maths: Mechanics)

Revision Note

Dan

Author

Dan

Last updated

Did this video help you?

Deriving the suvat Formulae

What is suvat?

  • suvat is an acronym for the five quantities used when modelling motion in a straight-line with constant acceleration
    • s – displacement (from the starting point)
    • u – initial velocity
    • v – final velocity
    • a – acceleration
    • t – time

  • All except time are vector quantities and can be negative
    • time is a scalar quantity

What are the suvat (constant acceleration) equations?

  • The five equations for motion in a straight line are:

v space equals space u space plus space a t v squared space equals space u squared space plus space 2 a s s space equals space 1 half left parenthesis u space plus space v right parenthesis t s space equals space u t space plus 1 half a t squared s space equals space v t space minus 1 half a t squared

  • The equations can only be used when the motion has constant acceleration
  • All equations connect four of the five quantities
    • Knowing any three allows a fourth to be found

  • The first four equations are provided in the exam
    • s equals v t minus 1 half a t squared is not given but it is easy to remember as it is very similar to s equals u t plus 1 half a t squared

How do I derive the suvat equations?

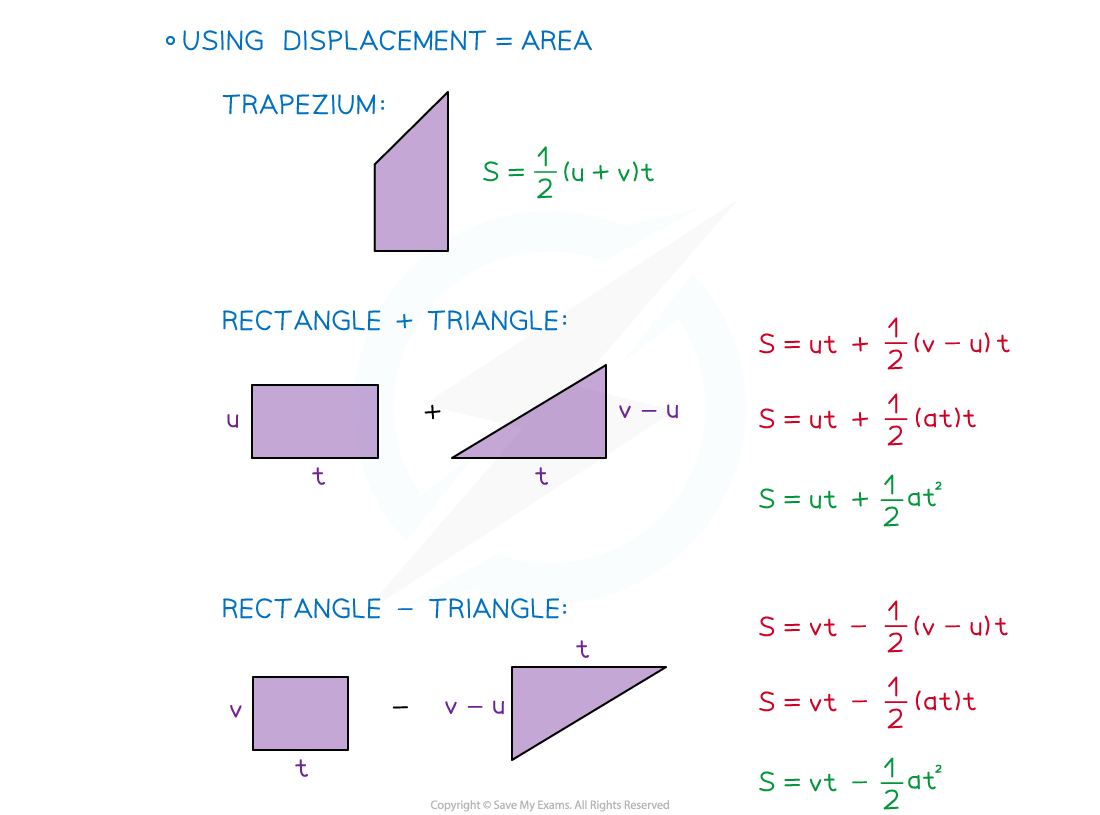
  • The four equations that involve time can be derived from a velocity-time graph
    • The velocity-time graph will be a straight line as the acceleration is constant
    • The fifth equation can be found by choosing any two of the equations and eliminating the t variable (see the worked example

qJL_I9LZ_2-3-1-deriving-the-suvat-formula-diagram-1_2

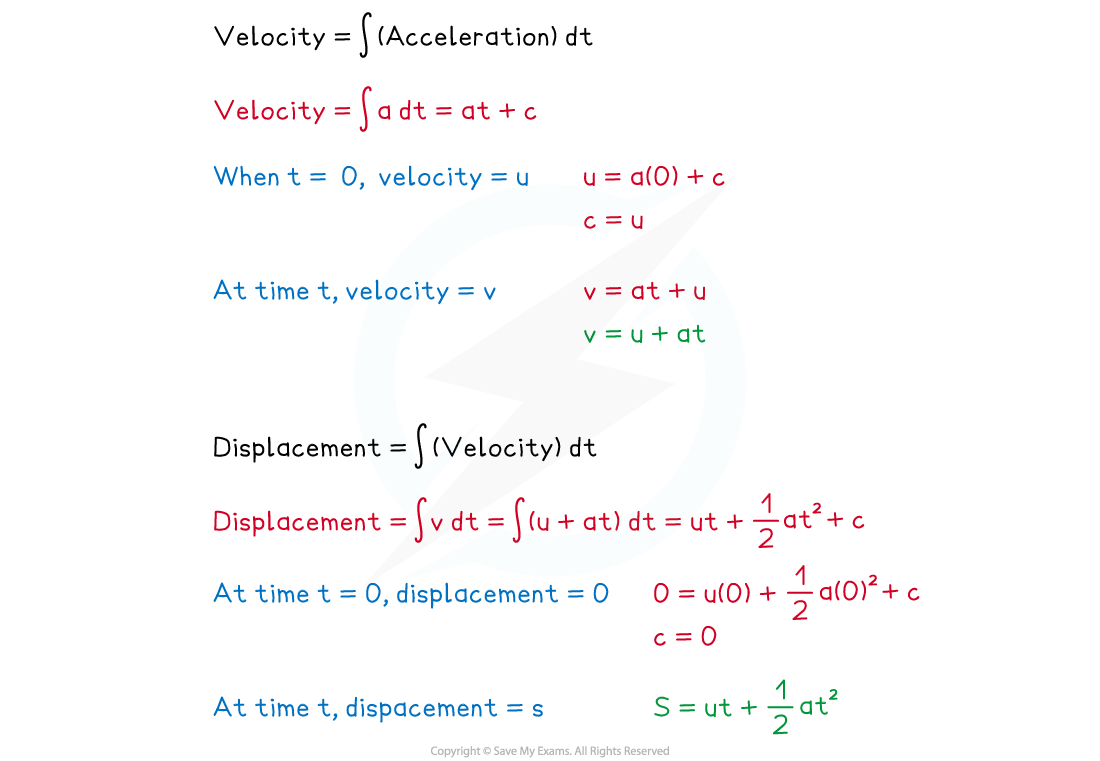
  • Two of the equations can also be derived using calculus
    • Velocity is found by integrating acceleration
    • Displacement is found by integrating velocity

    2-3-1-deriving-the-suvat-formula-diagram-2

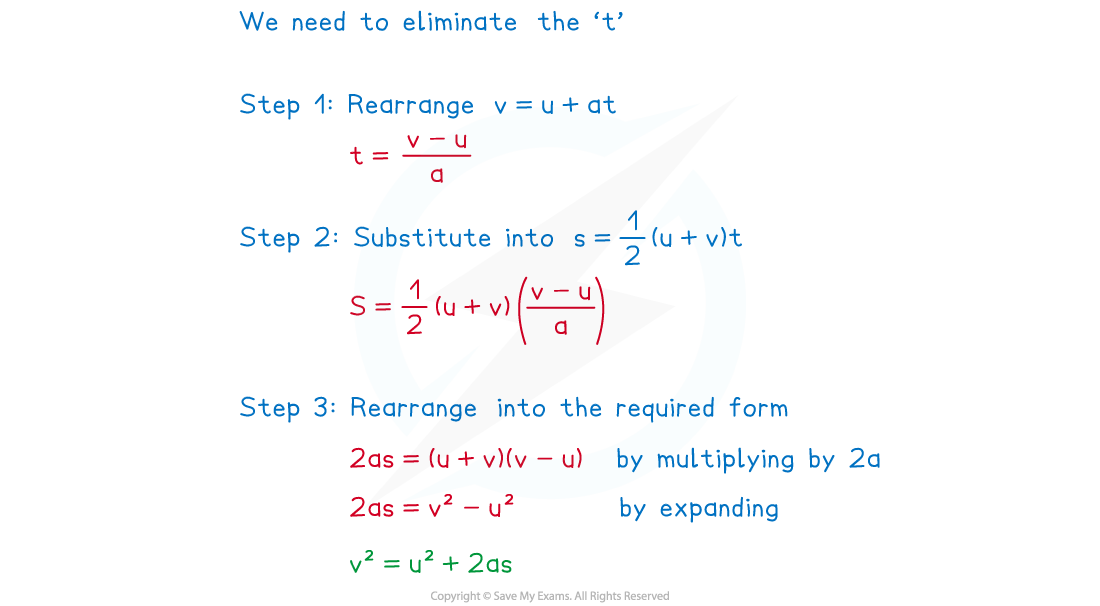
Worked example

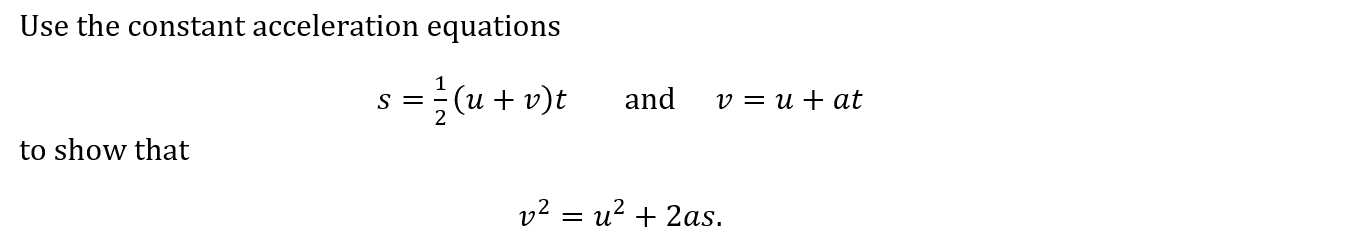
2.3.1_WE_Deriving the suvat formulae_1

Use the constant acceleration equations

s equals 1 half left parenthesis u plus v right parenthesis t and v equals u plus a t

to show that

v squared equals u squared plus 2 a s.

2-3-1-deriving-the-suvat-formula-example-solution

Examiner Tip

  • If you are asked to derive one of the formulae then the question will likely give you a hint as to which method to use. They may provide a velocity-time graph. Make sure you show each step and state any reasons such as the gradient of the graph being the acceleration.
  • If the question does not ask you to derive the formulae, then you can use them freely without proof.

You've read 0 of your 5 free revision notes this week

Sign up now. It’s free!

Join the 100,000+ Students that ❤️ Save My Exams

the (exam) results speak for themselves:

    Test yourself

    Did this page help you?

    Previous:2.2.1 Using Calculus in 1DNext:2.3.2 suvat in 1D
    Dan

    Author: Dan

    Expertise: Maths

    Dan graduated from the University of Oxford with a First class degree in mathematics. As well as teaching maths for over 8 years, Dan has marked a range of exams for Edexcel, tutored students and taught A Level Accounting. Dan has a keen interest in statistics and probability and their real-life applications.