A LevelFurther Maths: Further Statistics 1EdexcelRevision NotesStandard Discrete ModelsPoisson & Binomial DistributionsPoisson Approximations of Binomials

Poisson Approximations of Binomials (Edexcel A Level Further Maths: Further Statistics 1)

Revision Note

Roger

Author

Roger

Last updated

Poisson Approximations of Binomials

When can I use a Poisson distribution to approximate a binomial distribution?

  • A binomial distribution X tilde straight B open parentheses n comma space p close parentheses can be approximated by a Poisson distribution X subscript p tilde Po open parentheses lambda close parentheses provided
    • n is large
    • p is small
    • There is no firm rule for what 'large' and 'small' mean here
      • n greater than 30 is a good guide for 'large n' 
      • usually the value of bold italic n bold italic p should be bold less or equal than bold 10
  • The mean to use in the approximation can be calculated by:
    • bold italic lambda bold equals bold italic n bold italic p
    • This gives the Poisson the same mean as the binomial
    • Recall that for the binomial distribution
      • the mean is n p
      • the variance is n p open parentheses 1 minus p close parentheses
  • If n is large but p is near to 1, consider modelling the number of failures, X apostrophe
    • X apostrophe tilde B open parentheses n comma 1 minus p close parentheses
      • 1 minus p will be small
      • A Poisson approximation can then be used
  • The Poisson distribution is derived from the binomial distribution by letting n become infinitely large and p become infinitely small

Examiner Tip

An exam question will generally state if you need to use a Poisson approximation

Worked example

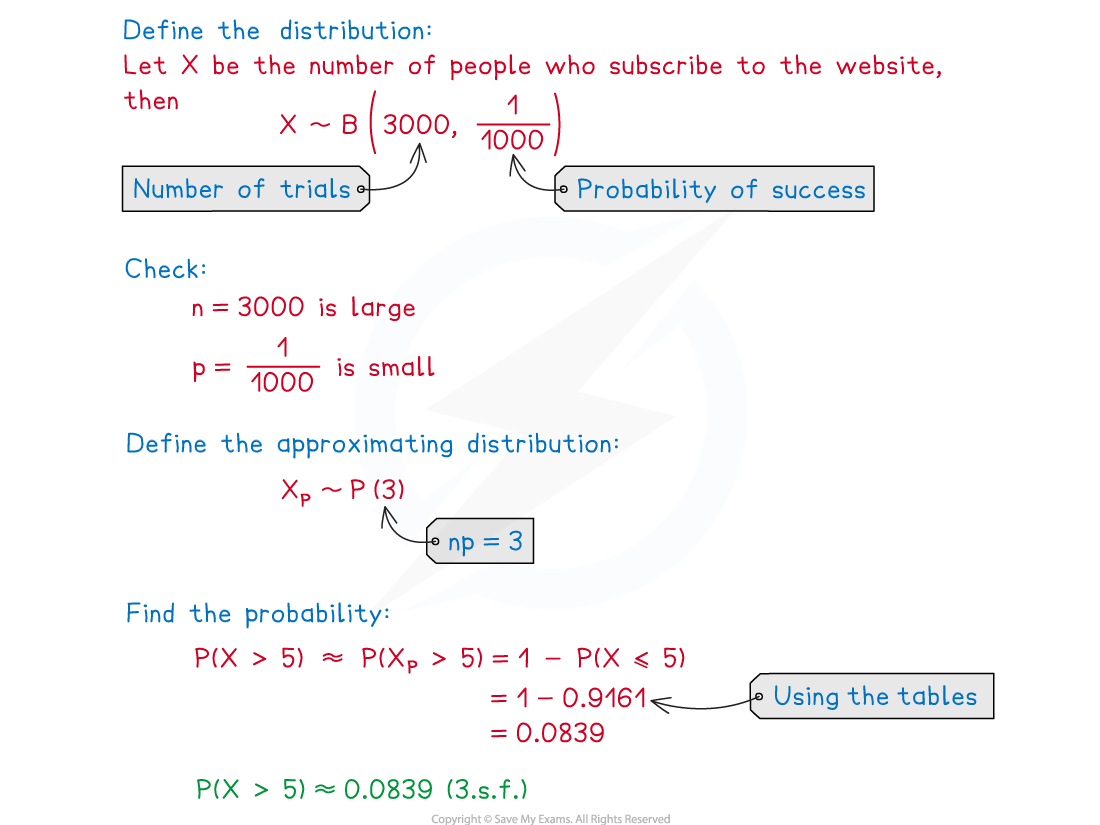
It is known that one person in a thousand who checks a revision website will choose to subscribe. Given that the website received 3000 hits yesterday, use a Poisson approximation to find the probability that more than 5 people subscribed.

1-5-3-poisson-approx-of-binomial-we-solution

You've read 0 of your 5 free revision notes this week

Sign up now. It’s free!

Join the 100,000+ Students that ❤️ Save My Exams

the (exam) results speak for themselves:

    Did this page help you?

    Previous:The Poisson DistributionNext:The Geometric Distribution
    Roger

    Author: Roger

    Expertise: Maths

    Roger's teaching experience stretches all the way back to 1992, and in that time he has taught students at all levels between Year 7 and university undergraduate. Having conducted and published postgraduate research into the mathematical theory behind quantum computing, he is more than confident in dealing with mathematics at any level the exam boards might throw at you.