The Negative Binomial Distribution | Edexcel A Level Further Maths: Further Statistics 1 Revision Notes 2017

Conditions for Negative Binomial Models

What is the negative binomial distribution?

The negative binomial distribution models the number of trials needed to reach a fixed number of successes, $r$
- For example, how many times will you have to roll a dice until it lands on a '6' for the third time
There is no one standard form of notation for the negative binomial distribution
- But for a random variable $X$ that has the negative binomial distribution you could write either:
  - $X ~ NB (r, p)$ or $X ~ Negative B (r, p)$
- $X$ is the number of trials that will be required to reach a total of $r$ successes
- $p$ is the fixed probability of success in any one trial

What are the conditions for using a negative binomial model?

A negative binomial model can be used for an experiment that satisfies the following conditions:
- The experiment consists of an indefinite number of successive trials
- The outcome of each trial is independent of the outcomes of all other trials
- There are exactly two possible outcomes for each trial (success and failure)
- The probability of success in any one trial ( $p$ ) is constant
Note that these conditions are very similar to the conditions for the binomial distribution
- But for a binomial distribution the number of trials ( $n$ ) is fixed
  - And you count the number of successes
- While for a negative binomial distribution the number of successes ( $r$ ) is fixed
  - And you count the number of trials it takes to reach that number of successes

When might the conditions not be satisfied?

If asked to criticise a negative binomial model, you may be able to question whether the trials are really independent
- For example, someone may be repeating an activity until they achieve the $r$ th success
  - The trials may not be independent because the person gets better from practising the activity
  - This also means the probability of success, $p$ , is not constant
- In order to proceed using the model, you will have to assume trials are independent

Examiner Tip

Replace the word "trials" with the context (e.g. "flips of a coin") when commenting on conditions and assumptions

Negative Binomial Probabilities

What are the probabilities for the negative binomial distribution?

If $X ~ Negative B (r, p)$ , then $X$ has the probability function:
- $P (X = x) = p (x) = (\begin{matrix} x - 1 \\ r - 1 \end{matrix}) p^{r} {(1 - p)}^{x - r}, x = r, r + 1, r + 2, . . .$
- the random variable $X$ is the number of trials needed to get $r$ successes
- $p$ is the constant probability of success in one trial
- $P (X = x)$ is the probability that the $r th$ success will occur on the $x th$ trial

Note that that is the product of
- the binomial probability of getting $r - 1$ successes in $x - 1$ trials, $(\begin{matrix} x - 1 \\ r - 1 \end{matrix}) p^{r - 1} {(1 - p)}^{x - r}$ ,
- and the probability of getting a success in the $x th$ trial, $p$
$(\begin{matrix} x - 1 \\ r - 1 \end{matrix})$ is the binomial coefficient
- i.e., $(\begin{matrix} x - 1 \\ r - 1 \end{matrix}) = {}^{(x - 1)}C_{(r - 1)}$
Also note that there is no greatest possible value of $x$
- It could require any number of trials to reach the $r th$ success
- However for any given $r$ , $P (X = x)$ gets closer and closer to zero as $x$ gets larger

Where does the formula come from?

Consider rolling a fair dice and wanting to know the probability that it would take 12 rolls for the dice to land on '6' a total of 3 times
- This is the same as saying that the third '6' occurs on the 12th roll
You can model this situation using the random variable $X ~ Negative B (3, \frac{1}{6})$
- 'success' here is defined as 'roll a 6'
- $r = 3$ because we're interested in the number of trials required to reach a total of 3 successes
- $p = \frac{1}{6}$ is the probability of rolling a '6' on a fair dice
- $X$ is the number of trials that will be required to reach 3 successes
The probability you are looking for is therefore $P (X = 12)$
For the third '6' to occur on the 12th roll, the following things need to happen:
- '6' must occur exactly 2 times in the first 11 rolls
  - It doesn't matter which rolls those two '6's occur on
  - The probability of that happening is $P (Y = 2)$ , for $Y ~ B (11, \frac{1}{6})$
  - So that part of the answer is a binomial probability
- Then a '6' must also occur on the 12th roll
  - The probability of that happening is $\frac{1}{6}$
- So the probability of both those things happening is

$P (Y = 2) \times \frac{1}{6} = ((\begin{matrix} 11 \\ 2 \end{matrix}) {(\frac{1}{6})}^{2} {(\frac{5}{6})}^{9}) \times \frac{1}{6} = (\begin{matrix} 11 \\ 2 \end{matrix}) {(\frac{1}{6})}^{3} {(\frac{5}{6})}^{9} = 0.0493489 . . .$

That same logic can be used to find the general formula for negative binomial probabilities

Is there a connection between negative binomial probabilities and geometric probabilities?

Note that when $r = 1$ ,the negative binomial probability function becomes:

$P (X = x) = p (x) = (\begin{matrix} x - 1 \\ 0 \end{matrix}) p^{1} {(1 - p)}^{x - 1} = p {(1 - p)}^{x - 1}, x = 1, 2, 3, . . .$

- That is the same as the geometric distribution probability function

The geometric distribution is the 'special case' of the negative binomial distribution when $r = 1$

Examiner Tip

Make sure you are clear about what $x$ , $r$ , $p$ and $P (X = x)$ refer to in the formula!
Read the question carefully to determine whether binomial, geometric or negative binomial probabilities are required

Worked example

Emanuel is playing in a chess tournament, where his probability of winning any one game is 0.55. Find the probability that:

his first win is in the third game he plays

negb-probs-we-a

he wins exactly 4 of his first 7 games

negb-probs-we-b

he wins for the fourth time in his seventh game

negb-probs-we-c

he wins for the fourth time in his seventh game, given that he won his first game

negb-probs-we-d

his fourth win occurs in or before his seventh game.

negb-probs-we-e

Criticise the model used in this question.

negb-probs-we-f

Negative Binomial Mean & Variance

What are the mean and variance of the negative binomial distribution?

If $X ~ Negative B (r, p)$ , then
- The mean of $X$ is $E (X) = μ = \frac{r}{p}$

- The variance of $X$ is $Var (X) = σ^{2} = \frac{r (1 - p)}{p^{2}}$
You need to be able to use these formulae to answer questions about the negative binomial distribution.

Examiner Tip

If a question gives you the mean and/or variance with one known parameters ( $r$ or $p$ ), form an equation to find the other

Worked example

Croesus is tossing a biased coin for which the probability of the coin landing on 'heads' is $p$ . The random variable $X$ represents the number of times he needs to flip the coin until it has landed on heads four times. Given that the mean of $X$ is 10, find:

the value of

p

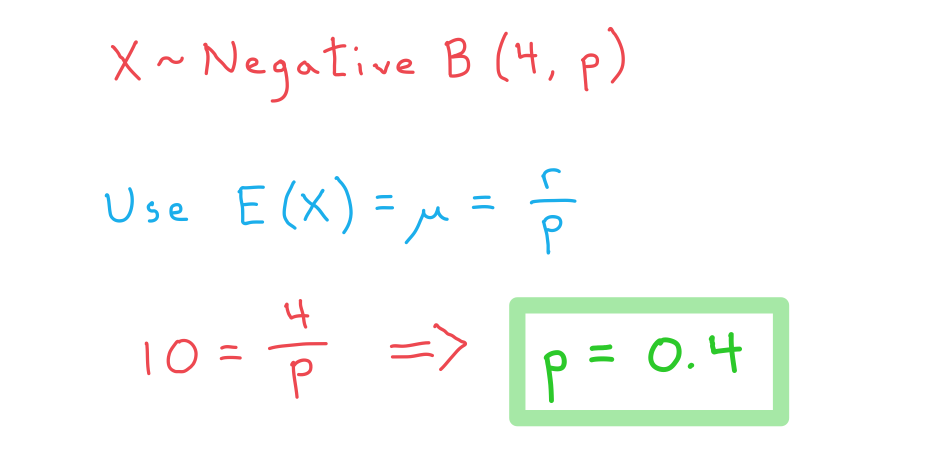
negb-mean-var-we-a

the standard deviation of

X

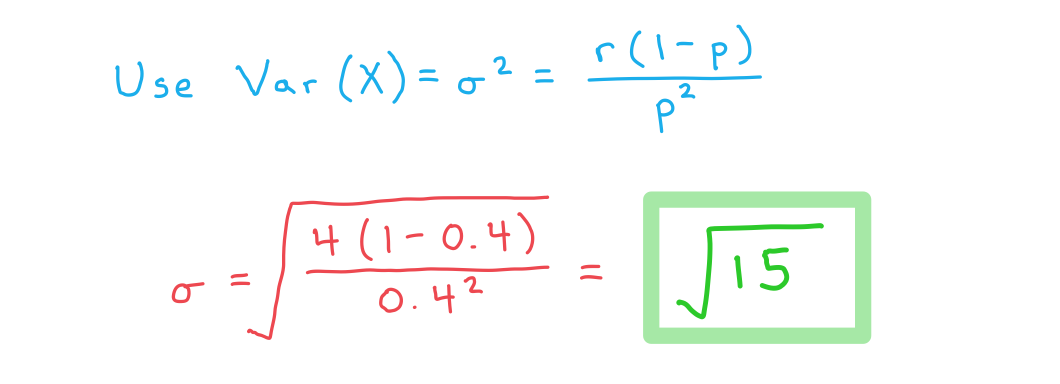
negb-mean-var-we-b

the probability that the fourth 'head' will occur on the seventh toss.

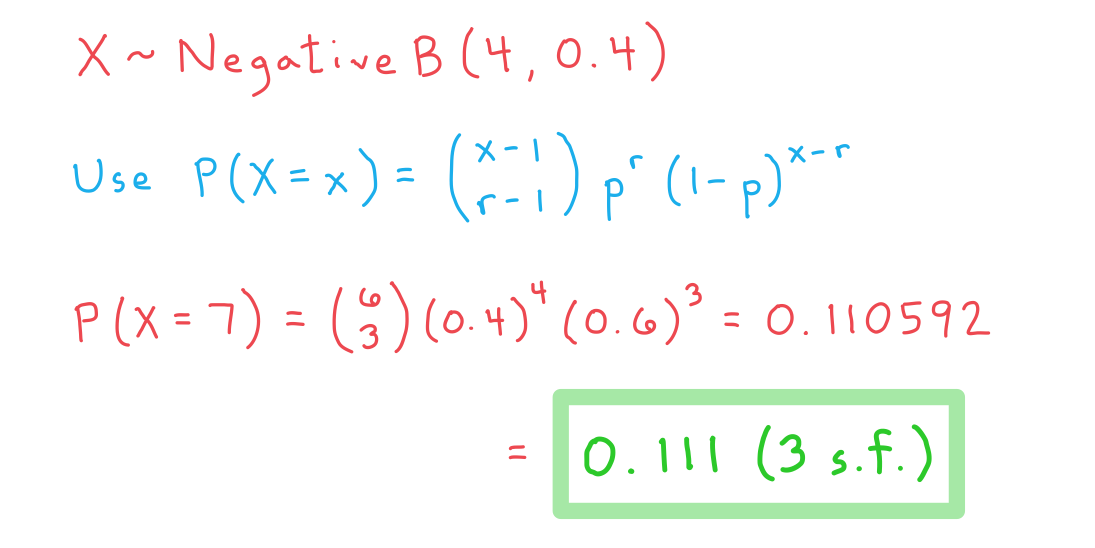
negb-mean-var-we-c

The Negative Binomial Distribution (Edexcel A Level Further Maths: Further Statistics 1)

Revision Note

Conditions for Negative Binomial Models

What is the negative binomial distribution?

What are the conditions for using a negative binomial model?

When might the conditions not be satisfied?

Examiner Tip

Negative Binomial Probabilities

What are the probabilities for the negative binomial distribution?

Where does the formula come from?

Is there a connection between negative binomial probabilities and geometric probabilities?

Examiner Tip

Worked example

Negative Binomial Mean & Variance

What are the mean and variance of the negative binomial distribution?

Examiner Tip

Worked example

You've read 0 of your 5 free revision notes this week

Sign up now. It’s free!

Join the 100,000+ Students that ❤️ Save My Exams

Author: Roger