Poisson Hypothesis Testing

How do I test for the mean of a Poisson distribution?

If $X ~ Po (λ)$ , test for the mean, $λ$ , using the following hypotheses
- $H_{0} : λ = . . .$
- $H_{1} : λ \neq . . .$ or $λ < . . .$ or $λ > . . .$
- with significance level $α$
  - For example, $α = 0.05$ for 5%
You will be given an observed value, $x$ , in the question
- This is what is being tested against $λ$
  - For example, "There's usually 3 accidents per hour ( $λ = 3$ ), but last week there was 5 accidents per hour ( $x = 5$ )"
- You may need to rescale $λ$ to fit in the same interval of time or space as $x$
Assuming $H_{0} : λ = . . .$
- Find the probability that $X$ is the observed value $x$ , or more extreme than that
- If the total probability of these values is $< α$ (or $< \frac{α}{2}$ for two-tailed tests)
  - Write that "there is sufficient evidence to reject $H_{0}$ "
- If not, write that "there is insufficient evidence to reject $H_{0}$ "
Write a conclusion in context
- For example
  - "the mean number of accidents has increased from 3 per hour"
  - or "the mean number of accidents has not changed from 3 per hour"

How do I find the critical region for a Poisson hypothesis test?

If $H_{1} : λ < . . .$
- Assume that $H_{0} : λ = . . .$
- Then test different integer values, $c$ , to get $P (X \leq c)$ as close to $α$ as possible, without exceeding it
  - Use cumulative Poisson tables or a calculator to help
  - The integer that's the nearest is called the critical value
  - Checking one integer higher should show that $P (X \leq c + 1)$ is $> α$
- The critical region is $X \leq c$
If $H_{1} : λ > . . .$
- It's the same process, but with $P (X \geq c)$ as close to $α$ as possible, without exceeding it
  - Beware of integers with discrete inequalities
    - $P (X \geq c)$ means $1 - P (X \leq c - 1)$
    - You may have found what $c - 1$ is, not what $c$ is!
- The critical region is $X \geq c$
If $H_{1} : λ \neq . . .$
- The critical region is $X \leq c_{1}$ or $X \geq c_{2}$
  - $P (X \leq c_{1})$ is as close to $\frac{α}{2}$ as possible, without exceeding it
  - $P (X \geq c_{2})$ is as close to $\frac{α}{2}$ as possible, without exceeding it

What is the actual significance level?

As the Poisson model is discrete, it's not possible to get a critical region whose probability sums to $α$ exactly
- That's because $X$ can only take integer values
Whatever it does sum to is called the actual significance level
- The actual amount of probability in the tail (or tails)
For example, if $H_{1} : λ < . . .$ has the critical region $X \leq c$
- Then $P (X \leq c)$ will be just less than $α$
  - It's value is the actual significance level
  - It represents the probability of rejecting $H_{0}$ incorrectly (when $H_{0}$ was actually true)
Some questions want a critical region that's as close to $α$ as possible, even if that means probabilities that exceed $α$
- For example, if $P (X \leq 6) = 0.0298$ and $P (X \leq 7) = 0.0510$ where $α = 0.05$
  - Then $X \leq 7$ is the critical region that's as close to $α$ as possible
  - The actual significance level is 0.0510

Examiner Tip

For finding critical regions, sometimes cumulative Poisson tables can be easier to read than calculators

Worked example

Mr Viajo believes that his travel blog receives an average of 8 likes per day (24 hour period). He tries a new advertising campaign and carries out a hypothesis test at the 5% level of significance to see if there is a change in the number of likes he gets. Over a 12-hour period chosen at random Mr Viajo’s travel blog receives 7 likes.

(i)

State null and alternative hypotheses for Mr Viajo’s test.

(ii)

Find the critical regions for the test.

(iii)

Find the actual level of significance.

(iv)

Carry out the hypothesis test, writing your conclusion clearly.

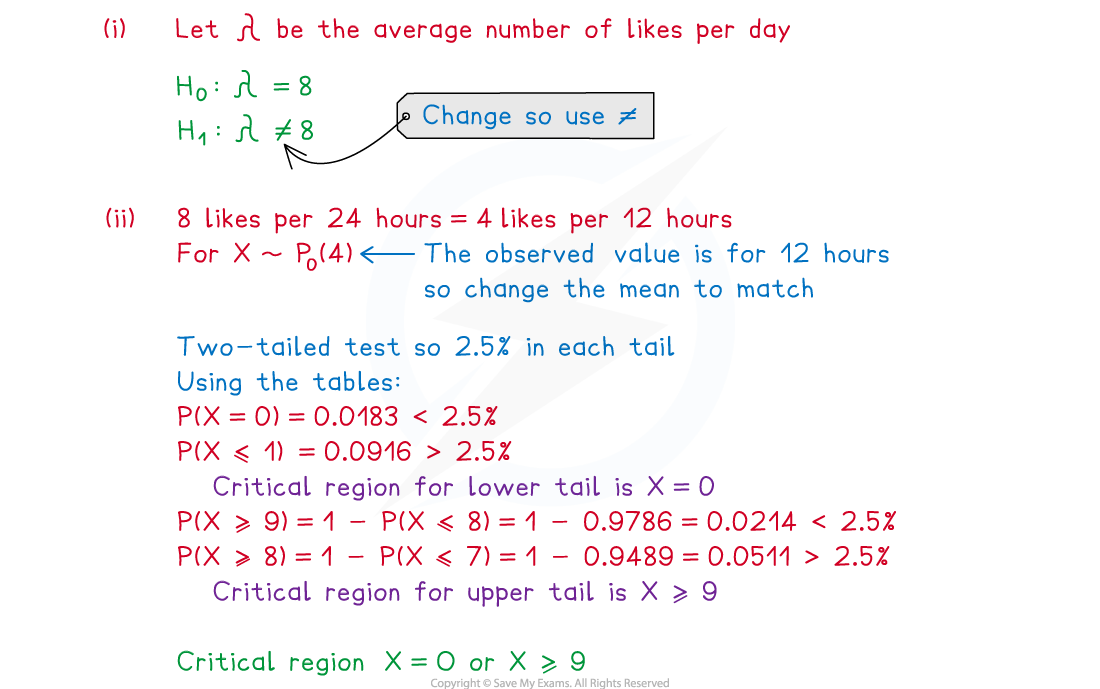
2-2-2-poisson-hyp-testing-we-solution-part-1

Poisson Hypothesis Testing (Edexcel A Level Further Maths: Further Statistics 1)

Revision Note