Angle between Lines (Edexcel A Level Further Maths) : Revision Note
Scalar Product
The scalar product is an important link between the algebra of vectors and the trigonometry of vectors. We shall see that the scalar product is somewhat comparable to the operation of multiplication on real numbers.
What is the scalar (dot) product?
The scalar product between two vectors a and b is represented by
This is also called the dot product because of the symbol used
The scalar product between two vectors
and
is defined as
The result of taking the scalar product of two vectors is a real number
i.e. a scalar
For example,
and
The scalar product has some important properties:
The order of the vectors doesn’t affect the result:
In effect we can ‘multiply out’ brackets:
This means that we can do many of the same things with vectors as we can do when operating on real numbers – for example,
The scalar product between a vector and itself is equal to the square of its magnitude:
For example,
and
What is the connection between the scalar product and trigonometry?
There is another important method for finding
involving the angle between the two vectors
:
Here
is the angle between the vectors when they are placed ‘base to base’
when the vectors are placed so that they begin at the same point
This formula can be derived using the cosine rule and expanding
The scalar product of two vectors gives information about the angle between the two vectors
If the scalar product is positive then the angle between the two vectors is acute (less than 90°)
If the scalar product is negative then the angle between the two vectors is obtuse (between 90° and 180°)
If the scalar product is zero then the angle between the two vectors is 90° (the two vectors are perpendicular)

How do I tell if vectors or lines are perpendicular?
Two (non-zero) vectors
and
are perpendicular if, and only if,
If the a and b are perpendicular then:
If
then:
a and b are perpendicular
For example, the vectors
and
are perpendicular since
Examiner Tips and Tricks
When writing a scalar product, it’s important to write a distinctive dot between the vectors – otherwise your meaning will not be clear.
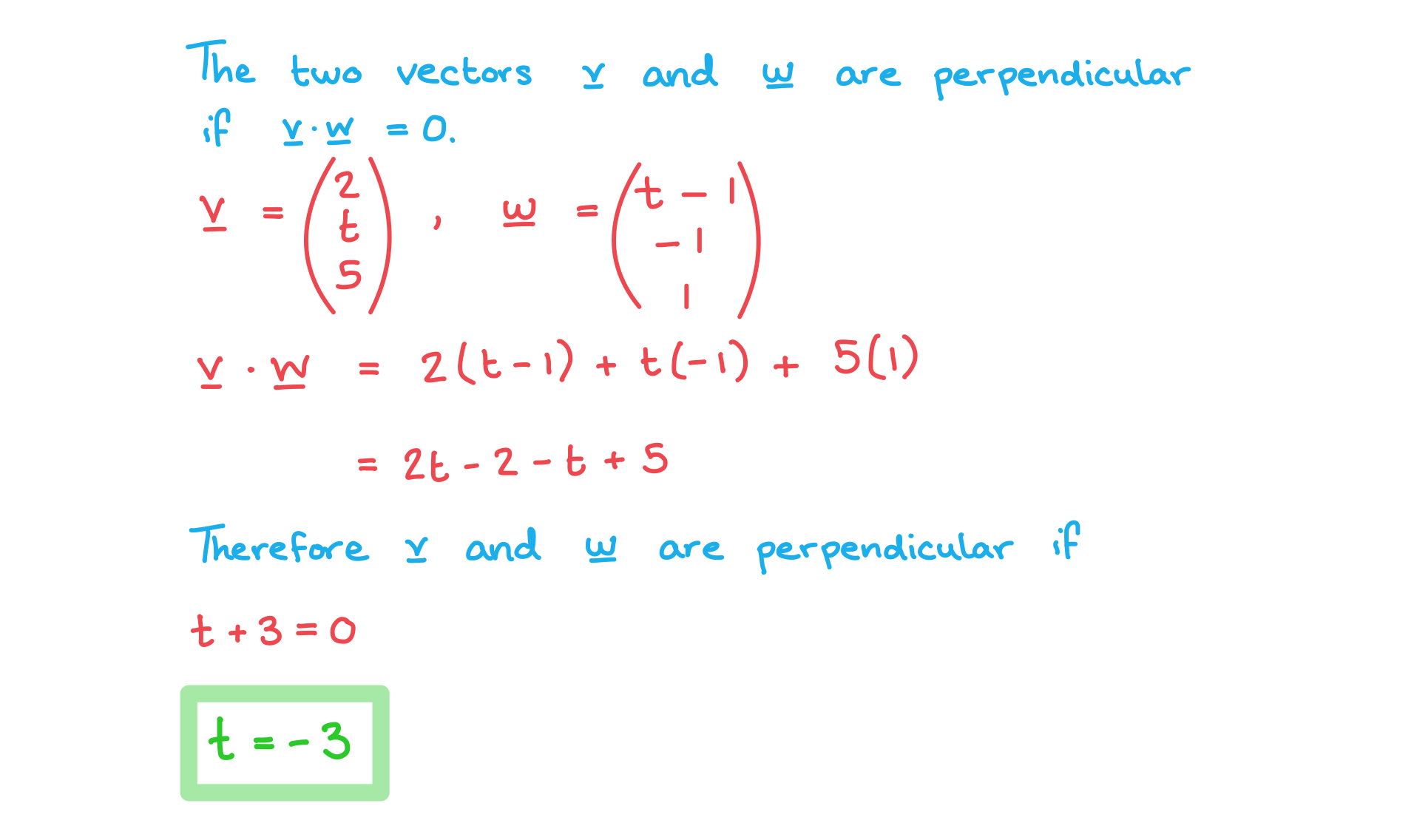
Worked Example
Find the value of t such that the two vectors and
are perpendicular to each other.
Angle between Lines
How do I find the angle between two vectors?
Recall that a formula for the scalar (or ‘dot’) between vectors
and
is
where
is the angle between the vectors when they are placed ‘base to base’
that is, when the vectors are positioned so that they start at the same point
We arrange this formula to make
the subject:
To find the angle between two vectors
Calculate the scalar product between them
Calculate the magnitude of each vector
Use the formula to find
Use inverse trig to find
How do I find the angle between two lines?
To find the angle between two lines, find the angle between their direction vectors
For example, if the lines have equations
and
, then the angle
between the lines is given by
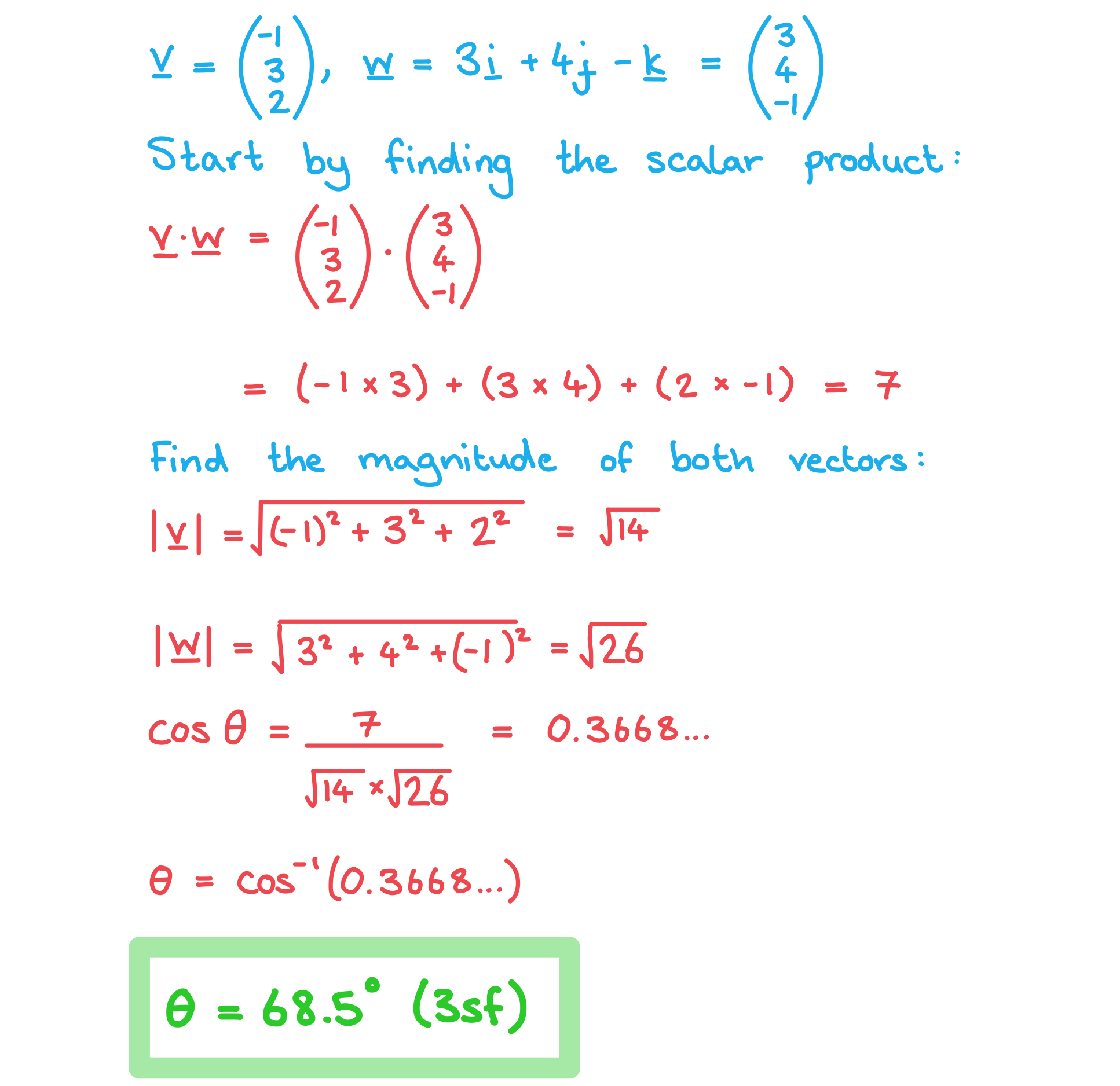
Worked Example
Calculate the angle formed by the two vectors and
.

You've read 0 of your 5 free revision notes this week
Unlock more, it's free!
Did this page help you?
