Revision NotesExam QuestionsPast Papers
A LevelFurther MathsEdexcelCore PureRevision NotesDifferential EquationsSimple Harmonic MotionSimple Harmonic Motion

Simple Harmonic Motion (Edexcel A Level Further Maths): Revision Note

Roger B

Author

Roger B

Last updated

Simple Harmonic Motion (SHM) Equations

What is simple harmonic motion?

  • A particle undergoing simple harmonic motion moves along a straight line subject to the following constraints:

    • The acceleration of the particle is always directed towards a fixed point on the line of motion

    • The acceleration is proportional to the displacement of the particle from the fixed point

  • As a result the particle oscillates back and forth along the line around the fixed point

  • Many physical systems can be modelled using simple harmonic motion

    • One example is an object attached to a spring oscillating in one dimension, when friction, air resistance and other such resistive forces are disregarded

What is the equation that describes simple harmonic motion?

  • The standard form of the simple harmonic motion equation is x with ¨ on top equals negative omega squared x

    • x is the displacement of the particle from the fixed point

      • The fixed point is normally indicated by O and is the origin (i.e. zero point) of the coordinate system

      • The fixed point O is known as the centre of oscillation

    • omega squared is the constant of proportionality, and represents the strength of the force accelerating the particle back towards point O

      • The negative sign means that the acceleration is always directed back towards O

      • We use omega squared to assure that the constant is positive, and also to simplify the notation for the solution to the equation

    • x with ¨ on top equals fraction numerator d squared x over denominator d t squared end fraction is the acceleration of the particle

      • With simple harmonic motion, Newton’s ‘dot notation’ is often used for the derivatives

      • In this notation, x with ¨ on top equals fraction numerator d squared x over denominator d t squared end fraction and x with. on top equals fraction numerator d x over denominator d t end fraction

Solutions to SHM Equations

What is the solution to the standard simple harmonic motion equation?

  • The SHM equation may be solved using the standard techniques for second order differential equations

    • x with ¨ on top equals negative omega squared x may be rearranged to give the homogeneous second order equation x with ¨ on top plus omega squared x equals 0

    • That has auxiliary equation  m squared plus omega squared equals 0 with roots m equals plus-or-minus omega straight i

    • Therefore the general solution is x equals A cos omega t plus B sin omega t

      • Initial or boundary conditions given with a question may allow you to find the precise values of the arbitrary constants A and B

  • The linear combination of sine and cosine terms in the general solution may be rewritten in the form x equals R sin open parentheses omega t plus alpha close parentheses

    • This can sometimes be a more useful form for the general solution

    • This form of the equation shows that the particle oscillates around the fixed point O (i.e., around the centre of oscillation)

    • The amplitude of the motion is R

      • R equals square root of A squared plus B squared end root

      • This is the maximum distance that the particle moves away from the fixed point O

    • The period of the motion is fraction numerator 2 straight pi over denominator omega end fraction

      • This is the length of time it takes the particle to complete one oscillation

    • The constant α is a ‘phase constant’

      • alpha equals tan to the power of negative 1 end exponent open parentheses A over B close parentheses 

      • If α = 0, then the particle is at point O (i.e., x = 0) when t = 0

      • If alpha equals straight pi over 2 then the particle is at its maximum displacement (i.e., x = R) when t = 0

  • Because acceleration is the derivative of velocity, and velocity is the derivative of displacement, we may use the chain rule to write

x with ¨ on top equals fraction numerator d v over denominator d t end fraction equals fraction numerator d x over denominator d t end fraction cross times fraction numerator d v over denominator d x end fraction equals v fraction numerator d v over denominator d x end fraction

  • Substituting that into the standard SHM equation gives

v fraction numerator d v over denominator d x end fraction equals negative omega squared x

  • That form of the SHM equation may be solved using separation of variables:

    integral v d v equals integral negative omega squared x d x rightwards double arrow 1 half v squared equals negative 1 half omega squared x squared plus c

    • But the velocity is momentarily zero when the particle reaches its maximum displacement

      • After this the particle’s velocity changes direction and the particle heads back towards the centre of oscillation

    • This gives us a boundary condition (because we know that v = 0 when x = R) and allows us to find the value of the constant of integration:

      1 half left parenthesis 0 right parenthesis squared equals negative 1 half omega squared left parenthesis R right parenthesis squared plus c rightwards double arrow c equals 1 half omega squared R squared

    • Substituting that value of c into the solution and rearranging gives

      v squared equals omega squared open parentheses R squared minus x squared close parentheses

      • where R again is the amplitude of the simple harmonic motion

  • Note that this version of the solution connects the velocity v to the displacement x, and is independent of the time t

    • Taking square roots gives v equals plus-or-minus omega square root of R squared minus x squared end root

    • Be careful when using this to answer questions

      • The direction of the velocity (plus or minus) will depend on the displacement (plus or minus) and whether the particle is moving towards or away from the centre of oscillation

Examiner Tips and Tricks

  • Even though you may have memorised the forms of the solutions for the SHM equation, it is important on an exam question to derive the solution ‘from scratch’, showing your method and working

Worked Example

A particle is moving along a straight line.  At time t seconds its displacement x metres from a fixed point O is such that fraction numerator d squared x over denominator d t squared end fraction equals negative 9 x.  At time t = 0, x = 2 and the velocity of the particle is 9 ms-1.

a) Find an expression for the displacement of the particle at time t seconds.

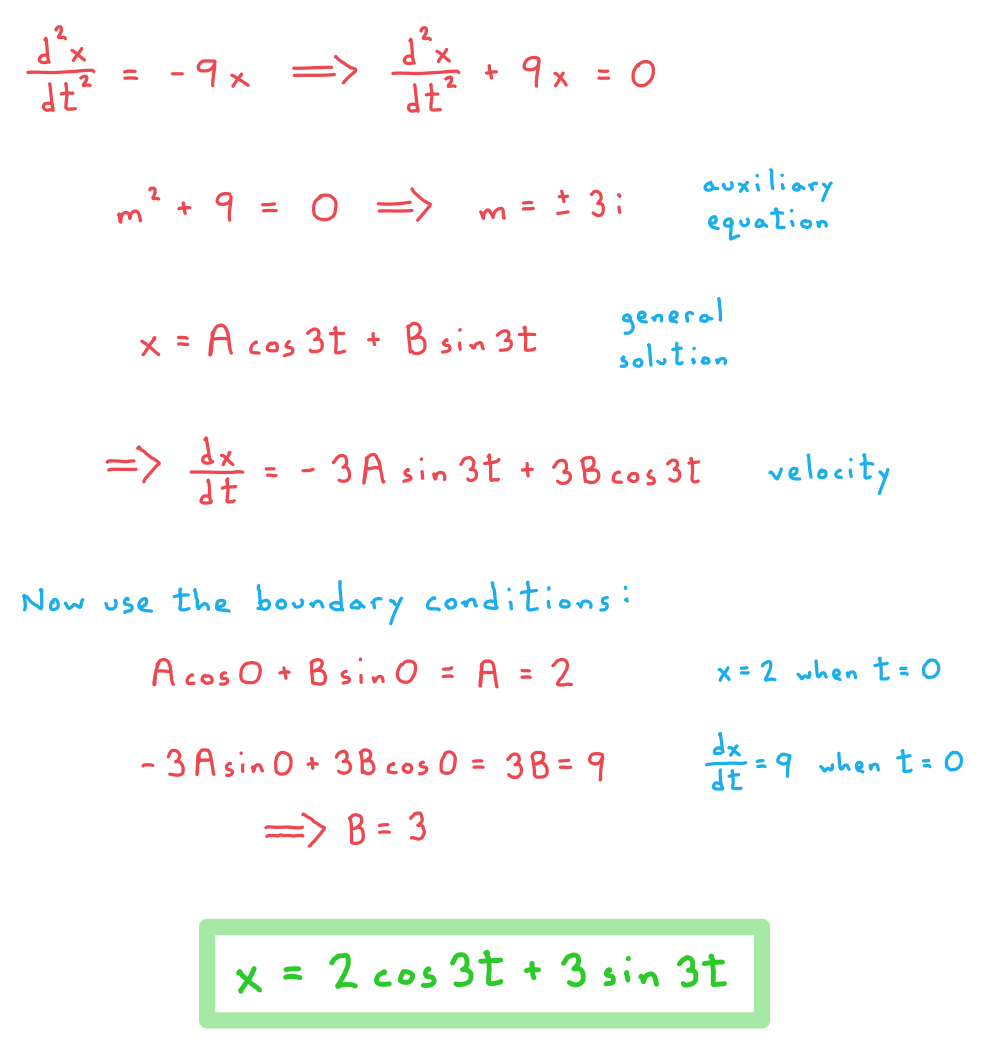
8-3-1-solutions-to-shm-eqns-a-we-solution

b) Hence determine the maximum displacement of the particle from O.

8-3-1-solutions-to-shm-eqns-b-we-solution

c) Show that the relationship between the velocity v and displacement x of the particle may be described by the equation v squared equals 117 minus 9 x squared.

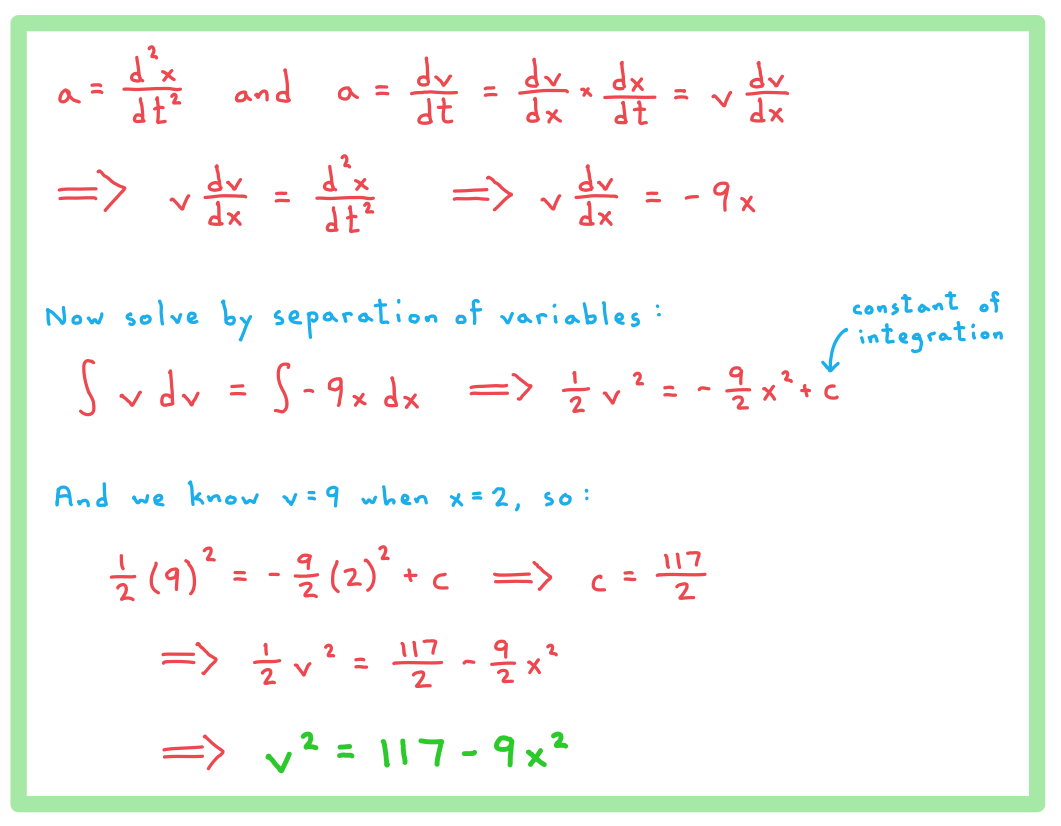
8-3-1-solutions-to-shm-eqns-c-we-solution

You've read 0 of your 5 free revision notes this week

Sign up now. It’s free!

Join the 100,000+ Students that ❤️ Save My Exams

the (exam) results speak for themselves:

    Test yourself

    Did this page help you?

    Previous:Coupled First Order Linear EquationsNext:Damped or Forced Harmonic Motion
    Roger B

    Author: Roger B

    Expertise: Maths

    Roger's teaching experience stretches all the way back to 1992, and in that time he has taught students at all levels between Year 7 and university undergraduate. Having conducted and published postgraduate research into the mathematical theory behind quantum computing, he is more than confident in dealing with mathematics at any level the exam boards might throw at you.