Calculus involving Inverse Trig | Edexcel A Level Further Maths: Core Pure Revision Notes 2017

Differentiating Inverse Trig Functions

What are the inverse trigonometric functions?

arcsin, arccos and arctan are functions defined as the inverse functions of sine, cosine and tangent respectively

$\arcsin (\frac{\sqrt{3}}{2}) = \frac{π}{3}$ which is equivalent to $\sin (\frac{π}{3}) = \frac{\sqrt{3}}{2}$
$\arctan (- 1) = \frac{3 π}{4}$ which is equivalent to $\tan (\frac{3 π}{4}) = - 1$

What are the derivatives of the inverse trigonometric functions?

$f (x) = \arcsin x$
- $f' (x) = \frac{1}{\sqrt{1 - x^{2}}}$
$f (x) = \arccos x$
- $f' (x) = - \frac{1}{\sqrt{1 - x^{2}}}$
$f (x) = \arctan x$
- $f' (x) = \frac{1}{1 + x^{2}}$
Unlike other derivatives these look completely unrelated at first
- their derivation involves use of the identity $\cos^{2} x + \sin^{2} x \equiv 1$
- hence the squares and square roots!
All three are given in the formula booklet
Note with the derivative of $\arctan x$ that $(1 + x^{2})$ is the same as $(x^{2} + 1)$

How do I show or prove the derivatives of the inverse trigonometric functions?

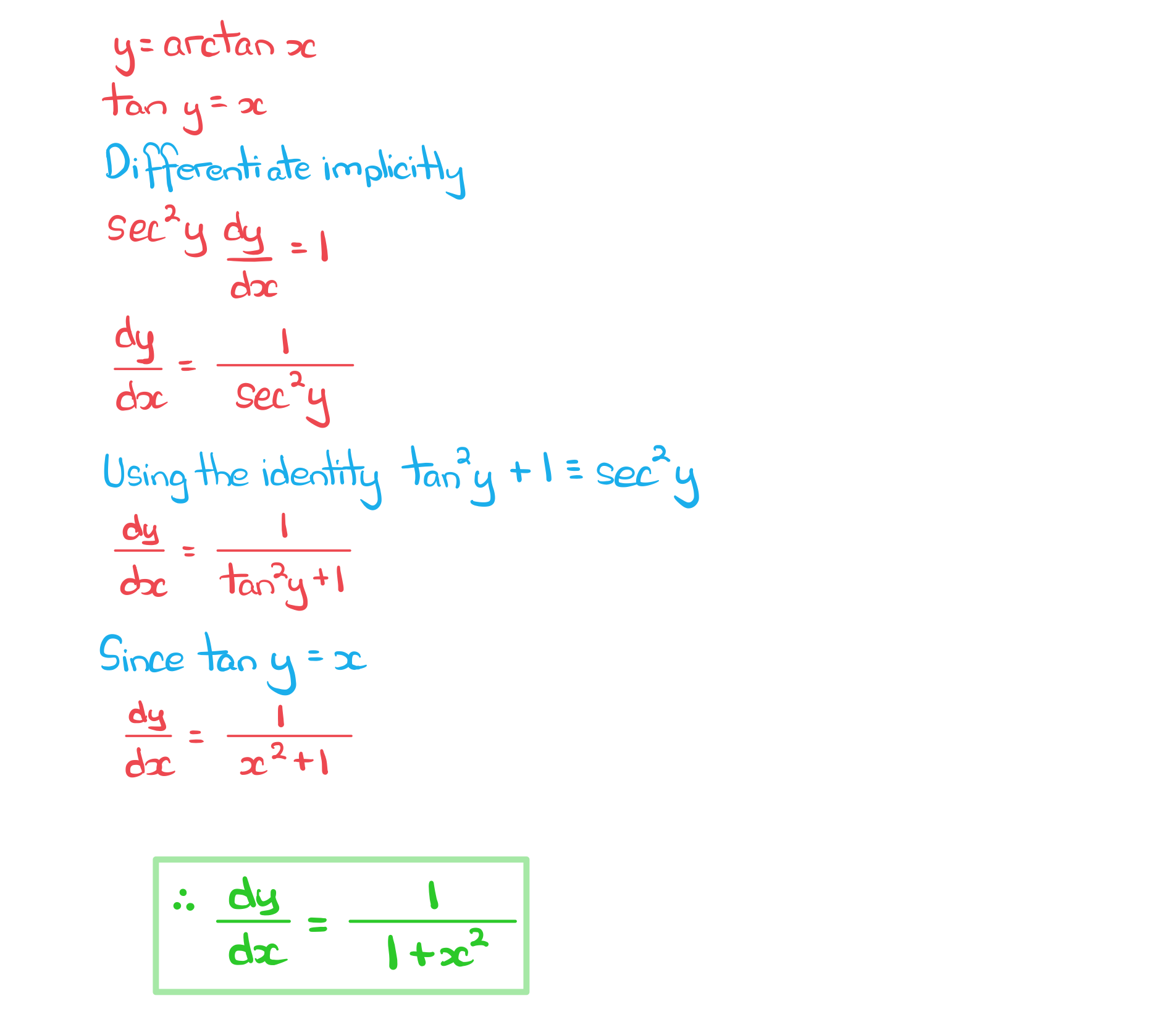
For $y = \arcsin x$
- Rewrite, $\sin y = x$
- Differentiate implicitly, $\cos y \frac{d y}{d x} = 1$
- Rearrange, $\frac{d y}{d x} = \frac{1}{\cos y}$
- Using the identity $\cos^{2} y \equiv 1 - \sin^{2} y$ rewrite, $\frac{d y}{d x} = \frac{1}{\sqrt{1 - \sin^{2} y}}$
- Since, $\sin y = x$ , $\frac{d y}{d x} = \frac{1}{\sqrt{1 - x^{2}}}$
Similarly, for $y = \arccos x$
- $\cos y = x$
- $- \sin y \frac{d y}{d x} = 1$
- $\frac{d y}{d x} = - \frac{1}{\sin y}$
- $\frac{d y}{d x} = - \frac{1}{\sqrt{1 - \cos^{2} y}}$
- $\frac{d y}{d x} = - \frac{1}{\sqrt{1 - x^{2}}}$
Notice how the derivative of $y = \arcsin x$ is positive but is negative for $y = \arccos x$
- This subtle but crucial difference can be seen in their graphs
  - $y = \arcsin x$ has a positive gradient for all values of $x$ in its domain
  - $y = \arccos x$ has a negative gradient for all values of $x$ in its domain

Examiner Tip

For $f (x) = \arctan x$ the terms on the denominator can be reversed (as they are being added rather than subtracted)
- $f' (x) = \frac{1}{1 + x^{2}} = \frac{1}{x^{2} + 1}$
- Don't be fooled by this, it sounds obvious but on awkward "show that" questions it can be off-putting!

Worked example

a) Show that the derivative of $\arctan x$ is $\frac{1}{1 + x^{2}}$

5-8-3-ib-hl-aa-only-we2a-soltn

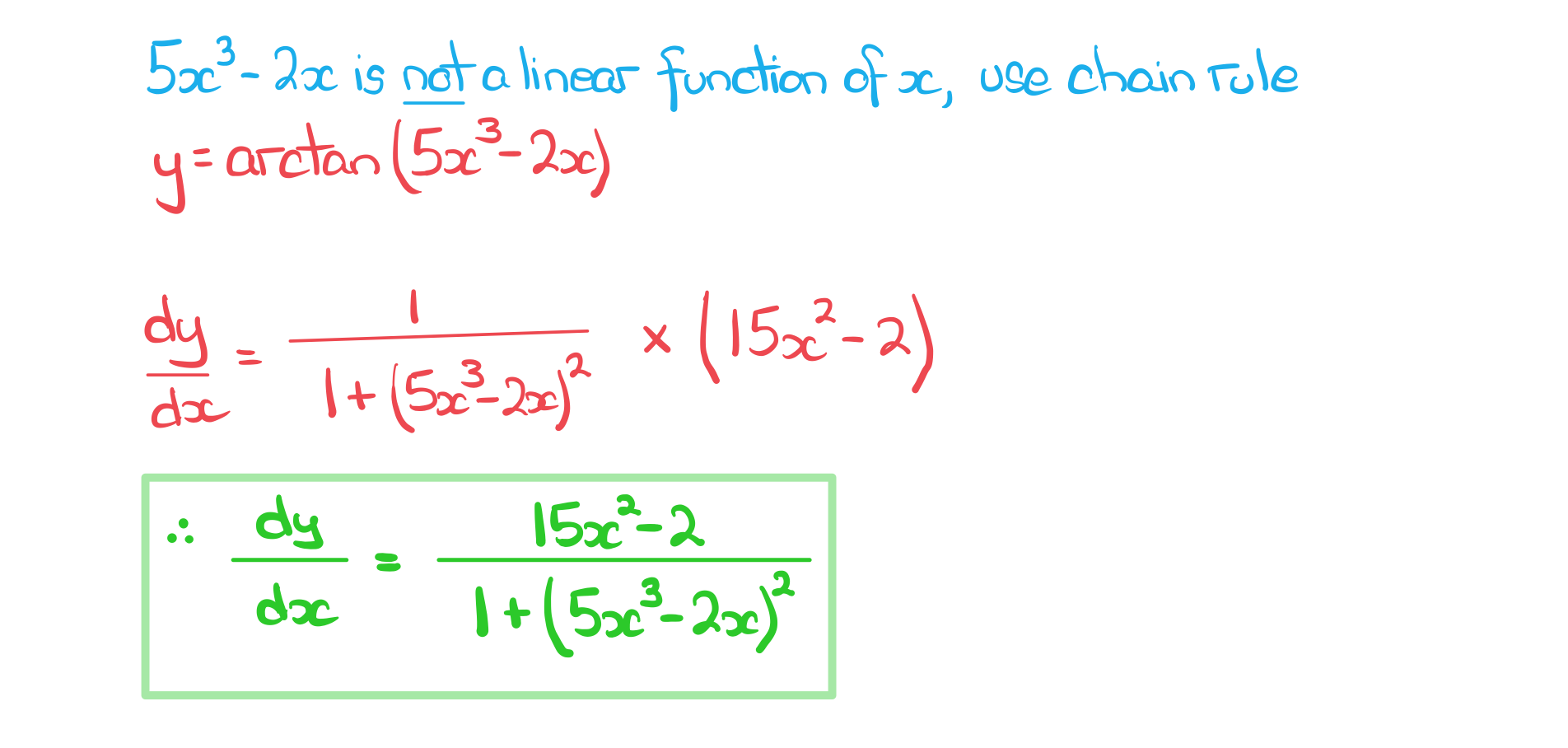
Find the derivative of

\arctan (5 x^{3} - 2 x)

5-8-3-ib-hl-aa-only-we2b-soltn

Integrating Inverse Trig Functions

How do I integrate inverse trig functions?

Use integration by parts in the same way you would integrate $\ln x$
These can be integrated using parts however
- rewrite as the product ‘ $1 \times f (x)$ ’ and choose $u = f (x)$ and $\frac{d v}{d x} = 1$
- 1 is easy to integrate and the inverse trig functions have standard derivatives listed in the formula booklet
The expression $\frac{x}{1 + x^{2}}$ integrates to $\frac{1}{2} \ln (1 + x^{2})$
The expression $\pm \frac{x}{\sqrt{1 - x^{2}}}$ integrates to $\mp \sqrt{1 - x^{2}}$

$\begin{array}{rcl} \int \arcsin (x) d x & = & x \arcsin (x) + \sqrt{1 - x^{2}} + c \\ \int arc \cos (x) d x & = & x arc \cos (x) - \sqrt{1 - x^{2}} + c \\ \int arc \tan (x) d x & = & x arc \tan (x) - \frac{1}{2} \ln (1 + x^{2}) + c \end{array}$

Calculus involving Inverse Trig (Edexcel A Level Further Maths: Core Pure)

Revision Note

Differentiating Inverse Trig Functions

What are the inverse trigonometric functions?

What are the derivatives of the inverse trigonometric functions?

How do I show or prove the derivatives of the inverse trigonometric functions?

Examiner Tip

Worked example

Integrating Inverse Trig Functions

How do I integrate inverse trig functions?

You've read 0 of your 10 free revision notes

Unlock more, it's free!

Join the 100,000+ Students that ❤️ Save My Exams

Author: Paul