Mean Value of a Function (Edexcel A Level Further Maths): Revision Note
Exam code: 9FM0
Mean value of a function
What is the mean value of a function?
The mean value of a function may be thought of as the ‘average’ value of a function over a given interval
For a function f(x), the mean value of the function over the interval [a, b] is given by
Note that the mean value
is simply a real number – it is not a function
The mean value depends on the interval chosen – if the interval [a, b] changes, then the mean value may change as well
Because
is a real number, the graph of
is a horizontal line
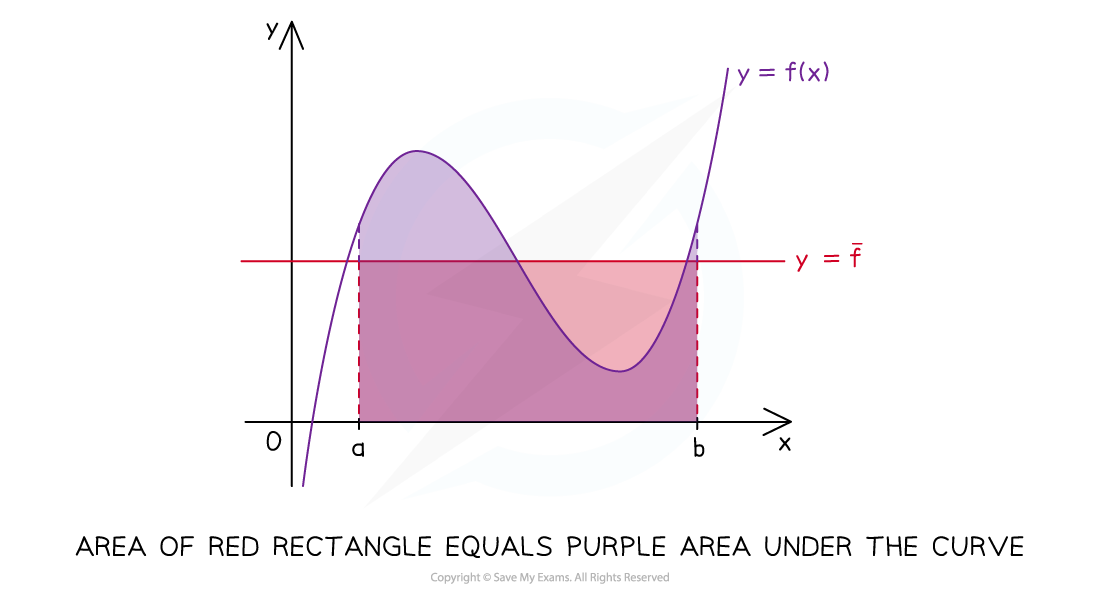
This gives a geometrical interpretation of the mean value of a function over a given interval
If A is the area bounded by the curve y = f(x), the x-axis and the lines x = a and x = b, then the rectangle with its base on the interval [a, b] and with height also has area A
i.e.
What are the properties of the mean value of a function?
If
is the mean value of a function f(x) over the interval [a, b], and k is a real constant, then:
f(x) + k has mean value
over the interval [a, b]
kf(x) has mean value
over the interval [a, b]
-f(x) has mean value
over the interval [a, b]
If
then the area that is above the x-axis and under the curve is equal to the area that is below the x-axis and above the curve
Worked Example
Let be the function defined by
.
a) Find the exact mean value of over the interval
.
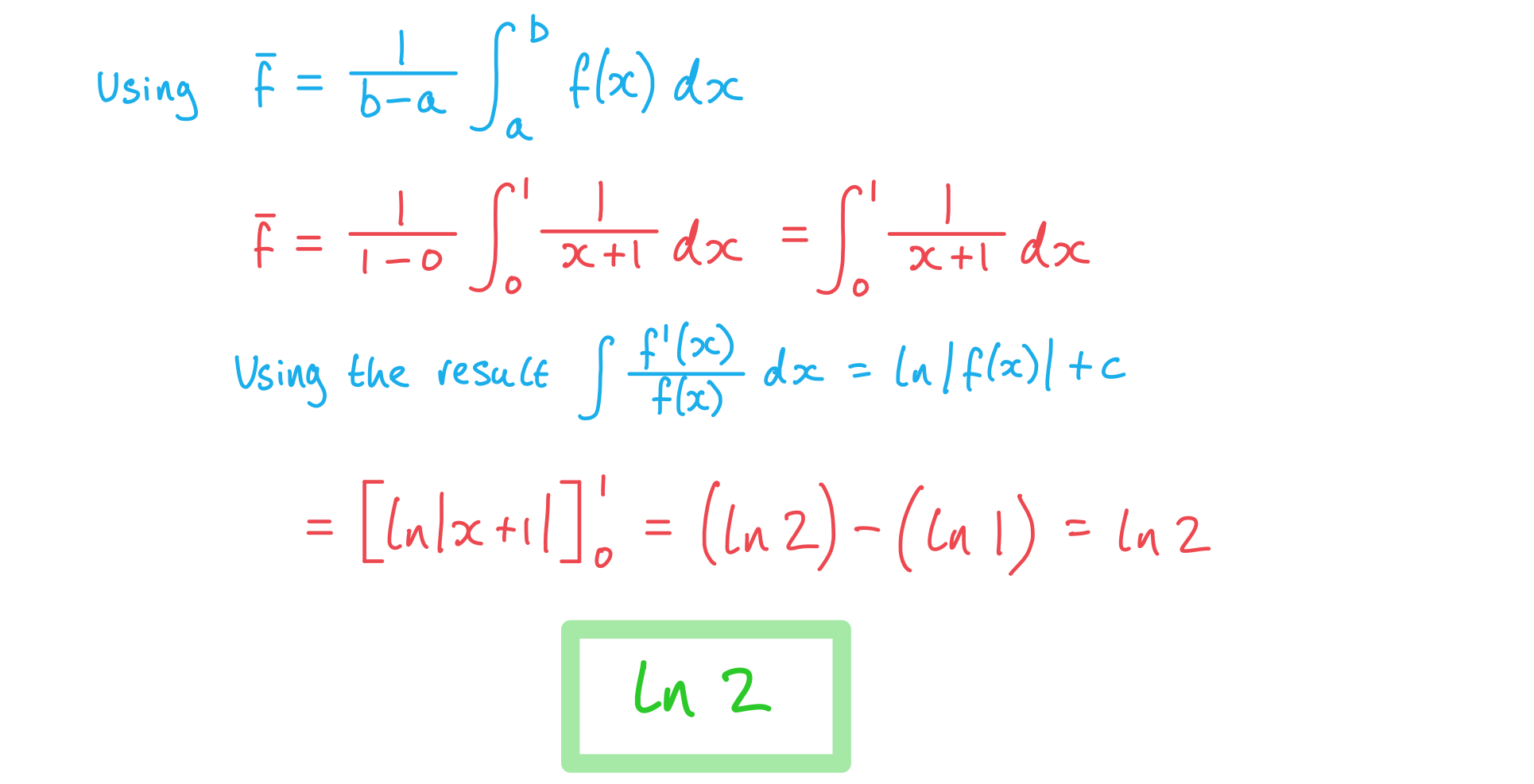
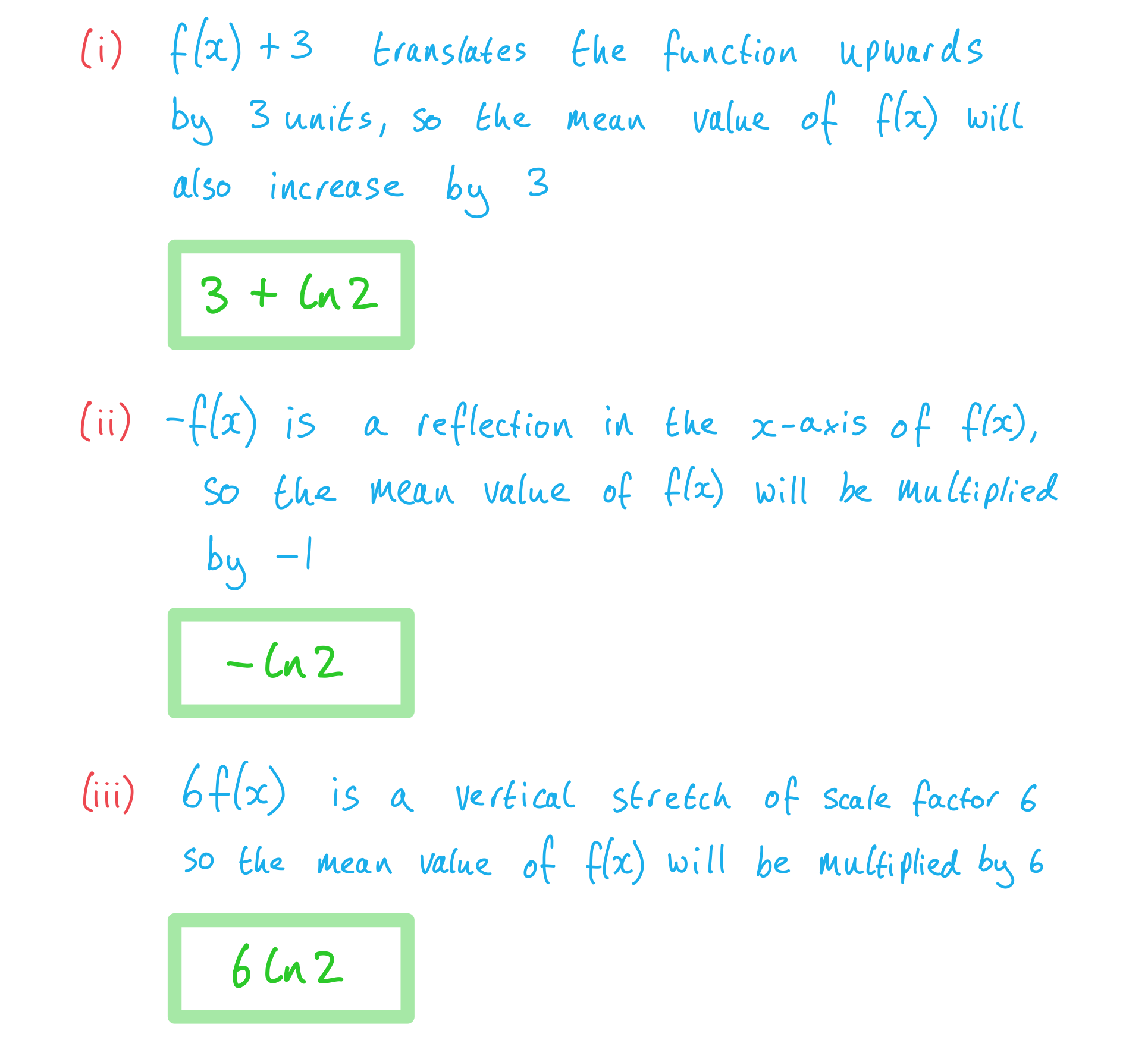
b) Write down the exact mean value of each of the following functions over the interval :
(i)
(ii)
(iii)

Unlock more, it's free!
Did this page help you?