Roots of Polynomials (Edexcel A Level Further Maths: Core Pure)

Revision Note

Test yourself

Author

Jamie W

Last updated

2 September 2022

Roots of Quadratics

How are the roots of a quadratic linked to its coefficients?

Because a quadratic equation $a x^{2} + b x + c = 0$ (where $a \neq 0$ ) has roots $α$ and $β$ , you can write this equation instead in the form $a (x - α) (x - β) = 0$
- - Note that $(x - α) (x - β) = x^{2} - (α + β) x + α β$
  - It is possible that the roots are repeated, i.e. that $α = β$
- You can then equate the two forms:
  - $a x^{2} + b x + c = a (x - α) (x - β)$
- Then (because $a \neq 0$ ) you can divide both sides of that by a and expand the brackets:
  - $x^{2} + \frac{b}{a} x + \frac{c}{a} = x^{2} - (α + β) x + α β$

- Finally, compare the coefficients
  - Coefficients of x: $- \frac{b}{a} = (α + β)$
  - Constant terms: $\frac{c}{a} = α β$

Therefore for a quadratic equation $a x^{2} + b x + c = 0$ :

- The sum of the roots $α + β$ is equal to $- \frac{b}{a}$
- The product of the roots $α β$ is equal to $\frac{c}{a}$
- Unless an exam question specifically asks you to prove these results, you can always use them without proof to answer questions about quadratics

Related Roots

You may be asked to consider two quadratic equations, with the roots of the second quadratic linked to the roots of the first quadratic in some way

- You are usually required to find the sum or product of the roots of the second equation

The strategy is to use identities which contain $α β$ and $α + β$ (where $α$ and $β$ are the roots of the first quadratic)

- If you know the values of $α$ and $β$ from the first quadratic, you can use them to help find the sum or product of the new roots
- If the second quadratic has roots $α^{2}$ and $β^{2}$ , then use the identities:
  - $(α + β)^{2} = α^{2} + β^{2} + 2 α β$ or $α^{2} + β^{2} = (α + β)^{2} - 2 α β$
  - $(α β)^{2} = α^{2} β^{2}$

- If the second quadratic has roots $α^{3}$ and $β^{3}$ , then use the identities:
  - $(α + β)^{3} = α^{3} + β^{3} + 3 α β (α + β)$
  - $(α β)^{3} = α^{3} β^{3}$

- If the second quadratic has roots $\frac{1}{α}$ and $\frac{1}{β}$ , then use the identities:
  - $\frac{1}{α} + \frac{1}{β} = \frac{α + β}{α β}$
  - $\frac{1}{α} \times \frac{1}{β} = \frac{1}{α β}$

You can then form a new equation for a quadratic with the new roots

This is done by recalling that a quadratic with a given pair of roots can be written in the form x² – (sum of the roots)x + (product of the roots) = 0
Be aware that this will not give a unique answer

This is because multiplying an entire quadratic by a constant does not change its roots
You can use this fact, for example, to find a quadratic that has a particular pair of roots AND has all integer coefficients

See the worked example below for an example of how to do some of this!

Worked example

The roots of an equation $a x^{2} + b x + c = 0$ are $α = - \frac{1}{2}$ and $β = 3$ .

Find integer values of a, b, and c.

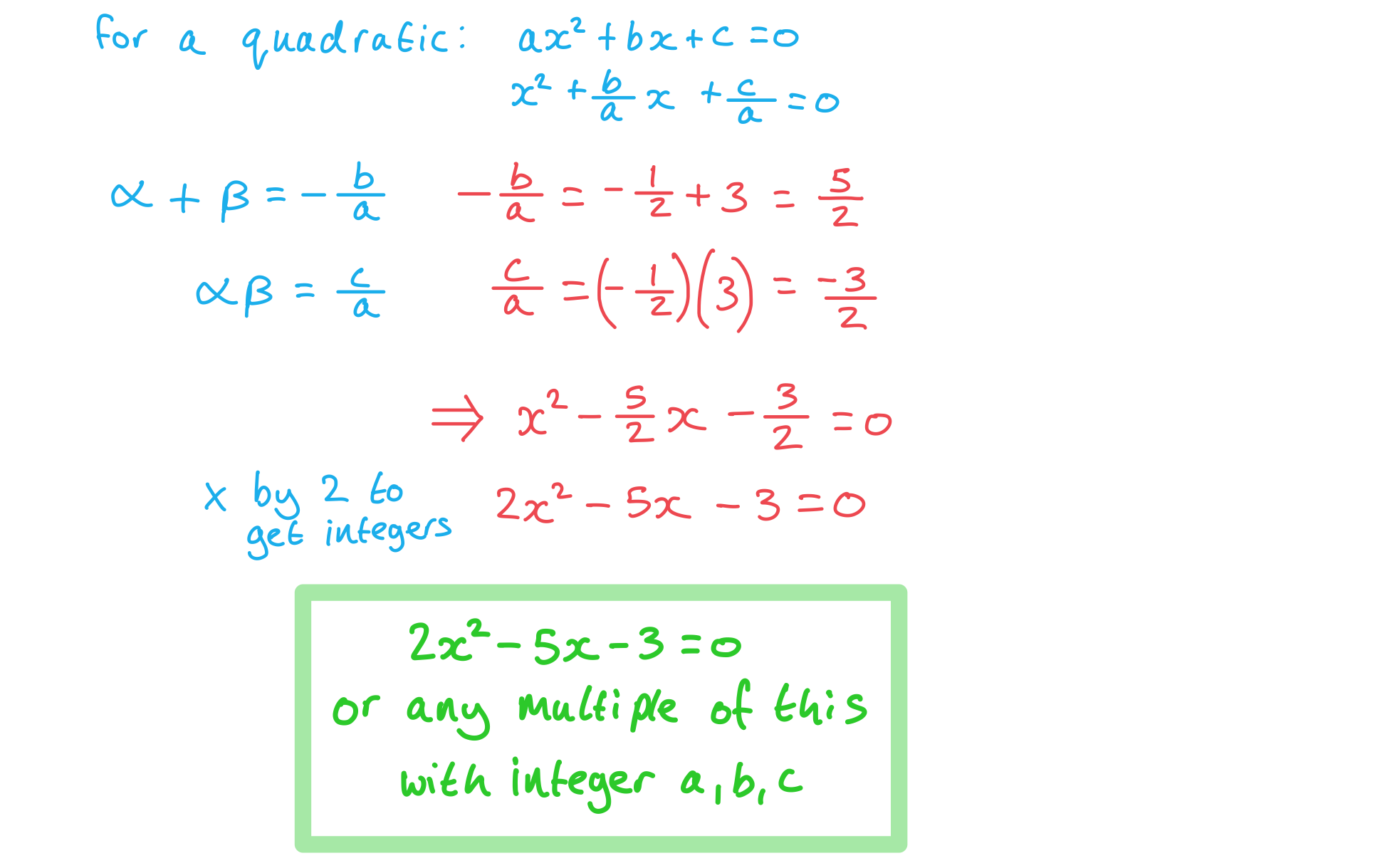
3-1-1-edx-a-fm-we1a-soltn

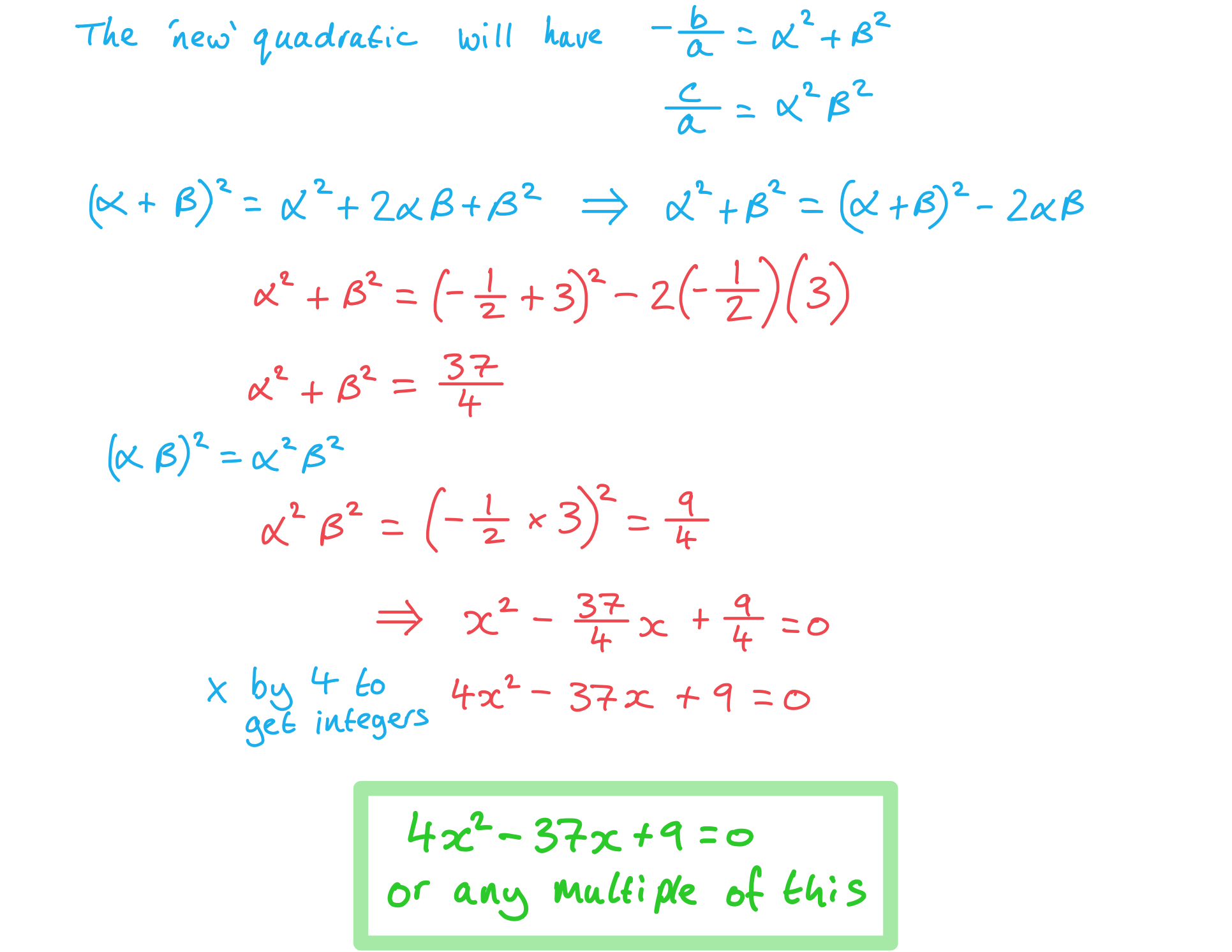
Hence find a quadratic equation whose roots are

α^{2}

and

β^{2}

3-1-1-edx-a-fm-we1b-soltn

Roots of Cubics

How are the roots of a cubic linked to its coefficients?

Because a cubic equation $a x^{3} + b x^{2} + c x + d = 0$ (where $a \neq 0$ ) has roots $α$ , $β$ and $γ$ , you can write this equation instead in the form $a (x - α) (x - β) (x - γ) = 0$

- - Note that $(x - α) (x - β) (x - γ) = x^{3} - (α + β + γ) x^{2} + (α β + β γ + α γ) x - α β γ$
  - It is possible that some of the roots are repeated, i.e. that some or all of them are equal to each other

- You can then equate the two forms:
  - $a x^{3} + b x^{2} + c x + d = a (x - α) (x - β) (x - γ)$
- Then (because $a \neq 0$ ) you can divide both sides of that by a and expand the brackets:
  - $x^{3} + \frac{b}{a} x^{2} + \frac{c}{a} x + \frac{d}{a} = x^{3} - (α + β + γ) x^{2} + (α β + β γ + α γ) x - α β γ$
- Finally, compare the coefficients
  - Coefficients of x²: $- \frac{b}{a} = α + β + γ$
  - Coefficients of x: $\frac{c}{a} = α β + β γ + α γ$
  - Constant terms: $- \frac{d}{a} = α β γ$

Therefore for a cubic equation $a x^{3} + b x^{2} + c x + d = 0$ :

- The sum of the roots $α + β + γ$ is equal to $- \frac{b}{a}$
  - The sum of roots $α + β + γ$ can also be denoted by $\sum_{}^{} α$
- The sum of the product pairs of roots $α β + β γ + α γ$ is equal to $\frac{c}{a}$
  - This ‘sum of pairs’ $α β + β γ + α γ$ can also be denoted by $\sum_{}^{} α β$
- The product of the roots $α β γ$ is equal to $- \frac{d}{a}$
  - The product of roots $α β γ$ can also be denoted by $\sum_{}^{} α β γ$
  - See quartic equations where using this ‘sum of triples’ notation makes more sense!
- Unless an exam question specifically asks you to prove these results, you can always use them without proof to answer questions about cubics

Related Roots

You may be asked to consider two cubic equations, with the roots of the second cubic linked to the roots of the first cubic in some way

- You are usually required to find the sum or product of the roots of the second equation

The strategy is to use identities which contain $\sum_{}^{} α$ , $\sum_{}^{} α β$ , and $\sum_{}^{} α β γ$ (where $α$ , $β$ and $γ$ are the roots of the first cubic)

- If you know the values of $α$ , $β$ , and $γ$ from the first cubic, you can use them to help find the sum or product of the new roots

- If the second cubic has roots $α^{2}$ , $β^{2}$ , and $γ^{2}$ , then use the identities:
  - $(α + β + γ)^{2} = α^{2} + β^{2} + γ^{2} + 2 (α β + β γ + α γ)$

- - i.e., $(α + β + γ)^{2} = α^{2} + β^{2} + γ^{2} + 2 \sum α β$
  - $(α β γ)^{2} = α^{2} β^{2} γ^{2}$

- If the second cubic has roots $α^{3}, β^{3}, γ^{3}$ , then use the identities:
  - $(α + β + γ)^{3} = α^{3} + β^{3} + γ^{3} + 3 (α + β + γ) (α β + β γ + α γ) - 3 α β γ$
  - i.e., $(α + β + γ)^{3} = α^{3} + β^{3} + γ^{3} + 3 \sum α \sum α β - 3 \sum α β γ$
  - $(α β γ)^{3} = α^{3} β^{3} γ^{3}$

- If the second cubic has roots $\frac{1}{α}$ , $\frac{1}{β}$ , and $\frac{1}{γ}$ , then use the identities:
  - $\frac{1}{α} + \frac{1}{β} + \frac{1}{γ} = \frac{α β + β γ + γ α}{α β γ}$
  - $\frac{1}{α} \times \frac{1}{β} \times \frac{1}{γ} = \frac{1}{α β γ}$

You can then form a new equation for a cubic with the new roots

This is done by recalling that a cubic with three given roots can be written in the form $x^{3} - (sum of roots) x^{2} + (sum of product pairs) x - (product of roots) = 0$
Be aware that this will not give a unique answer

This is because multiplying an entire cubic by a constant does not change its roots
You can use this fact, for example, to find a cubic that has a particular pair of roots AND has all integer coefficients

Worked example

a) Given the cubic equation

x^{3} + 3 x^{2} - 10 x - 24 = 0

, find

\sum_{}^{} α

\sum_{}^{} α β

, and

\sum_{}^{} α β γ

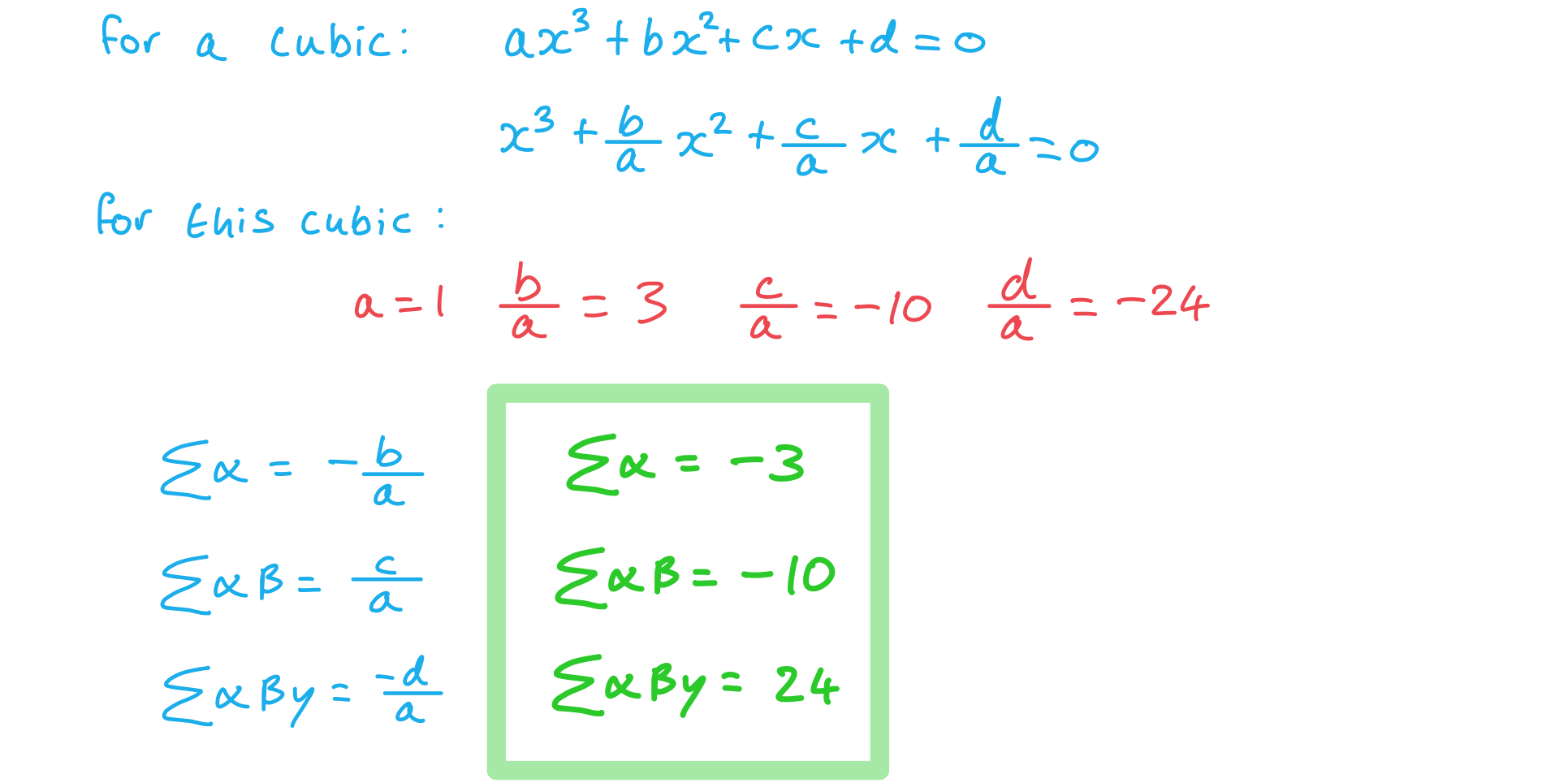
3-1-1-edx-a-fm-we2a-soltn

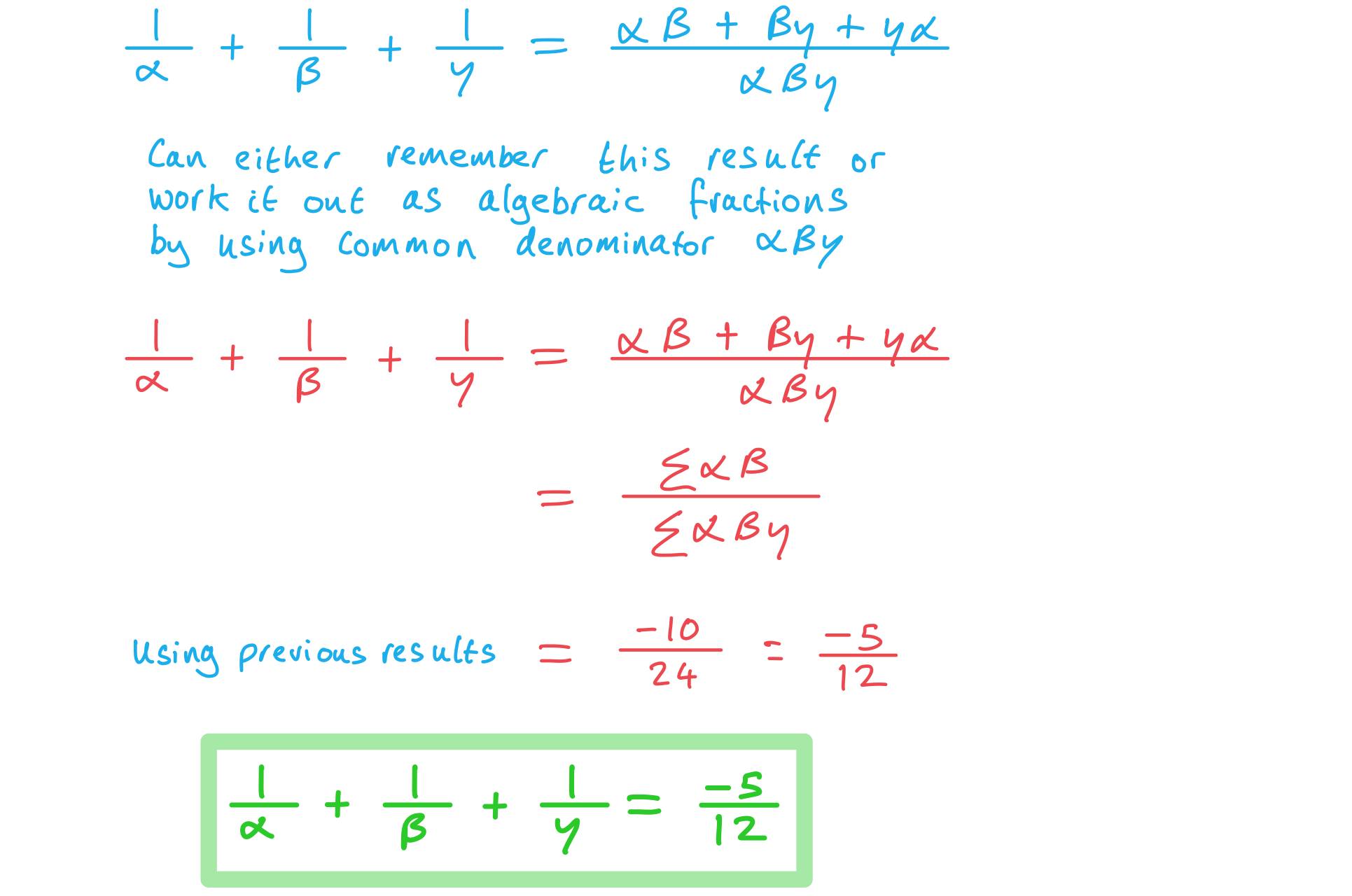
b) Another cubic has roots

\frac{1}{α}

\frac{1}{β}

, and

\frac{1}{γ}

. Find

\frac{1}{α} + \frac{1}{β} + \frac{1}{γ}

3-1-1-edx-a-fm-we2b-soltn

Roots of Quartics

How are the roots of a quartic linked to its coefficients?

Because a quartic equation $a x^{4} + b x^{3} + c x^{2} + d x + e = 0$ (where $a \neq 0$ ) has roots $α$ , $β$ , $γ$ and $δ$ , you can write this equation instead in the form $a (x - α) (x - β) (x - γ) (x - δ) = 0$

- - Note that $(x - α) (x - β) (x - γ) (x - δ)$ expands to $x^{4} - (α + β + γ + δ) x^{3} + (α β + β γ + α γ + γ δ + α δ + β δ) x^{2} - (α β γ + α β δ + α γ δ + β γ δ) x + α β γ δ$
  - It is possible that some of the roots are repeated, i.e. that some or all of them are equal to each other

- You can then equate the two forms:
  - $a x^{4} + b x^{3} + c x^{2} + d x + e = a (x - α) (x - β) (x - γ) (x - δ)$
- Then (because $a \neq 0$ ) you can divide both sides of that by a and expand the brackets:
  - $x^{3} + \frac{b}{a} x^{2} + \frac{c}{a} x + \frac{d}{a} =$ $x^{4} - (α + β + γ + δ) x^{3} + (α β + β γ + α γ + γ δ + α δ + β δ) x^{2} - (α β γ + α β δ + α γ δ + β γ δ) x + α β γ δ$
- Finally, compare the coefficients
  - Coefficients of x³: $- \frac{b}{a} = α + β + γ + δ$
  - Coefficients of x²: $\frac{c}{a} = α β + β γ + α γ + γ δ + α δ + β δ$
  - Coefficients of x: $- \frac{d}{a} = α β γ + α β δ + α γ δ + β γ δ$
  - Constant terms: $\frac{e}{a} = α β γ δ$

Therefore for a quartic equation $a x^{4} + b x^{3} + c x^{2} + d x + e = 0$ :

- The sum of the roots $α + β + γ + δ$ is equal to $- \frac{b}{a}$
  - The sum of roots $α + β + γ + δ$ can also be denoted by $\sum α$
- The sum of the product pairs of roots $α β + β γ + α γ + γ δ + α δ + β δ$ is equal to $\frac{c}{a}$
  - This ‘sum of pairs’ $α β + β γ + α γ + γ δ + α δ + β δ$ can also be denoted by $\sum α β$
- The sum of the product triples of roots $α β γ + α β δ + α γ δ + β γ δ$ is equal to $- \frac{d}{a}$
  - This ‘sum of triples’ can also be denoted by $\sum α β γ$
- The product of the roots $α β γ δ$ is equal to $\frac{e}{a}$
  - The product of roots (or ‘product of fours’) $α β γ δ$ can also be denoted by $\sum α β γ δ$
- Unless an exam question specifically asks you to prove these results, you can always use them without proof to answer questions about quartics

Related Roots

You may be asked to consider two quartic equations, with the roots of the second quartic linked to the roots of the first quartic in some way
- You are usually required to find the sum or product of the roots of the second equation

The strategy is to use identities which contain $\sum α$ , $\sum α β$ , $\sum α β γ$ , and $\sum α β γ δ$ (where $α$ , $β$ , $γ$ and $δ$ are the roots of the first quartic)
- If you know the values of $α$ , $β$ , $γ$ , and $δ$ from the first quartic, you can use them to help find the sum or product of the new roots
- If the second quartic has roots $α^{2}$ , $β^{2}$ , $γ^{2}$ and $δ^{2}$ , then use the identities:
  - $(α + β + γ + δ)^{2} = α^{2} + β^{2} + γ^{2} + δ^{2} + 2 (α β + β γ + α γ + γ δ + α δ + β δ)$
  - i.e., $(α + β + γ + δ)^{2} = α^{2} + β^{2} + γ^{2} + δ^{2} + 2 \sum α β$
  - $(α β γ δ)^{2} = α^{2} β^{2} γ^{2} δ^{2}$

- (Note that you will not be asked about a quartic with roots $α^{3}, β^{3}, γ^{3}$ and $δ^{3}$ )
- If the second quartic has roots $\frac{1}{α}$ , $\frac{1}{β}$ , $\frac{1}{γ}$ and $\frac{1}{δ}$ , then use the identities:
  - $\frac{1}{α} + \frac{1}{β} + \frac{1}{γ} + \frac{1}{δ} = \frac{α β γ + α β δ + α γ δ + β γ δ}{α β γ δ}$
  - $\frac{1}{α} \times \frac{1}{β} \times \frac{1}{γ} \times \frac{1}{δ} = \frac{1}{α β γ δ}$

You can then form a new equation for a quartic with the new roots

This is done by recalling that a quartic with four given roots can be written in the form $x^{4} - (sum of roots) x^{3} + (sum of product pairs) x^{2} - (sum of product triples) x + (product of roots) = 0$
Be aware that this will not give a unique answer

This is because multiplying an entire quartic by a constant does not change its roots
You can use this fact, for example, to find a quartic that has a particular pair of roots AND has all integer coefficients

Worked example

a) The roots of

x^{4} - 12 x^{3} + 33 x^{2} + 38 x - 168 = 0

are

α, β, γ

and

δ

. Find

{\sum α,}_{}^{} {\sum α β,}_{}^{} {\sum α β γ}_{}^{}

, and

{\sum α β γ δ}_{}^{}

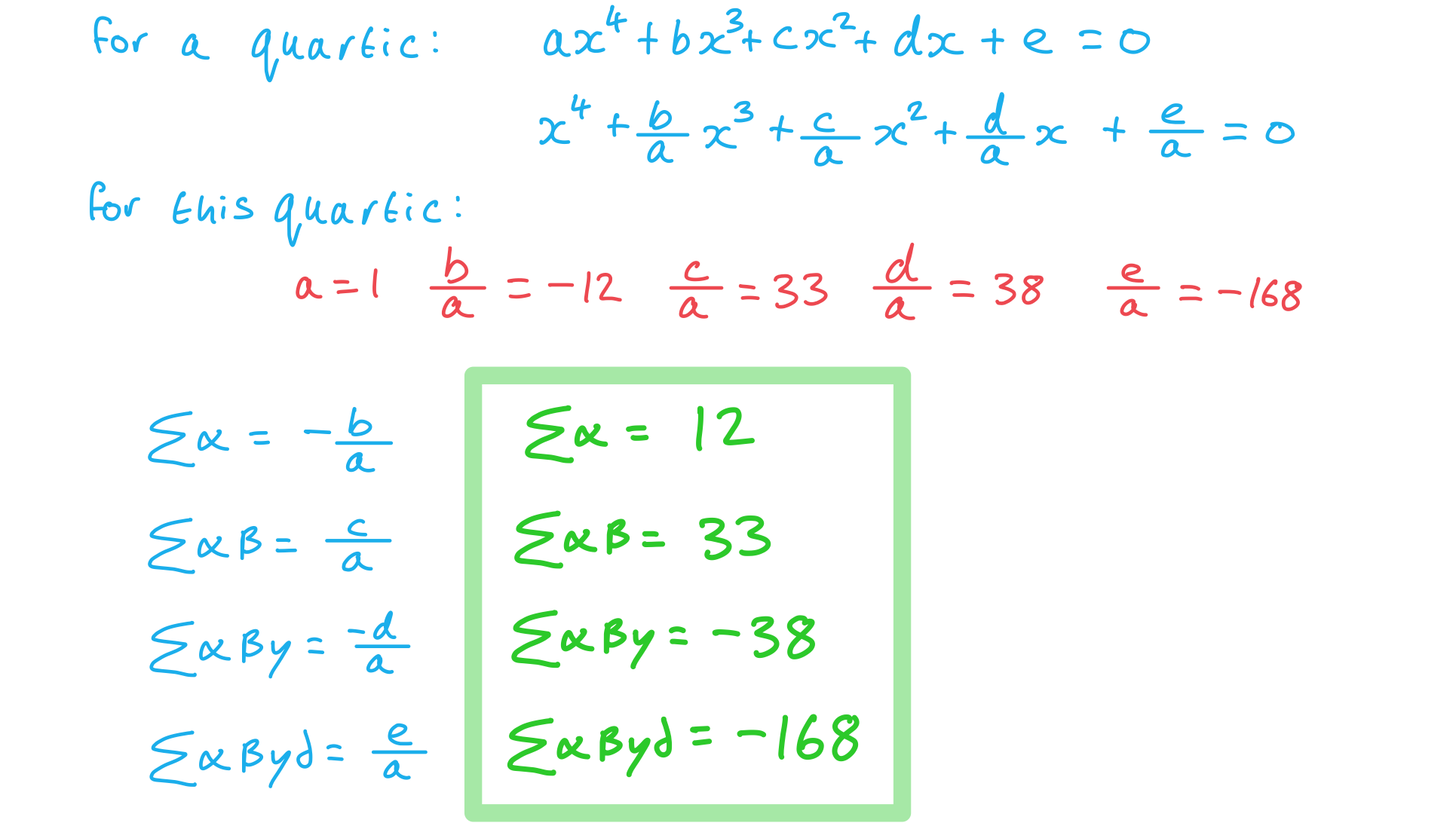
3-1-1-edx-a-fm-we3a-soltn

Another quartic has roots $α^{2}, β^{2}, γ^{2}$ and $δ^{2}$ . Find the value of $α^{2} + β^{2} + γ^{2} + δ^{2}$ .

3-1-1-edx-a-fm-we3b-soltn

Roots of Polynomials

What is the general pattern linking the roots to the coefficients of a polynomial?

By looking at the links between the coefficients and the roots of quadratics, cubics, and quartics, you can see that a pattern emerges, which also holds true for higher order polynomials
It is useful to use sigma notation to keep expressions for sums of roots concise
- For a quartic with roots $α, β, γ, δ$ , for example:
  - The sum of the roots $α + β + γ + δ$ is denoted by $\sum α$
  - The sum of the pairs of roots $α β + β γ + α γ + γ δ + α δ + β δ$ is denoted by $\sum α β$
  - The sum of the triples of roots $α β γ + α β δ + α γ δ + β γ δ$ is denoted by $\sum α β γ$
  - The sum of the sets of fours (in this case just one term) $α β γ δ$ is denoted by $\sum α β γ δ$
The table below summarises the relationships between the coefficients and roots of quadratics, cubics, and quartics:

$a x^{2} + b x + c = 0$

Roots $α, β$

$a x^{3} + b x^{2} + c x + d = 0$

Roots $α, β, γ$

$a x^{4} + b x^{3} + c x^{2} + d x + e = 0$

Roots $α, β, γ, δ$

\sum α

$α + β$

$= - \frac{b}{a}$

$α + β + γ$

$= - \frac{b}{a}$

$α + β + γ + δ$

$= - \frac{b}{a}$

\sum α β

$α β$

$= \frac{c}{a}$

$α β + β γ + α γ$

$= \frac{c}{a}$

$α β + β γ + α γ + γ δ + α δ + β δ$

$= \frac{c}{a}$

\sum α β γ

$α β γ$

$= - \frac{d}{a}$

$α β γ + α β δ + α γ δ + β γ δ$

$= - \frac{d}{a}$

\sum α β γ δ

$α β γ δ$

$= \frac{e}{a}$

How can I find sums and products of related roots?

You may be asked to consider a second equation, that has roots linked to the roots of the first equation in some way
- You are usually required to find the sum or product of the roots of the second equation

The strategy is to use identities containing $\sum α, \sum α β, \sum α β γ$ , and/or $\sum α β γ δ$ (depending on the question and the degree of the polynomial)
- If you know the value of the roots from the first equation, these identities can help you find the sum or product of the roots of the second equation
The table below shows useful identities for finding a new quadratic equation whose roots are related to the roots $α$ and $β$ of the original quadratic equation
- In each case the sum or the product of the ‘new roots’ can be linked back to $α β$ or $α + β$ for the original equation

Roots of new Equation	Useful Identities to find sums and products of new roots
$\frac{1}{α}, \frac{1}{β}$	$\frac{1}{α} + \frac{1}{β} = \frac{α + β}{α β}$ $\frac{1}{α} \times \frac{1}{β} = \frac{1}{α β}$
$α^{2}, β^{2}$	$α^{2} + β^{2} = (α + β)^{2} - 2 α β$ $α^{2} β^{2} = (α β)^{2}$
$α^{3}, β^{3}$	$α^{3} + β^{3} = (α + β)^{3} - 3 α β (α + β)$ $α^{3} β^{3} = (α β)^{3}$

Similar identities that could be useful for cubics and quartics are listed earlier in this revision note in the cubics and quartics sections
A good place to start if the new roots are squared, is by considering $(\sum α)^{2}$

or if the new roots are cubed, then start by considering $(\sum α)^{3}$
or if the new roots are reciprocals (i.e., $\frac{1}{α}, \frac{1}{β}$ , etc.), then start by adding the new roots together to form a single algebraic fraction

Examiner Tip

Although you may be asked to tackle questions on this topic showing full working and without relying on a calculator, you can still use your calculator to check your work by finding the roots of a polynomial in the polynomial solver
You can then use these to check your answers for sums of roots, products of roots, etc.

Worked example

Given a polynomial equation of order 5 (a quintic);

a x^{5} + b x^{4} + c x^{3} + d x^{2} + e x + f = 0

, make 5 conjectures linking the coefficients a, b, c, d, e, f to its roots

α, β, γ, δ, ε

3-1-1-edx-a-fm-we4a-soltn

Test your conjectures on the example:

x^{5} - 15 x^{4} + 85 x^{3} - 225 x^{2} + 274 x - 120 = 0

which has roots

x = 1, 2, 3, 4, 5

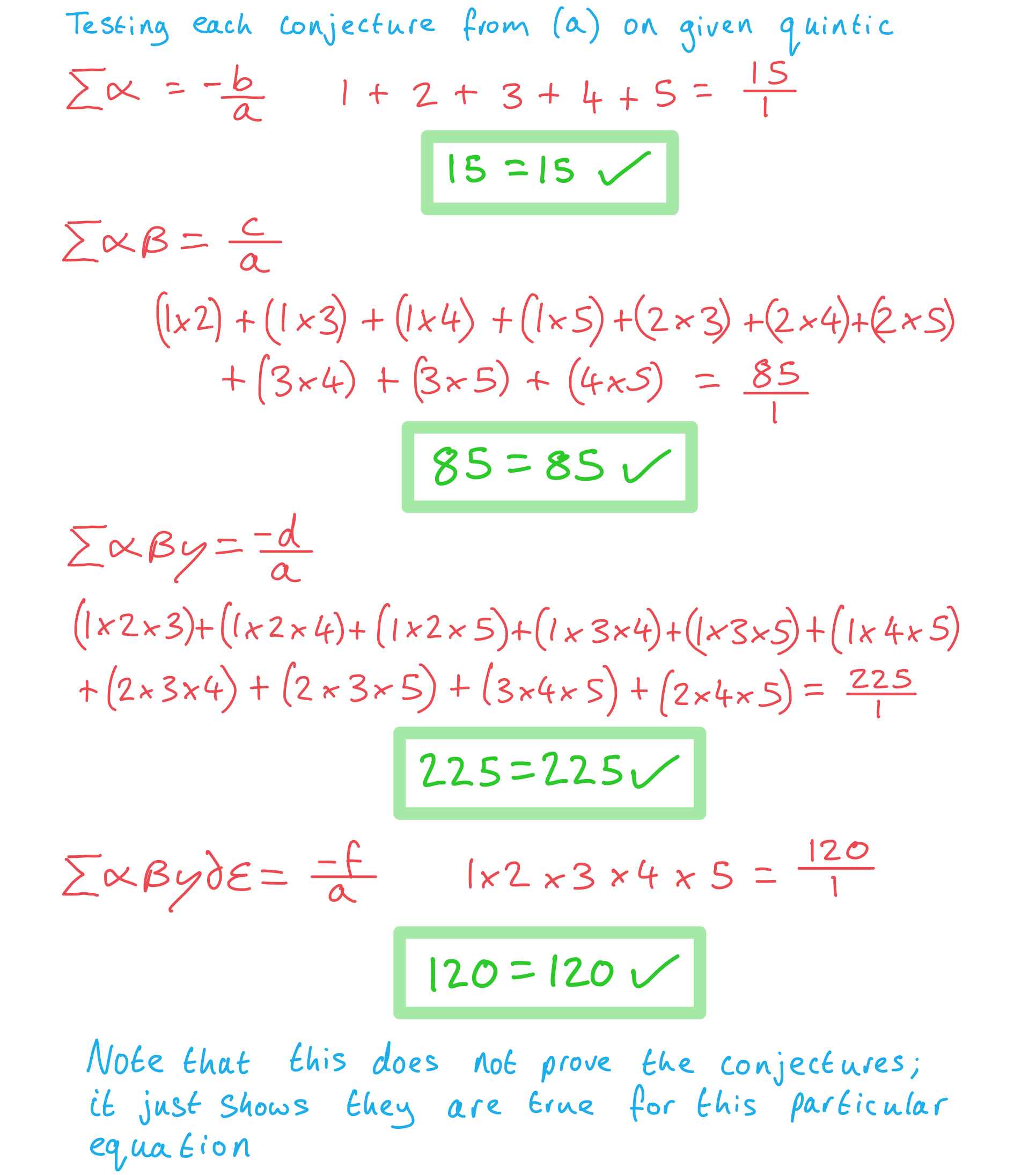
3-1-1-edx-a-fm-we4b-soltn

You've read 0 of your 10 free revision notes

Unlock more, it's free!

Join the 100,000+ Students that ❤️ Save My Exams

the (exam) results speak for themselves:

Test yourself Next topic

Did this page help you?

Roots of Polynomials (Edexcel A Level Further Maths: Core Pure)

Revision Note

How are the roots of a quadratic linked to its coefficients?

How are the roots of a cubic linked to its coefficients?

How are the roots of a quartic linked to its coefficients?

What is the general pattern linking the roots to the coefficients of a polynomial?

How can I find sums and products of related roots?

You've read 0 of your 10 free revision notes

Unlock more, it's free!

Join the 100,000+ Students that ❤️ Save My Exams

Author: Jamie W