Regions in Argand Diagrams | Edexcel A Level Further Maths: Core Pure Revision Notes 2017

Inequalities & Regions in Argand Diagrams

How do I sketch inequalities such as $Re z < k$ or $Im z < k$ on an Argand diagram?

The inequality $Re z < 2$ is satisfied by all complex numbers, $z = x + i y$ , with a real part less than 2
- On an Argand diagram, this would be the region to the left of the vertical line $x = 2$
- The vertical line itself, $x = 2$ , should be drawn dotted to show that points on this line are not permitted due to the strict inequality (<)
In general, dotted lines are used with strict inequalities ( < or > ) and solid lines are used with inequalities that can be equal ( ≤ or ≥ )
Here is how to represent the following inequalities as regions on an Argand diagram…
- $Re z < k$ : shade the region to the left of the dotted vertical line (or solid vertical line for ≤)
- $Re z > k$ : shade the region to the right of the dotted vertical line (or solid vertical line for ≥)
Similarly, here is how to represent the following inequalities as regions on an Argand diagram…
- $Im z < k$ : shade the region below the dotted horizontal line (or solid horizontal line for ≤)
- $Im z > k$ : shade the region above the dotted horizontal line (or solid horizontal line for ≥)

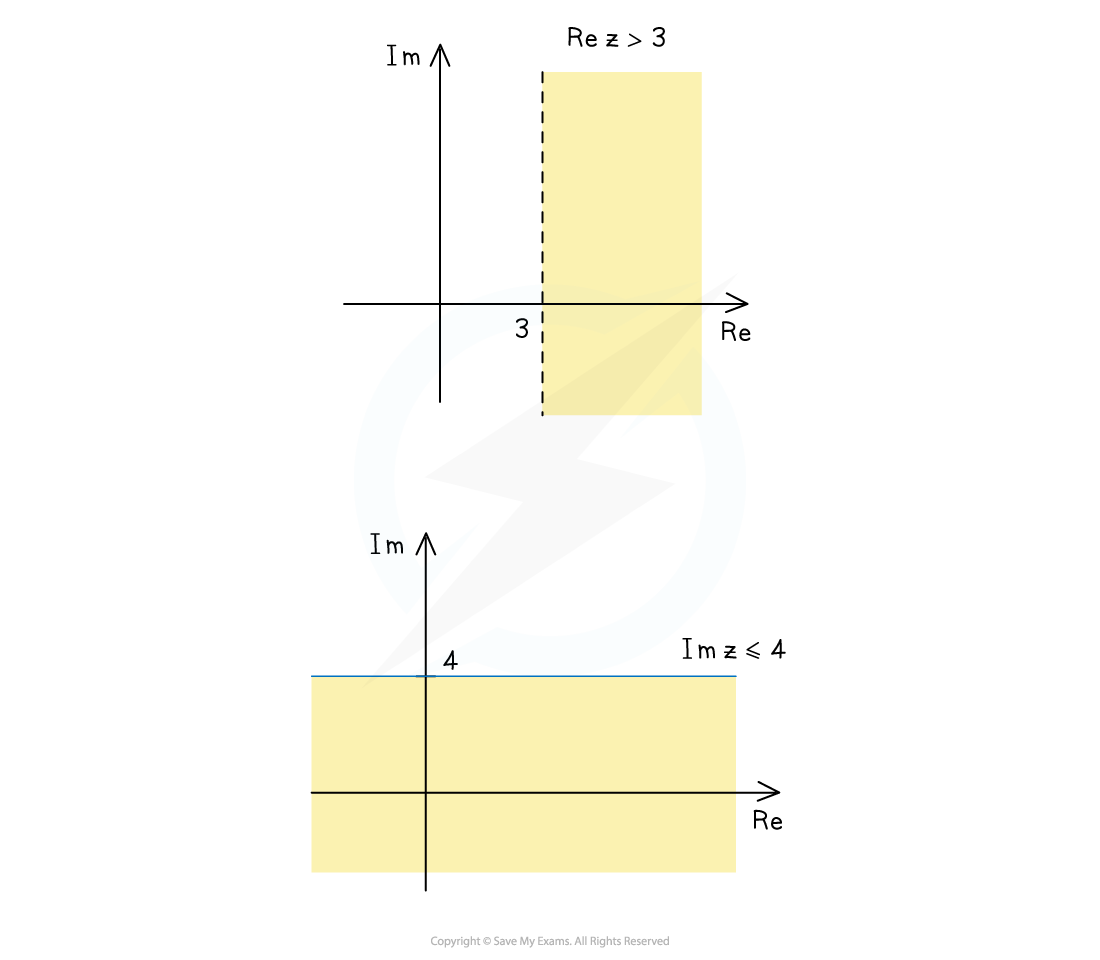
8-2-5_notes_fig1

Sketching the inequalities $Re z > 3 a n d Im z \leq 4$

How do I sketch inequalities such as $|z - a| < k$ on an Argand diagram?

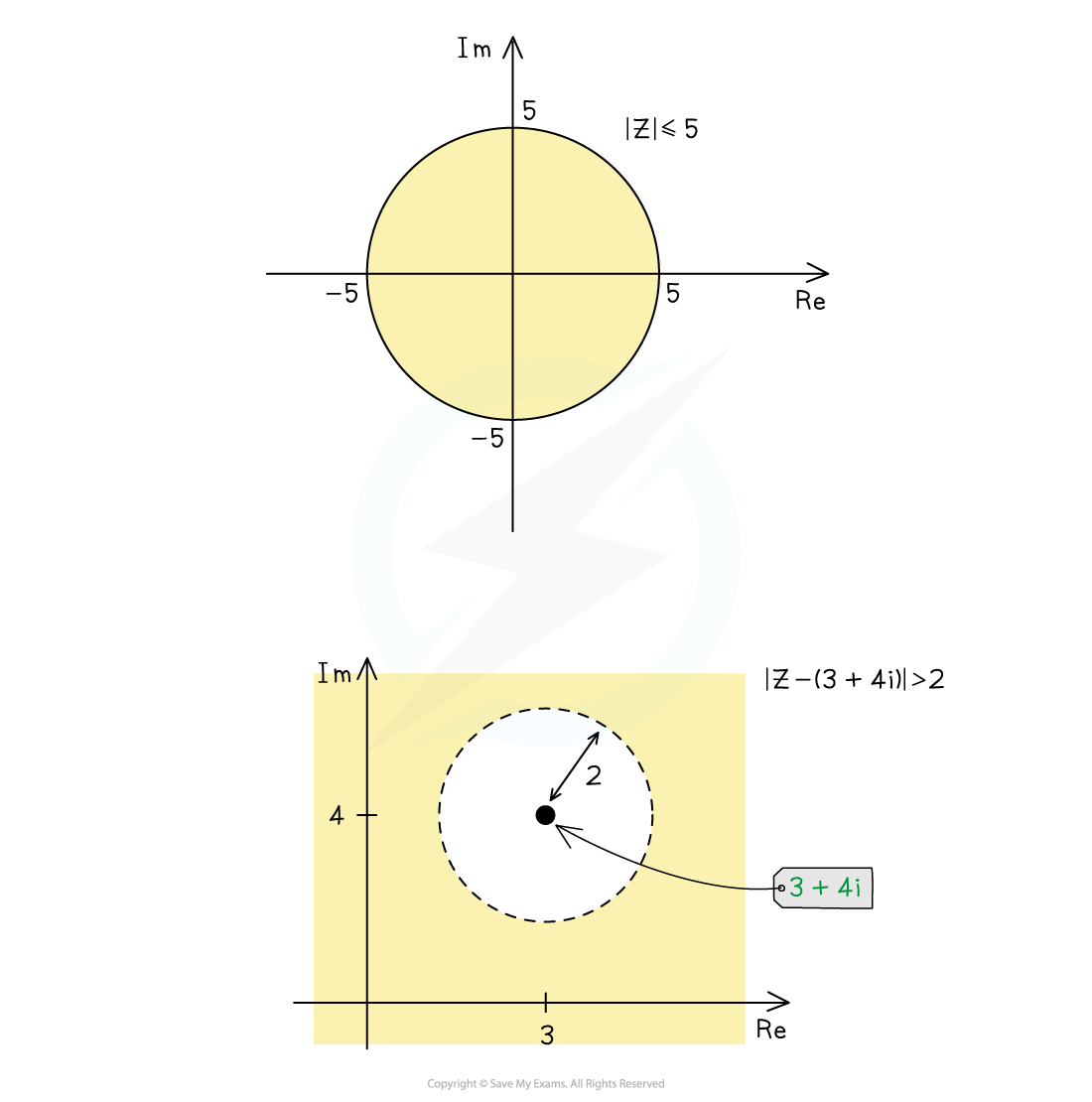
For a given complex number $a$ , the inequality $|z - a| < k$ represents all complex numbers, $z$ , that lie inside the circle of radius $k$ , centred at $a$
Here is how to represent the following inequalities as regions on an Argand diagram…
- $|z - a| < k$ : shade the region inside the circle of radius $k$ , centred at $a$
- $|z - a| > k$ : shade the (unbounded) region outside circle of radius $k$ , centred at
- Again use a dotted line for strict inequalities (< and >) and a solid line for weak inequalities ( ≤ or ≥ )

8-2-5_notes_fig2

Sketching the inequalities $| z | \leq 5$ and $| z - (3 + 4 i) | > 2$

How do I sketch inequalities such as $| z - a | < | z - b |$ on an Argand diagram?

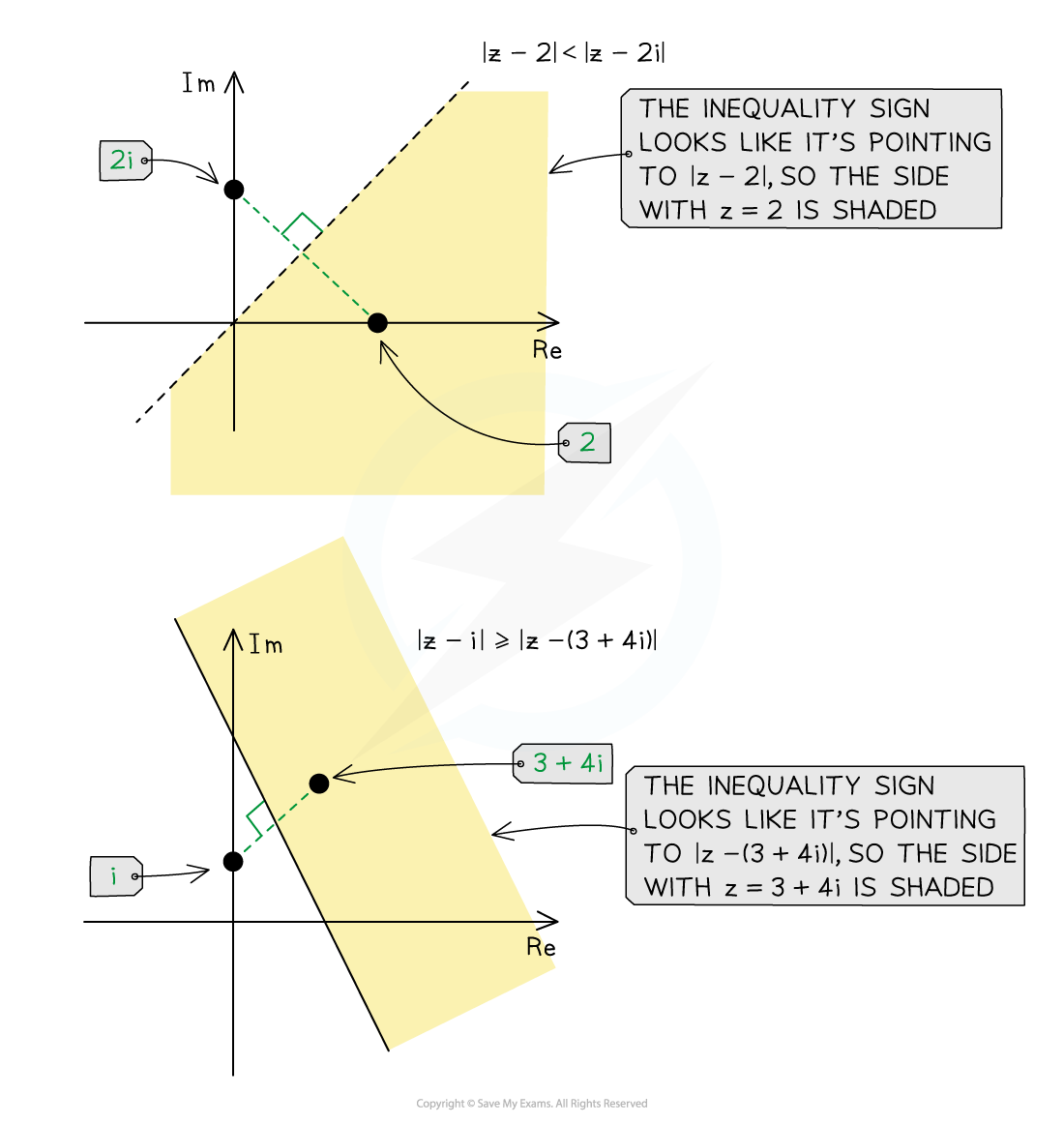
For two given complex numbers $a$ and $b$ , the inequality $|z - a| < | z - b |$ represents all complex numbers, $z$ , that lie closer to $a$ than to $b$
Here is how to represent the following inequalities as regions on an Argand diagram…
- $| z - a | < | z - b |$ : shade the region on the side of the perpendicular bisector of $a$ and $b$ that includes the point $a$
- $| z - a | > | z - b |$ : shade the region on the side of the dotted perpendicular bisector of $a$ and $b$ that includes the point $b$
A good way to remember which side to shade is that the inequality sign points (like an arrow) to the side to be shaded

Sketching the inequalities $| z - 2 | < | z - 2 i |$ and $| z - i | \geq | z - (3 + 4 i) |$

How do I sketch inequalities such as $α < \arg (z - a) < β$ on an Argand diagram?

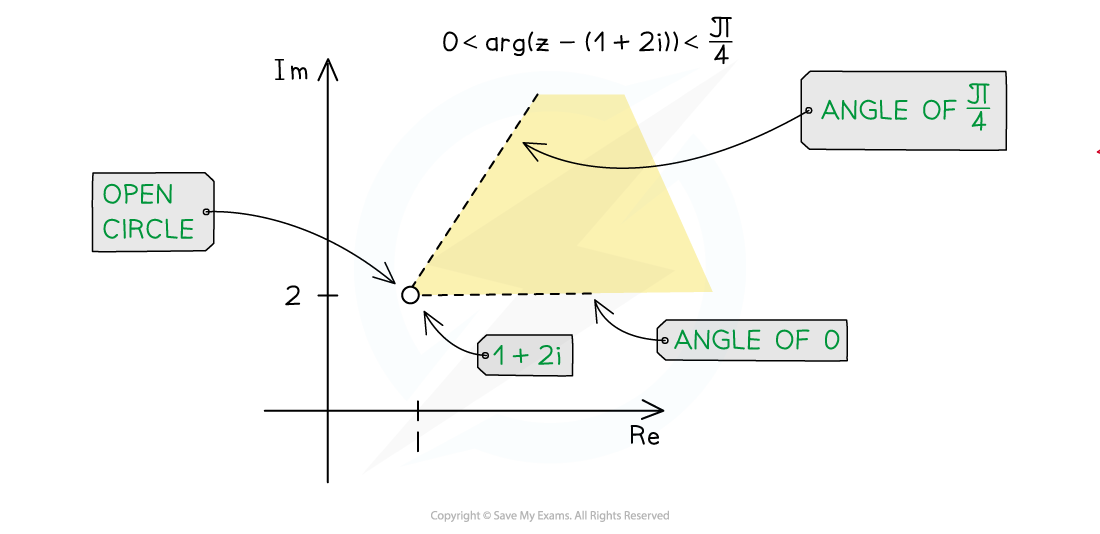
For a given complex number, $a$ , the inequality $α < \arg (z - a) < β$ represents all complex numbers, $z$ , that have an argument between $α$ and $β$ , as measured from the point $α$
Here is how to represent the following inequalities as regions on an Argand diagram…
- $α < \arg (z - a) < β$ : shade the wedge-shaped region between the half-line $\arg (z - a) = α$ and the half-line $\arg (z - a) = β$ , with an open circle at $z = a$ to show the exclusion of this point (to avoid the undefined value of $\arg 0$ )

8-2-5_notes_fig4

Sketching the inequalities $0 < \arg (z - (1 + 2 i)) < \frac{π}{4}$

How do I draw multiple inequalities on the same Argand diagram?

To sketch a region that satisfies multiple inequalities, we need to find the intersection of all the regions (where all regions overlap)
- E.g. the region satisfied by $2 < |z| \leq 5$ can be found as follows…
  - Using diagonal lines in the same direction, lightly shade the region $| z | > 2$ (the outside of a circle of radius 2 about the origin)
  - Using diagonal lines in a different direction, lightly shade the region $|z| \leq 5$ (the inside of a circle of radius 5 about the origin)
  - Where the diagonal lines cross each other highlights the region satisfying both inequalities; this should be shaded boldly
- E.g. the region satisfied by $|z - i| < | z - 1 |$ and $0 \leq \arg z \leq \frac{3 π}{4}$ can be found as follows…
  - Using diagonal lines in the same direction, lightly shade the region $|z - i| < | z - 1 |$ (the side including $z = i$ from the perpendicular bisector of the points $i$ and 1)
  - Using diagonal lines in a different direction, lightly shade the region $0 \leq \arg z \leq \frac{3 π}{4}$ (the wedge-shaped region from 0 to $\frac{3 π}{4}$ radians from the origin)
  - Where the diagonal lines cross each other highlights the region satisfying both inequalities; this should be shaded boldly

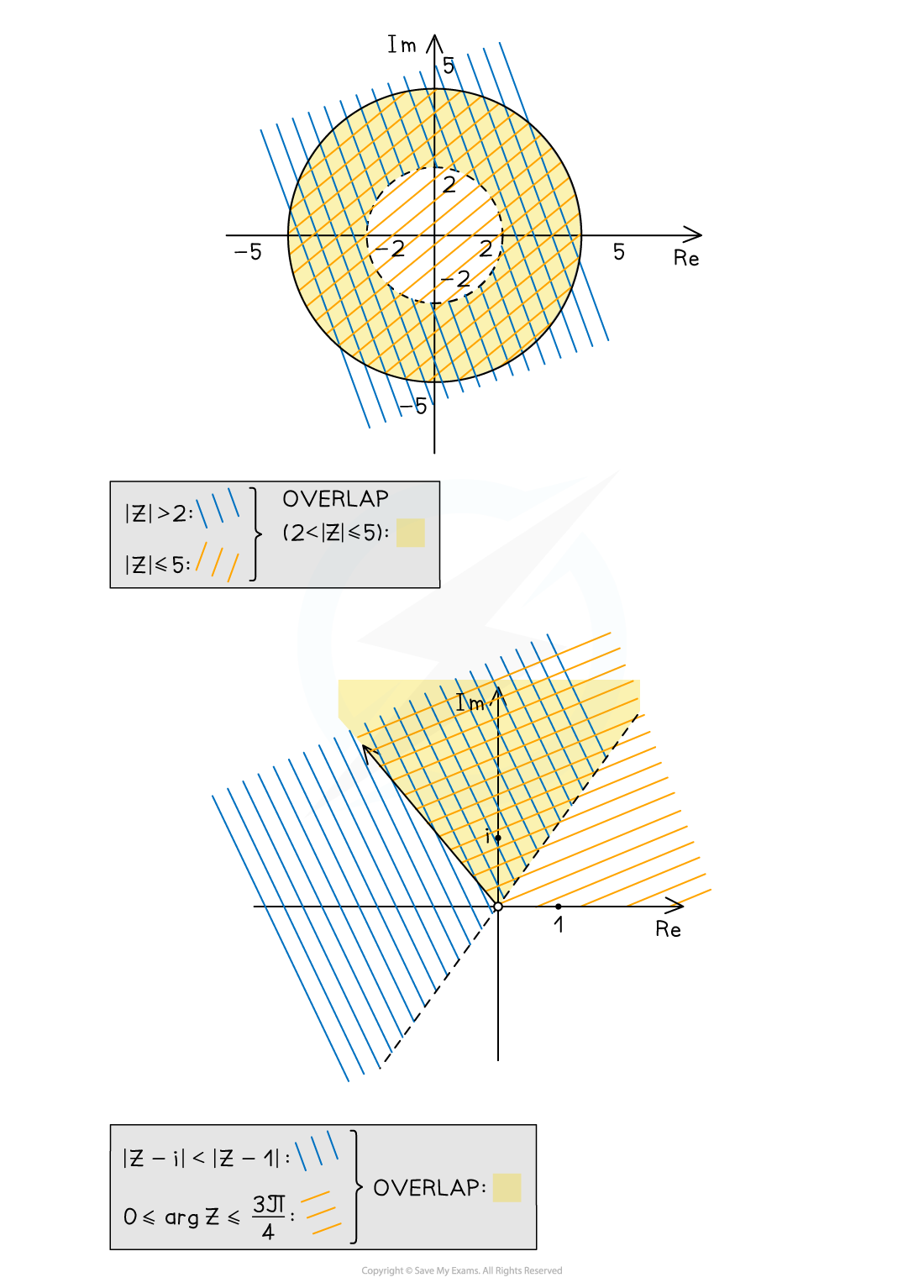
The inequalities $2 < | z | \leq 5$ on the left, the inequalities $| z - i | < | z - 1 |$ and $0 \leq \arg z < \frac{3 π}{4}$ on the right

How do I find the greatest (or least) values of $| z |$ or $\arg z$ in a region?

Every complex number, $z$ , that lies within a region on an Argand diagram will have its own modulus, $|z|$ , that is measured from the origin
For any shaped region…
- The least value of $| z |$ is the distance from the origin to the nearest point in the region
- The greatest value of $| z |$ is the distance from the origin to the farthest point in the region
- Sometimes the least value is zero (if the origin is in the region) and/or the greatest value is infinite (if the region is unbounded)
For circular regions that do not contain the origin…
- Find the length from the origin to the centre of the circle…
- then add a radius for the greatest value of $| z |$ or subtract a radius for the least value of $| z |$
Every complex number, $z$ , that lies within a region on an Argand diagram will have its own argument, $\arg z$ , as measured from the origin
For any shaped region…
- The least value of is $\arg z$ the smallest angle a line through the origin can make to a point in the region
- The greatest value of $\arg z$ is the largest angle a line through the origin can make to a point in the region
For circular regions that do not contain the origin…
- Find $θ$ , the argument of the centre…
- Find $α$ , the angle between a tangent to the circle through the origin and the line from the origin to the centre…
  - This can be done using trigonometry, as a radius meets a tangent at right angles
- The greatest value of $\arg z$ is $θ + α$ and the least value of $\arg z$ is $θ - α$

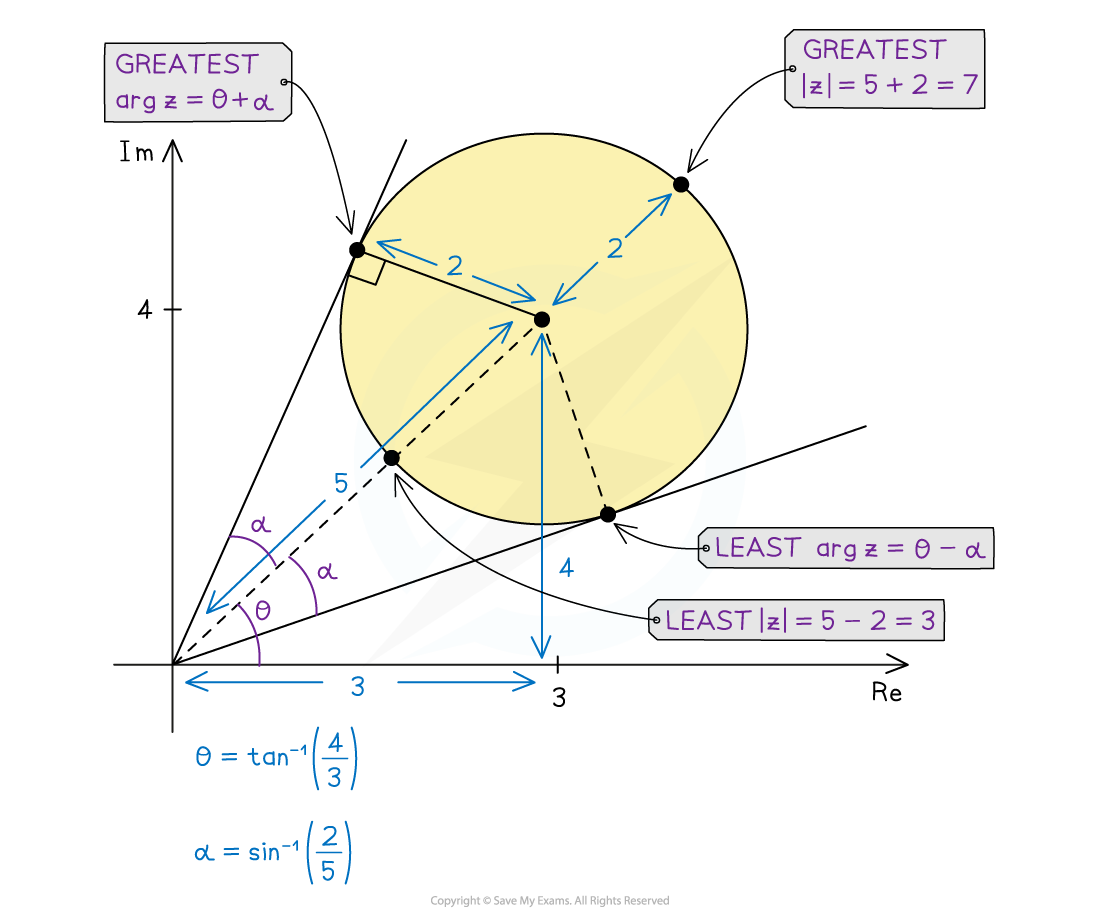
8-2-5_notes_fig6

Find greatest and least values of $|z|$ and $\arg z$

Examiner Tip

When shading an unbounded region in the exam, make sure you extend your shading outwards, crossing any axes where necessary.
When shading multiple inequalities to find a common region, lots of methods are accepted; these include lightly shading individual regions to see where they overlap, or starting to shade along the boundaries of individual regions to find the common region, or marking individual regions to find the region with multiple marks, etc.

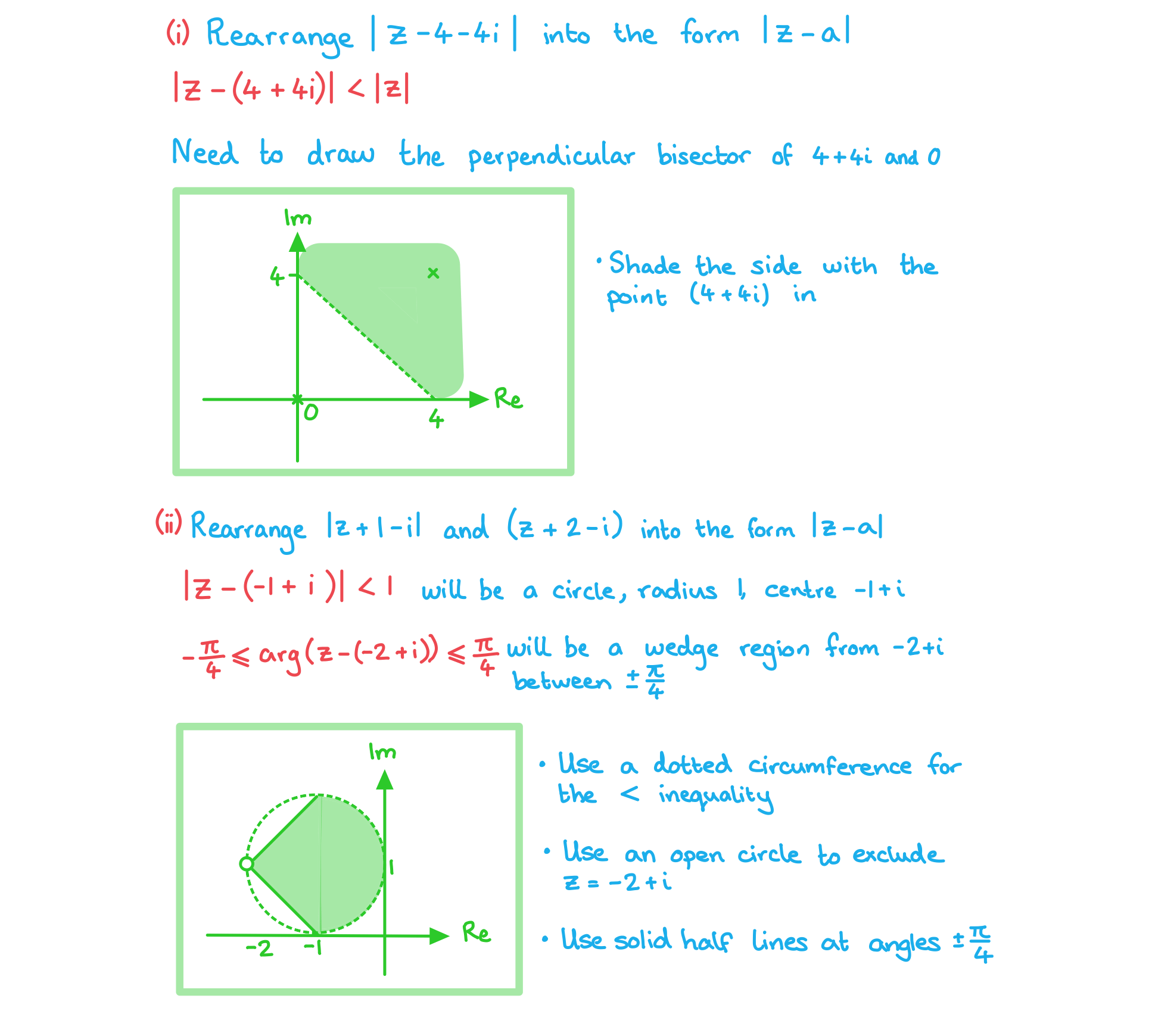
Worked example

On separate Argand diagrams, shade the region whose points represent complex numbers

z

satisfying

(i) the inequality

|z - 4 - 4 i| < |z|

(ii) both inequalities

|z + 1 - i| < 1

and

- \frac{π}{4} \leq \arg (z + 2 - i) \leq \frac{π}{4}

al-fm-1-1-6-regions-in-argand-diagrams-we-solution-a

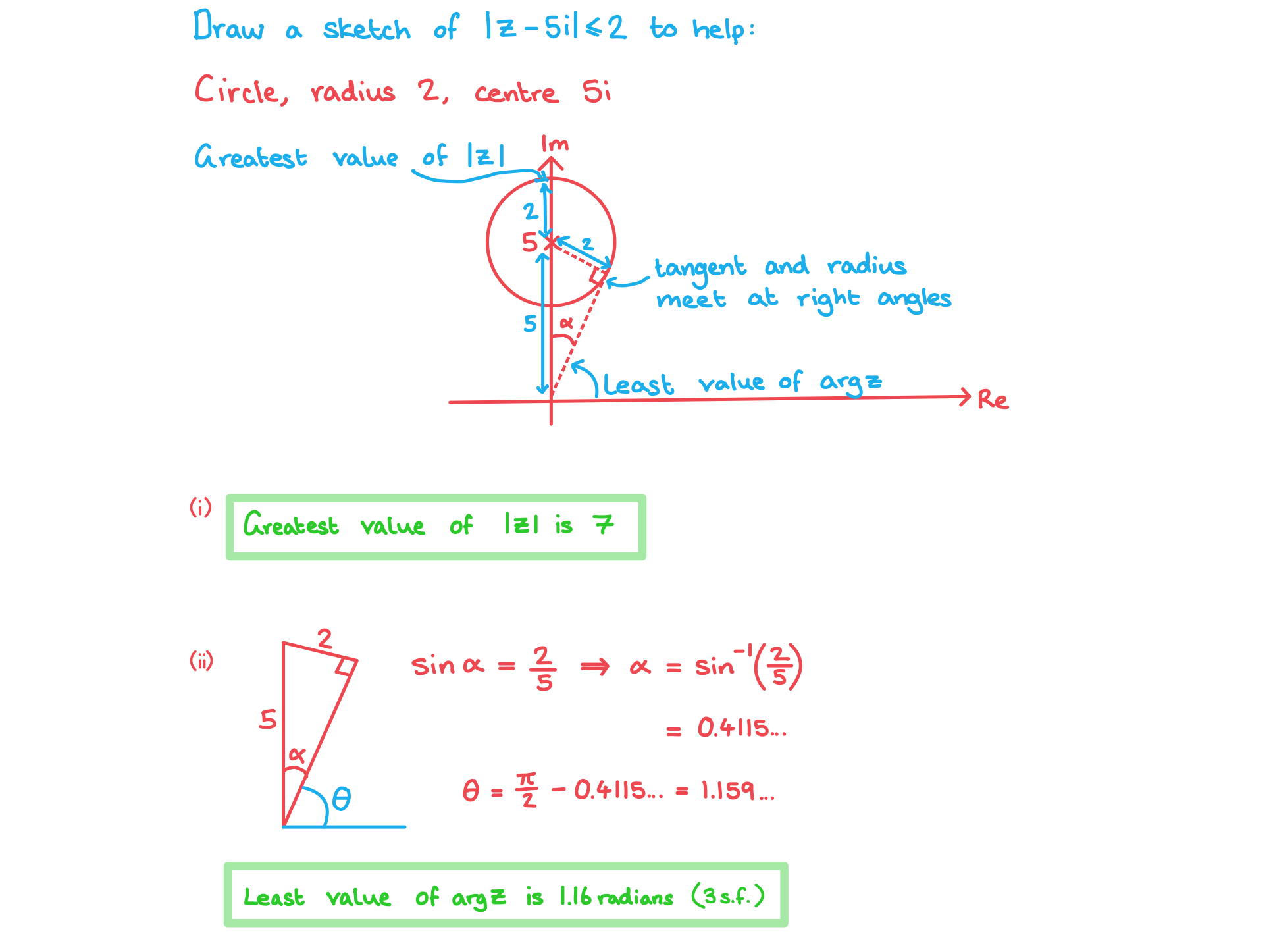
For complex numbers

z

satisfying the inequality

|z - 5 i| \leq 2

, find

(i) the greatest value of

|z|

(ii) the least value of

\arg (z)

al-fm-1-1-6-regions-in-argand-diagrams-we-solution-b-png

Regions in Argand Diagrams (Edexcel A Level Further Maths: Core Pure)

Revision Note

How do I sketch inequalities such as or on an Argand diagram?

How do I sketch inequalities such as on an Argand diagram?

How do I sketch inequalities such as on an Argand diagram?

How do I sketch inequalities such as on an Argand diagram?

How do I draw multiple inequalities on the same Argand diagram?

How do I find the greatest (or least) values of or in a region?

You've read 0 of your 5 free revision notes this week

Sign up now. It’s free!

Join the 100,000+ Students that ❤️ Save My Exams

1. Complex Numbers

1.1 Complex Numbers & Argand Diagrams

1.1.1 Introduction to Complex Numbers

1.1.2 Solving Equations with Complex Roots

1.1.3 Modulus & Argument

1.1.4 Modulus-Argument Form

1.1.5 Loci in Argand Diagrams

1.1.6 Regions in Argand Diagrams

1.2 Exponential Form & de Moivre's Theorem

1.2.1 Exponential Form

1.2.2 de Moivre's Theorem

1.2.3 Applications of de Moivre's Theorem

1.2.4 Roots of Complex Numbers

2. Matrices

2.1 Properties of Matrices

2.1.1 Introduction to Matrices

2.1.2 Determinants of Matrices

2.1.3 Inverses of Matrices

2.2 Transformations using Matrices

2.2.1 Transformations using a Matrix

2.2.2 Geometric Transformations with Matrices

2.2.3 Invariant Points & Lines

3. Further Algebra & Functions

3.1 Roots of Polynomials

3.1.1 Roots of Polynomials

3.1.2 Linear Transformations of Roots

3.2 Series

3.2.1 Sums of Integers, Squares & Cubes

3.2.2 Method of Differences

3.3 Maclaurin Series

3.3.1 Maclaurin Series

4. Hyperbolic Functions

4.1 Hyperbolic Functions

4.1.1 Hyperbolic Functions & Graphs

4.1.2 Logarithmic Forms of Inverse Hyperbolic Functions

4.1.3 Hyperbolic Identities & Equations

4.1.4 Differentiating & Integrating Hyperbolic Functions

5. Further Calculus

5.1 Volumes of Revolution

5.1.1 Volumes of Revolution

5.1.2 Modelling with Volumes of Revolution

5.2 Methods in Calculus

5.2.1 Improper Integrals

5.2.2 Mean Value of a Function

5.2.3 Integrating with Partial Fractions

5.2.4 Calculus involving Inverse Trig

5.2.5 Integration by Substitution

6. Further Vectors

6.1 Vector Lines

6.1.1 Equations of Lines in 3D

6.1.2 Pairs of Lines in 3D

6.1.3 Angle between Lines

6.1.4 Shortest Distances - Lines

6.2 Vector Planes

6.2.1 Equations of planes

6.2.2 Combinations of Lines & Planes

6.2.3 Combinations of Planes

6.2.4 Shortest Distances - Planes

7. Polar Coordinates

7.1 Polar Coordinates

7.1.1 Polar Coordinates

7.1.2 Calculus with Polar Coordinates

8. Differential Equations

8.1 First Order Differential Equations

8.1.1 Intro to Differential Equations

8.1.2 Solving First Order Differential Equations

8.1.3 Modelling using First Order Differential Equations

8.2 Second Order Differential Equations

8.2.1 Solving Second Order Differential Equations

How do I sketch inequalities such as $Re z < k$ or $Im z < k$ on an Argand diagram?

How do I sketch inequalities such as $|z - a| < k$ on an Argand diagram?

How do I sketch inequalities such as $| z - a | < | z - b |$ on an Argand diagram?

How do I sketch inequalities such as $α < \arg (z - a) < β$ on an Argand diagram?

How do I find the greatest (or least) values of $| z |$ or $\arg z$ in a region?