A LevelFurther MathsEdexcelFurther Statistics 1Revision NotesHypothesis TestingQuality of TestsSize & Power of Test

Size & Power of Test (Edexcel A Level Further Maths: Further Statistics 1): Revision Note

Exam code: 9FM0

Written by: Dan Finlay

Reviewed by: Lucy Kirkham

Updated on 2 July 2026

Size

What is the size of a test?

The size of a test is the probability of rejecting H₀ when it was in fact true
- P(in critical region | H₀ is true)
- The situation being described is not a good outcome
  - Something has been rejected when it was actually true!
- A better test has a smaller size
  - You want to minimise this error happening
Size is related to the significance level, α%
- A better test has a smaller significance level (e.g. 1%)
- For continuous distributions (e.g. normal)
  - Size = significance level, α
  - You can often write this down with no calculation
- For discrete distributions (e.g. binomial, Poisson, geometric)
  - Size = actual significance level (≤α)
  - As close to α% as a discrete variable can get, whilst still being critical

How does size relate to Type I errors?

The size is exactly the same as the probability of a Type I error
- Both want to know the probability of rejecting H₀ when it was in fact true

Worked Example

A student wants to test, at a 10% significant level, whether a coin is biased towards heads by counting the number of heads in 20 flips of the coin.

Calculate the size of this test.

Power

What is the power of a test?

The power of a test is the probability of rejecting H₀ when it was false
- P(in critical region | H₀ is false)
- The situation being described has a good outcome
  - The null hypothesis was false and it rightly got rejected
- A better test has a higher power
  - You want to maximise this happening
In practice, you need to be given the actual population parameter to calculate the power
- For example, H₀ assumed $p = \frac{1}{2}$ but actually $p = \frac{1}{3}$
  - This is more helpful than just saying $p \neq \frac{1}{2}$
- Power is P(in the critical region | actual population parameter)

How does power relate to Type II errors?

The power of a test is 1 - P(Type II error)
- Power is when H₀ is false and it gets rejected
  - That's a good outcome
- A Type II error is when H₀ is false and it does not get rejected
  - That's a bad outcome
You ideally want the power of a test to be greater than 0.5
- That way it's less likely to produce a Type II error
  - And more likely to reach the correct conclusion

Worked Example

Let $X ~ Po (λ)$ . A hypothesis test is conducted at the 5% significance level in which $H_{0} : λ = 8$ and $H_{1} : λ < 8$ .

If is later discovered that $λ = 6$ , find the power of the test.

Power functions

What is a power function?

The power function is the power of a test written algebraically
- In terms of $p$ or $λ$
- For when you're not given the actual population parameter in the question
In reality, it's very unlikely you'll know the actual population parameter anyway
- Otherwise you wouldn't be doing a hypothesis test on it!
- Power functions don't need this information
The power function is P(in the critical region | population parameter is $p$ )
- Or, for Poisson
  - P(in the critical region | population parameter is $λ$ )

How do I find power functions?

It's easier to show in an example
- If the critical region is $X \leq 2$ for a binomial hypothesis test with $n = 50$
- Then the power function is P(in the critical region | population parameter is $p$ )
  - Let $X ~ B (50, p)$
  - $P (X \leq 2 | p) = (\begin{matrix} 50 \\ 0 \end{matrix}) p^{0} {(1 - p)}^{50} + (\begin{matrix} 50 \\ 1 \end{matrix}) p^{1} {(1 - p)}^{49} + (\begin{matrix} 50 \\ 2 \end{matrix}) p^{2} {(1 - p)}^{48}$
- Simplify
  - ${(1 - p)}^{50} + 50 p {(1 - p)}^{49} + 1225 p^{2} {(1 - p)}^{48}$
- Factorise and collect like terms
  - The power function is ${(1 - p)}^{48} (1 + 48 p + 1176 p^{2})$

What can I do with power functions?

You can plot them against $p$ (or $λ$ )
- You can then see where the power is biggest
You can input different values of $p$ (or $λ$ )
- To compare two (or more) different hypothesis tests
  - The better test is the one with the higher power
- To check if the power of a test is greater than 0.5
  - So that it's more likely to reach the correct conclusion
  - And less likely to produce a Type II error
  - Because Power = 1 - P(Type II error)

Worked Example

Residents suspect that the number of accidents on a main road has decreased. They test, at a 5% significance level, the hypotheses $H_{0} : λ = 9$ and $H_{1} : λ < 9$ .

a) $\begin{matrix} \end{matrix}$ Show that the power function is $\frac{1}{6} e^{- λ} (a + b λ + c λ^{2} + d λ^{3})$ , where $a, b, c$ and $d$ are integers to be found.

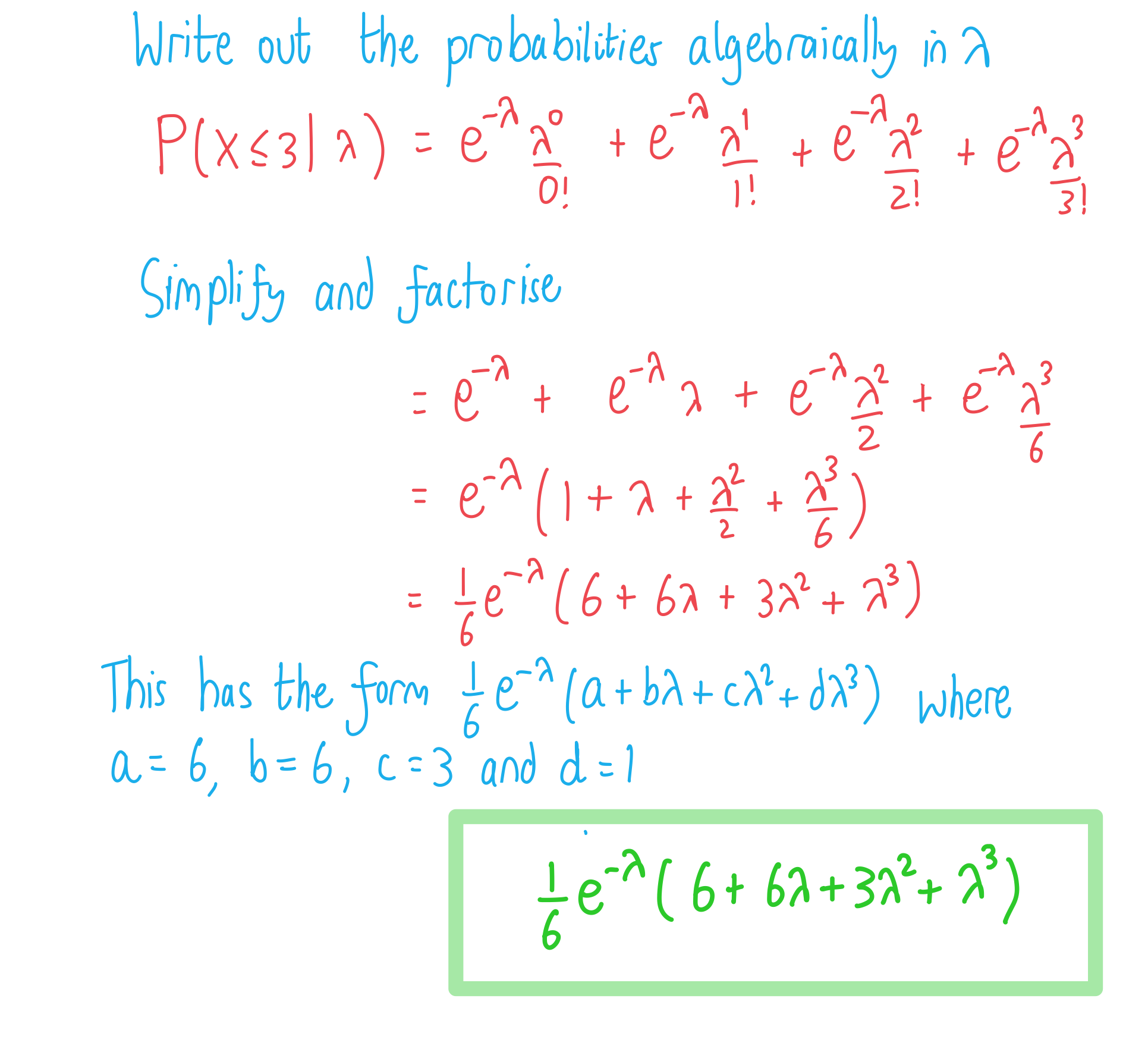
b) Find the largest integer value of $λ$ for which the probability of a Type II error is less than 20%.

Unlock more, it's free!

Join the 100,000+ Students that ❤️ Save My Exams

the (exam) results speak for themselves:

I would just like to say a massive thank you for putting together such a brilliant, easy to use website.I really think using this site helped me secure my top gradesin science and maths. You really did save my exams! Thank you.

Beth
IGCSE Student

This website is soooo useful and I can’t ever thank you enough for organising questions by topic like this. Furthermore, the name of the website could not have been more appropriate as it literally did SAVE MY EXAMS!

Fathima
A Level Student

Incredible! SO worth my money, the revision notes have everything I need to know and are so easy to understand. I actually enjoy revising! It makes me feel a lot more confident for my GCSEs in a few months.

Kate
GCSE Student

Absolutely brilliant, both my girls used it for A levels and GCSE. It's saves on paper copies, also beneficial exam questions ranked from easy to hard. It's removed a lot of stress from the exams.

Sameera
Parent

Just to say that your resources are the best I have seen and I have been teaching chemistry at different levels for about 40 years

Mark
Chemistry Teacher

Excellent

Size & Power of Test (Edexcel A Level Further Maths: Further Statistics 1): Revision Note

Size

What is the size of a test?

How does size relate to Type I errors?

Worked Example

Power

What is the power of a test?

How does power relate to Type II errors?

Worked Example

Power functions

What is a power function?

How do I find power functions?

What can I do with power functions?

Worked Example

Unlock more, it's free!

Join the 100,000+ Students that ❤️ Save My Exams

Author: Dan Finlay

Reviewer: Lucy Kirkham