Modulus-Argument Form (Edexcel A Level Further Maths) : Revision Note
Modulus-Argument Form
The complex number is said to be in Cartesian form. There are, however, other ways to write a complex number, such as in modulus-argument (polar) form.
How do I write a complex number in modulus-argument (polar) form?
The Cartesian form of a complex number,
, is written in terms of its real part,
, and its imaginary part,
If we let
and
, then it is possible to write a complex number in terms of its modulus,
, and its argument,
, called the modulus-argument (polar) form, given by...
It is usual to give arguments in the range
Negative arguments should be shown clearly, e.g.
without simplifying
to either
or
Occasionally you could be asked to give arguments in the range
If a complex number is given in the form
, then it is not currently in modulus-argument (polar) form due to the minus sign, but can be converted as follows…
By considering transformations of trigonometric functions, we see that
and
Therefore
can be written as
, now in the correct form and indicating an argument of
To convert from modulus-argument (polar) form back to Cartesian form, evaluate the real and imaginary parts
E.g.
becomes

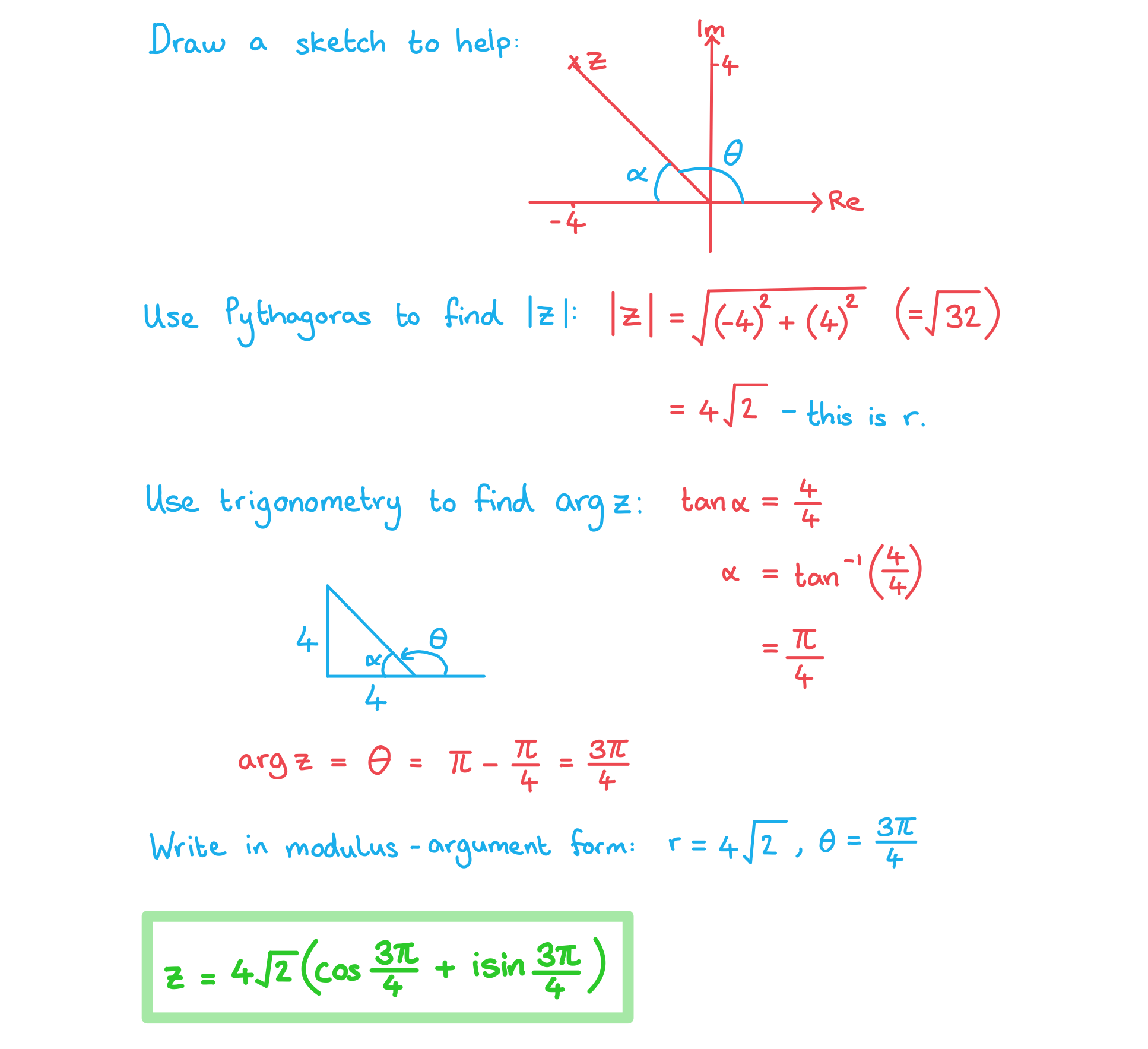
Worked Example
Write in the form
where
and
are exact.
Operations using Modulus-Argument Form
What are the rules for moduli and arguments under multiplication and division?
When two complex numbers,
and
, are multiplied to give
, their moduli are also multiplied
When two complex numbers,
and
, are divided to give
, their moduli are also divided
When two complex numbers,
and
, are multiplied to give
, their arguments are added
When two complex numbers,
and
, are divided to give
, their arguments are subtracted
How do I multiply complex numbers in modulus-argument (polar) form?
The main benefit of writing complex numbers in modulus-argument (polar) form is that they multiply and divide very easily (often quicker than when in Cartesian form)
To multiply two complex numbers,
and
, in modulus-argument (polar) form we use the rules from above to multiply their moduli and add their arguments
So if
and
then the rules above give…
Sometimes the new argument,
, does not lie in the range
(or
if this is being used)
An out-of-range argument can be adjusted by either adding or subtracting
E.g. If
and
then
This is currently not in the range , but by subtracting
from
to give
, a new argument is formed that lies in the correct range and represents the same angle on an Argand diagram
The rules of multiplying the moduli and adding the arguments can also be applied when…
…multiplying three complex numbers together,
, or more
…finding powers of a complex number (e.g.
can be written as
)
Whilst not examinable, the rules for multiplication can be proved algebraically by multiplying
by
, expanding the brackets and using compound angle formulae
How do I divide complex numbers in modulus-argument (polar) form?
To divide two complex numbers,
and
in modulus-argument (polar) form, we use the rules from above to divide their moduli and subtract their arguments
So if
and
then the rules above give…
As with multiplication, sometimes the new argument,
, can lie out of the range
(or the range
if this is being used)
You can add or subtract
to bring out-of-range arguments back in range
Whilst not examinable, the rules for division can be proved algebraically by dividing
by
, using complex division and compound angle formulae
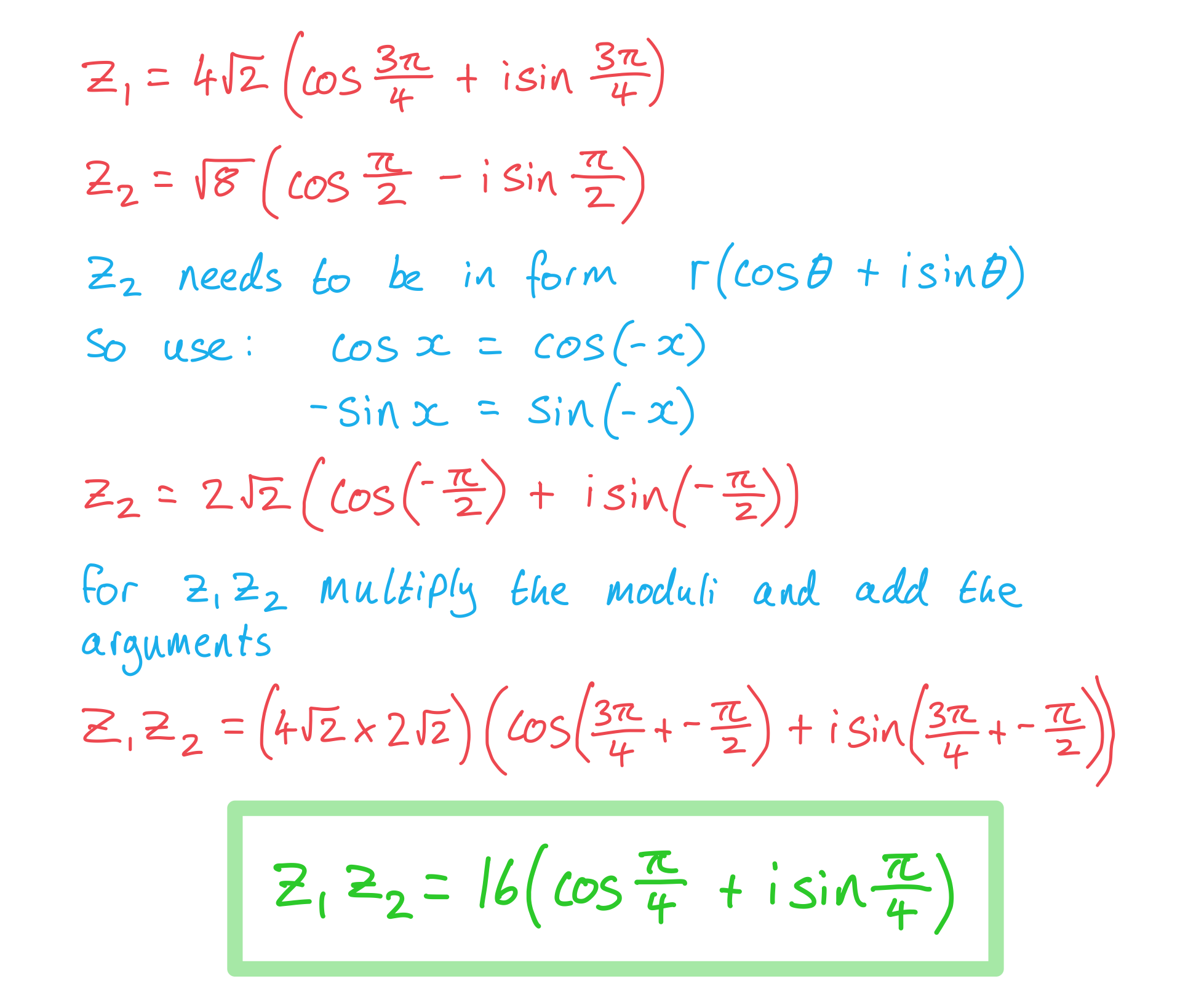
Worked Example
Let z1=42cos3π4+isin3π4 {"language":"en","fontFamily":"Times New Roman","fontSize":"18"} and z2=8cosπ2-isinπ2{"language":"en","fontFamily":"Times New Roman","fontSize":"18"}
a) Find z1z2{"language":"en","fontFamily":"Times New Roman","fontSize":"18"}, giving your answer in the form rcosθ+isinθ{"language":"en","fontFamily":"Times New Roman","fontSize":"18"} where 0≤θ<2π{"language":"en","fontFamily":"Times New Roman","fontSize":"18"}
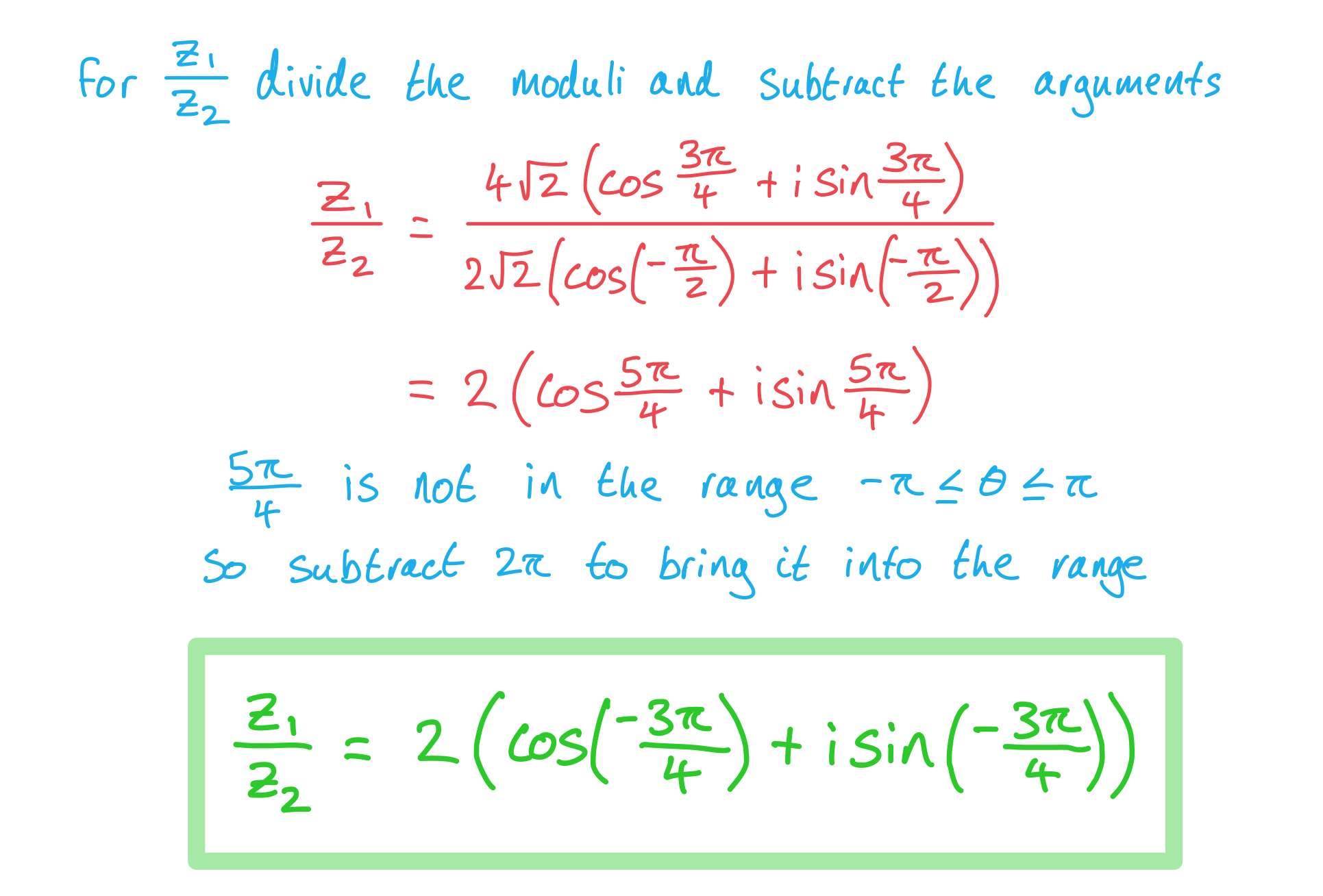
b) Find z1z2{"language":"en","fontFamily":"Times New Roman","fontSize":"18"}, giving your answer in the form rcosθ+isinθ{"language":"en","fontFamily":"Times New Roman","fontSize":"18"} where -π≤θ<π{"language":"en","fontFamily":"Times New Roman","fontSize":"18"}

You've read 0 of your 5 free revision notes this week
Sign up now. It’s free!
Did this page help you?
