Marginal, Average & Total Revenue (AQA A Level Economics)

• Total revenue is the total value of all sales a firm incurs

      
 

• Average revenue is the overall revenue per unit

• Marginal revenue is the extra revenue received from the sale of an additional unit of output

• The relationship between TR, AR & MR is different in perfect competition and imperfect competition

Revenue in perfect competition

The Relationship Between TR, AR & MR in Perfect Competition

P (£)	Q	TR	AR	MR
8	5	40	8	8
8	6	48	8	8
8	7	56	8	8
8	8	64	8	8

• The situation in the table above is illustrated in the diagram below

Diagram: The Relationship Between Average Revenue & Marginal Revenue

An illustration of the relationship between AR, MR & TR in a perfectly competitive market

Observations

•  The firm is a price taker at P₁ (£8)
  • Every unit of output is sold at the same price
  • A higher price would decrease sales to zero
  • A lower price would result in all sellers lowering their price
• TR increases at a constant rate
• MR = AR = Demand

Revenue in imperfect competition

The Relationship Between TR, AR & MR for Imperfect Competition

P (£)	Q	TR	AR	MR
8	1	8	8	8
7	2	14	7	6
6	3	18	6	4
5	4	20	5	2
4	5	20	4	0
3	6	18	3	-2
2	7	14	2	-4
1	8	8	1	-6

• The situation in the table above is illustrated in the diagram below

Diagram: Average Revenue & Marginal Revenue in Imperfect Competition

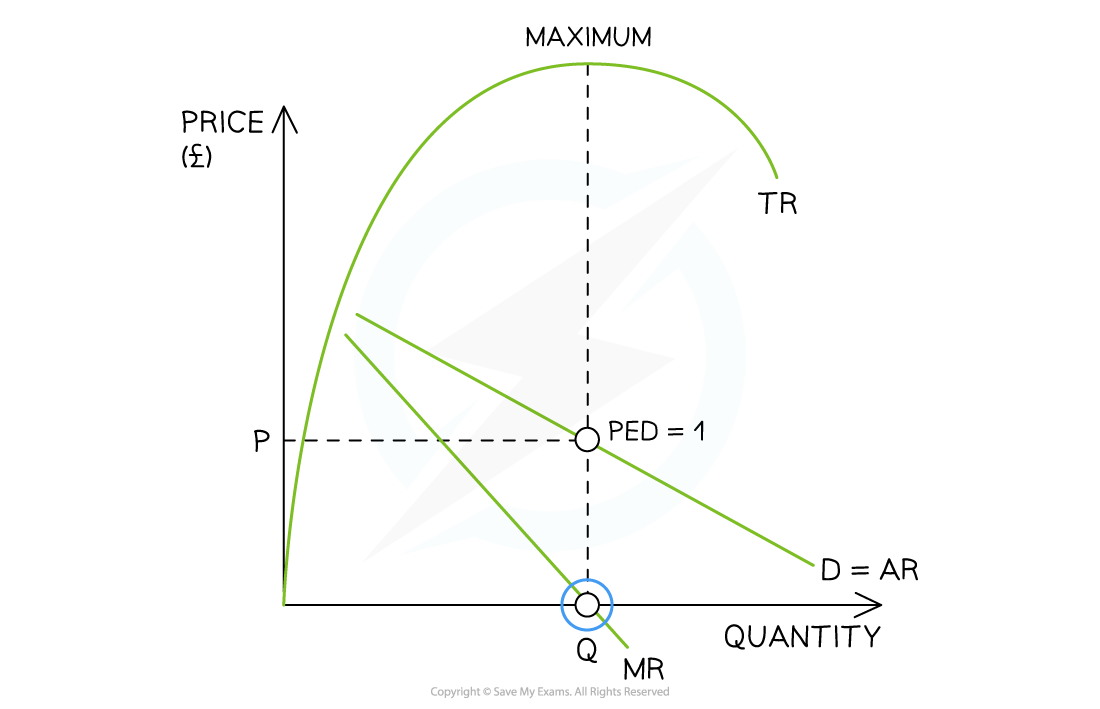
An illustration of the relationship between AR, MR & TR for imperfect competition

Observations

•  The firm is a price maker 
  • In order to sell an additional unit of output, the price (AR) must be lowered
  • Both AR and MR fall with additional units of sale
  • When the AR falls, the MR falls by twice as much
    • The gradient of the MR curve is twice as steep as the AR curve
  • TR is maximised when MR = 0
• AR is the demand (D) curve
• From the point where MR = 0, the total revenue begins to fall