Rates - Activation Energy (OCR A Level Chemistry A): Revision Note

PAG 10.3: Rates – Activation energy

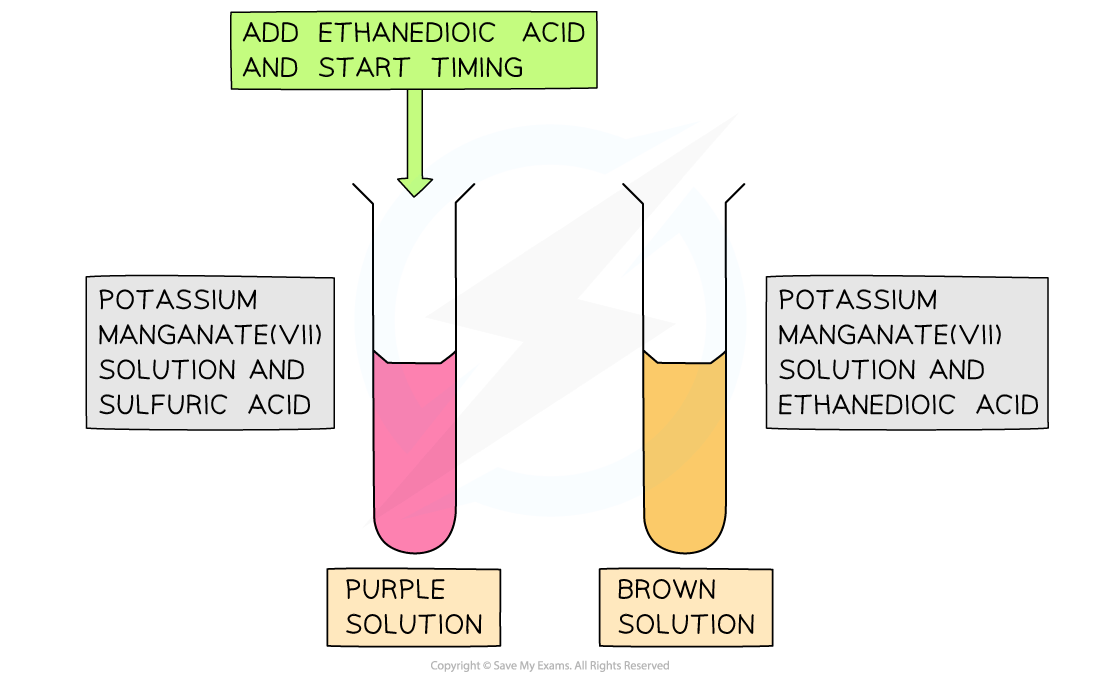
Ethanedioic acid and potassium manganate(VII)

• This experiment investigates the effect of temperature on rate using potassium manganate(VII) and ethanedioic acid in acidic solution:

2MnO₄^– (aq) + 5H₂C₂O₄ (aq) + 6H⁺ (aq) → 2Mn²⁺ (aq) + 8H₂O (l) + 10CO₂ (g)

• The key observable change is a colour change from purple to pale brown as Mn⁷⁺ is reduced to Mn²⁺

Method

1. Using a different / clean measuring cylinder for each chemical, transfer 10.0 cm³ of each of the following into a separate boiling tube

   • 0.1 mol dm^-3 potassium manganate(VII) solution, KMnO₄ (aq) 

   • 2.0 mol dm^-3 sulfuric acid, H₂SO₄ (aq)

   • 0.25 mol dm^-3 ethanedioic acid, H₂C₂O₄ (aq)

2. Warm all three boiling tubes in a water bath to the same temperature

3. Record the temperature of each solution

4. Into a clean test tube, add in order:

   • 0.5 cm³ potassium manganate(VII) solution

   • 0.5 cm³sulfuric acid

   • 1.0 cm³ ethanedioic acid

5. Immediately start the stopwatch and swirl the mixture

6. Stop the stopwatch when the purple colour changes to pale brown

7. Record the time

8. Repeat the experiment at least four more times at different temperatures

Specimen results

• The temperature of the reaction is converted to 1 / T by calculating the reciprocal value

• The rate is found by calculating the reciprocal of time, t

• This is then converted into the natural logarithm function to give ln(1 / t) or ln(rate)

• A graph of ln(rate) versus 1 / T is then plotted:

Analysis

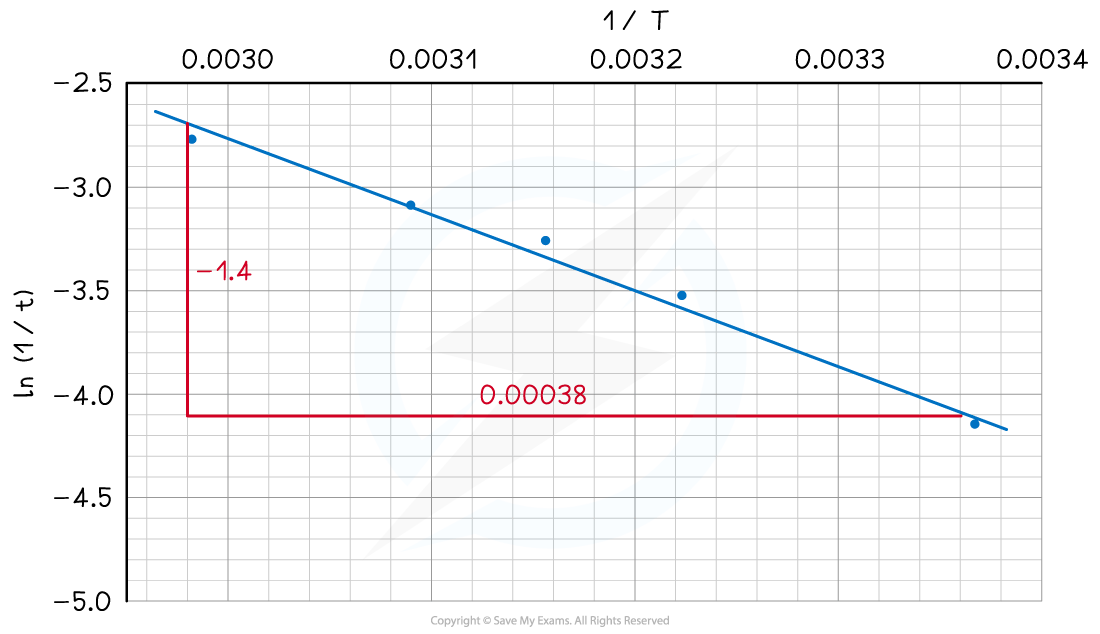
• From this graph, we can see that the line of best fit is a straight line

  • The equation for a straight line is y = mx + c

  • The Arrhenius equation can be rearranged to ln k = ln A - E_a/RT

  • Therefore, the gradient of the line is -E_a / R 

• This means that we can use the gradient from the graph to calculate the activation energy for this reaction:

Gradient =  can be rearranged to E_a = –gradient x R

E_a = –x 8.31 = 30616 J mol^-1

E_a = = 30.616 kJ mol^-1

Practical skills reminder

• This practical develops key skills in monitoring reaction rate through visible colour change and temperature control.

• It also supports:

  • Accurately measuring volumes and timing using simple lab apparatus

  • Controlling temperature using a water bath

  • Plotting ln(rate) against 1/T to determine activation energy from the gradient of a straight line