Buffers (Edexcel A Level Chemistry)

• A buffer solution is a solution which resists changes in pH when small amounts of acids or alkalis are added
  • A buffer solution is used to keep the pH almost constant
  • A buffer can consists of weak acid - conjugate base or weak base - conjugate acid

Ethanoic acid & sodium ethanoate as a buffer

• A common buffer solution is an aqueous mixture of ethanoic acid and sodium ethanoate
• Ethanoic acid is a weak acid and partially ionises in solution to form a relatively low concentration of ethanoate ions

• Sodium ethanoate is a salt which fully ionises in solution

• There are reserve supplies of the acid (CH₃COOH) and its conjugate base (CH₃COO^-)
  • The buffer solution contains relatively high concentrations of CH₃COOH (due to partial ionisation of ethanoic acid) and CH₃COO^- (due to full ionisation of sodium ethanoate)

• In the buffer solution, the ethanoic acid is in equilibrium with hydrogen and ethanoate ions

• When H⁺ ions are added:
  • The equilibrium position shifts to the left as H⁺ ions react with CH₃COO^- ions to form more CH₃COOH until equilibrium is re-established
  • As there is a large reserve supply of CH₃COO^- the concentration of CH₃COO^- in solution doesn’t change much as it reacts with the added H⁺ ions
  • As there is a large reserve supply of CH₃COOH the concentration of CH₃COOH in solution doesn’t change much as CH₃COOH is formed from the reaction of CH₃COO^- with H⁺
  • As a result, the pH remains reasonably constant

When hydrogen ions are added to the solution the pH of the solution would decrease; However, the ethanoate ions in the buffer solution react with the hydrogen ions to prevent this and keep the pH constant

• When OH^- ions are added:
  • The OH^- reacts with H⁺ to form water

OH^- (aq) + H⁺  (aq) → H₂O (l)

• • The H⁺ concentration decreases
  • The equilibrium position shifts to the right and more CH₃COOH molecules ionise to form more H⁺and CH₃COO^- until equilibrium is re-established

CH₃COOH (aq) → H⁺ (aq) + CH₃COO^- (aq)

• • As there is a large reserve supply of CH₃COOH the concentration of CH₃COOH in solution doesn’t change much when CH₃COOH dissociates to form more H⁺ ions
  • As there is a large reserve supply of CH₃COO^- the concentration of CH₃COO^- in solution doesn’t change much
  • As a result, the pH remains reasonably constant

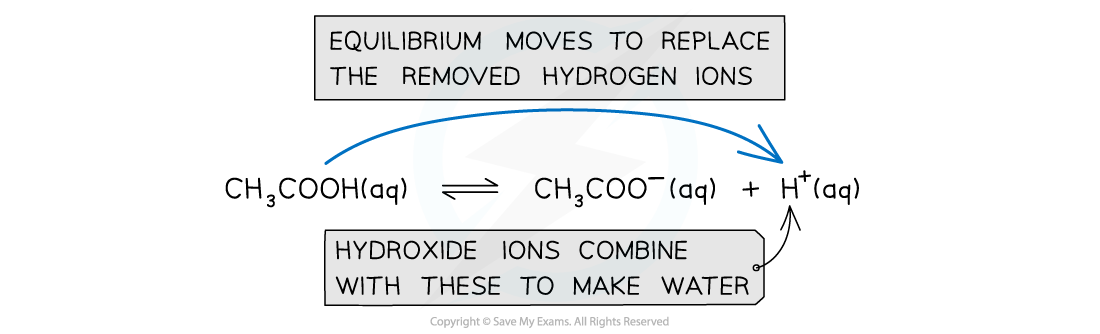
When hydroxide ions are added to the solution, the hydrogen ions react with them to form water; The decrease in hydrogen ions would mean that the pH would increase however the equilibrium moves to the right to replace the removed hydrogen ions and keep the pH constant

Answer: 

• B / NaOH (aq) and excess CH₃COOH (aq)

This is because:

• A buffer with a pH lower than 7 can be made from a weak acid and its salt or by partial neutralisation of a weak acid
• B contains a weak acid that is in excess and NaOH, so the weak acid will be partially neutralised leaving a solution that contains a weak acid and its conjugate base - this is a buffer solution
• All of the other options are incorrect because the solutions would not form a buffer

• The pH of a buffer solution can be calculated using:
  • The K_a of the weak acid
  • The equilibrium concentration of the weak acid and its conjugate base (salt)

• To determine the pH, the concentration of hydrogen ions is needed which can be found using the equilibrium expression

• To simplify the calculations, logarithms are used such that the expression becomes:

• Since -log₁₀ [H⁺] = pH, the expression can also be rewritten as:

• This is known as the Hendersen-Hasselbalch equation

Answer

Ethanoic acid is a weak acid that ionises as follows:

CH₃COOH (aq) ⇌ H⁺ (aq) + CH₃COO^- (aq)

Step 1: Write down the equilibrium expression to find K_a

Step 2: Rearrange the equation to find [H⁺]

Step 3: Substitute the values into the expression

   = 1.02 x 10-5 mol dm^-3

Step 4: Calculate the pH

   pH = - log [H+]

   = -log 1.02 x 10-5

   = 4.99

How to make a buffer solution with a required pH

• To make a buffer solution with a pH of less than 7, you need to use a mixture of a weak acid and its conjugate base
• Conversely, you can make a buffer solution with a pH greater than 7 by using a mixture of a weak base and its conjugate acid
• Imagine we want to make a buffer solution with a pH of 5.00 at a temperature of 298K
• This would require a hydrogen ion concentration of

      [H⁺_(aq)] = 1.00 x 10^-5 mol dm^-3

• The hydrogen ion concentration of a buffer solution of a weak acid and its conjugate base is calculated using the formula

• We will use ethanoic acid as our weak acid of choice

      K_a = 1.74 x 10^-5 mol dm^-3

• Substituting our known values into the equation we get

• This gives a value for the ratio of the concentrations of acid and base needed in our buffer solution

• Mixing an equal volume of ethanoic acid with a concentration of 0.575 mol dm^-3 and a sodium ethanoate solution of 1.00 mol dm^-3 would allow us to make this buffer solution
• This would give us a solution with an acid concentration of 0.2875 mol dm^-3 and a salt concentration of 0.500 mol dm^-3

Controlling the pH of blood

• In humans, HCO₃^- ions act as a buffer to keep the blood pH between 7.35 and 7.45
• Body cells produce CO₂ during aerobic respiration
• This CO₂ will combine with water in blood to form a solution containing H⁺ ions

CO₂ (g) + H₂O (l) ⇌ H⁺ (aq) + HCO₃^- (aq)

• This equilibrium between CO₂ and HCO₃^- is extremely important
• If the concentration of H⁺ ions is not regulated, the blood pH would drop and cause ‘acidosis’
  • Acidosis refers to a condition in which there is too much acid in the body fluids such as blood
  • This could cause body malfunctioning and eventually lead to coma

• If there is an increase in H⁺ ions
• The equilibrium position shifts to the left until equilibrium is restored

CO₂ (g) + H₂O (l) ⇌ H⁺ (aq) + HCO₃^- (aq)

• This reduces the concentration of H⁺ and keeps the pH of the blood constant
• If there is a decrease in H⁺ ions
  • The equilibrium position shifts to the right until equilibrium is restored

CO₂ (g) + H₂O (l) ⇌ H⁺ (aq) + HCO₃^- (aq)

• This increases the concentration of H⁺ and keeps the pH of the blood constant