Redox Titrations (AQA A Level Chemistry)

Redox Titrations

Redox Titrations

• Iron has variable oxidation states and this characteristic can be exploited in redox titrations of Fe²⁺ with MnO₄^- or Cr₂O₇^2-

• Both the manganate(VII) ion and dichromate(VI) ions can be used as strong oxidising agents to find the amount of iron(II) ions in solution

• You need to be able to perform these separate redox titrations with Fe²⁺ and understand the colour changes in each example

Potassium manganate(VII) titrations

• In these redox titrations the manganate(VII) is the oxidising agent and is reduced to Mn²⁺(aq)

• The iron is the reducing agent and is oxidised to Fe²⁺(aq) and the reaction mixture must be acidified, to excess acid is added to the iron(II) ions before the reaction begins

• The choice of acid is important, as it must not react with the manganate(VII) ions, so the acid normally used is dilute sulfuric acid

  • As it does not oxidise under these conditions and does not react with the manganate(VII) ions

• You could be asked why other acids are not suitable for this redox titration in the exam so make sure you understand the suitability of dilute sulfuric acid

Table explaining why other acids are not suitable for the redox titration

Indicator and end point

• Potassium permanganate acts as its own indicator, as the purple potassium permanganate solution is added to the titration flask from the burette and reacts rapidly with the Fe²⁺(aq)

  • The burette used in this practical should be one with white numbering not black, as you would struggle to read the values for your titres against the purple colour of the potassium permanganate if black numbering was used

• The manganese(II) ions, Mn²⁺(aq), have a very pale pink colour but they are present in such a low concentration that the solution looks colourless

• As soon as all of the iron(II), Fe²⁺(aq), ions have reacted with the added manganate(VII) ions, Mn⁷⁺(aq), a pale pink tinge appears in the flask due to an excess of manganate(VII) ions, Mn⁷⁺(aq)

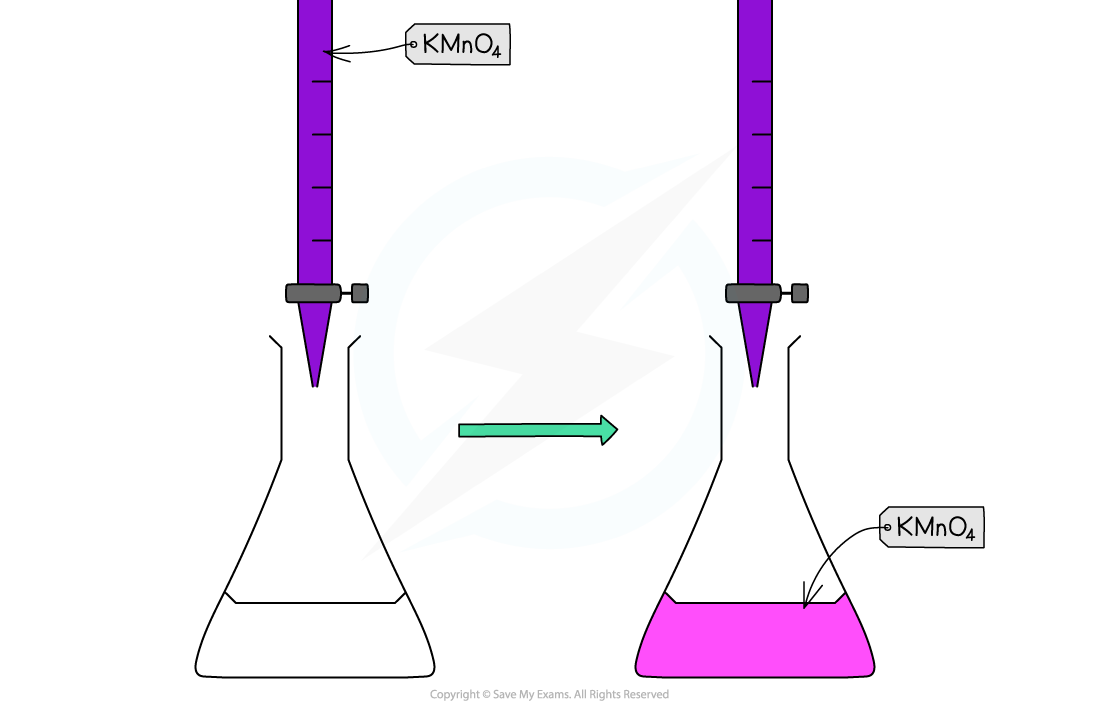
Redox titration colour change for potassium permanganate and iron(II) ions

Potassium dichromate(VI) titrations

• Dichromate(VI) is a powerful oxidising agent when it changes from +6 oxidation state in the dichromate(VI) ion, Cr₂O₇^2-, to the +3 oxidation state in Cr³⁺

• The two half equations are:

  • Cr₂O₇^2- (aq) + 14H⁺ (aq) + 6e^- → 2Cr³⁺ (aq) + 7H₂O (l)

  • 6Fe²⁺ (aq) → 6Fe³⁺ (aq) + 6e^-

• Overall equation is:

  • Cr₂O₇^2- (aq) + 14H⁺ (aq) + 6Fe²⁺ → 2Cr³⁺ (aq) + 6Fe³⁺ (aq) + 7H₂O (l)

Indicator and end point

• The colour change for this titration is from orange to bluish green

  • to give a more definitive and visible endpoint, the indicator sodium diphenylaminesulfonate is used

  • This turns from colourless to purple at the endpoint