The Ideal Gas Equation (AQA A Level Chemistry)

The Ideal Gas Equation

Kinetic theory of gases

• The kinetic theory of gases states that molecules in gases are constantly moving

• The theory makes the following assumptions:

  • That gas molecules are moving very fast and randomly

  • That molecules hardly have any volume

  • That gas molecules do not attract or repel each other (no intermolecular forces)

  • No kinetic energy is lost when the gas molecules collide with each other (elastic collisions)

  • The temperature of the gas is related to the average kinetic energy of the molecules

• Gases that follow the kinetic theory of gases are called ideal gases

• However, in reality gases do not fit this description exactly but may come very close and are called real gases

Ideal gases

• The volume that an ideal gas occupies depends on:

  • Its pressure

  • Its temperature

• When a gas is heated (at constant pressure) the particles gain more kinetic energy and undergo more frequent collisions with the container wall

• To keep the pressure constant, the molecules must get further apart and therefore the volume increases

• The volume is therefore directly proportional to the temperature (at constant pressure)

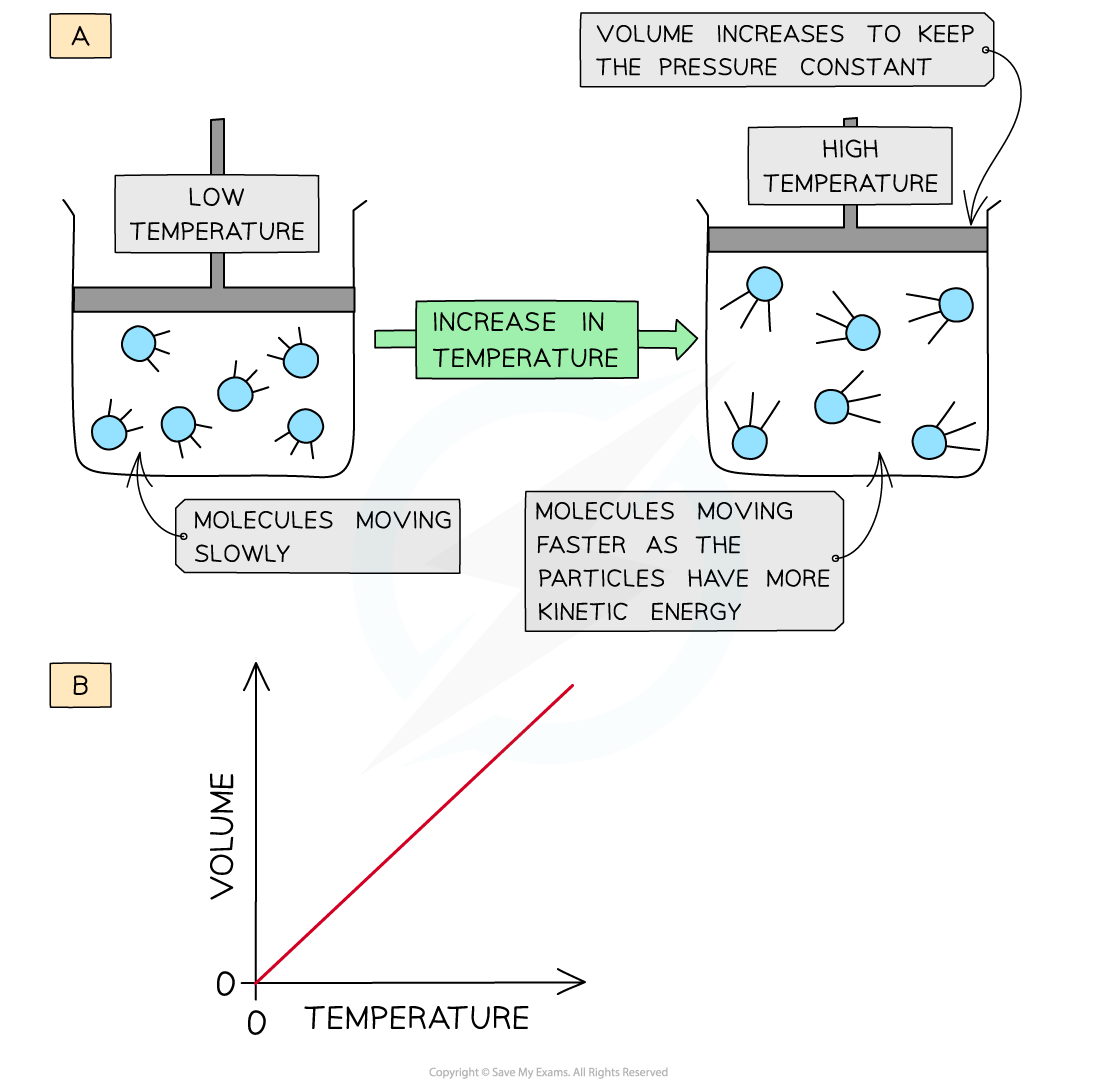
The volume of a gas increases upon heating to keep a constant pressure (a); volume is directly proportional to the temperature (b)

Limitations of the ideal gas law

• At very low temperatures and high pressures real gases do not obey the kinetic theory as under these conditions:

  • Molecules are close to each other

  • There are instantaneous dipole- induced dipole or permanent dipole- permanent dipole forces between the molecules

  • These attractive forces pull the molecules away from the container wall

  • The volume of the molecules is not negligible

• Real gases therefore do not obey the following kinetic theory assumptions at low temperatures and high pressures:

  • There is zero attraction between molecules (due to attractive forces, the pressure is lower than expected for an ideal gas)

  • The volume of the gas molecules can be ignored (volume of the gas is smaller than expected for an ideal gas)

Ideal gas equation

• The ideal gas equation shows the relationship between pressure, volume, temperature and number of moles of gas of an ideal gas:

PV = nRT

P = pressure (pascals, Pa)

V = volume (m³)

n = number of moles of gas (mol)

R = gas constant (8.31 J K^-1 mol^-1)

T = temperature (kelvin, K)