Mean & Standard Deviation (AQA A Level Biology)

Maths Skill: Calculating Mean Values & Standard Deviation

• Descriptive statistics are invaluable when interpreting data from experiments

• Some experiments have thousands or millions of data values/observations

• Descriptive statistics allow for sample data to be summarised in a concise manner

• Other statistics have different purposes such as:

  • Testing for a significant difference between means

  • Testing for correlation between variables

  • Investigating discrete data (data that falls into distinct categories)

Mean

• A mean value is what is usually meant by “an average” in biology

Mean = sum of all measurements ÷ number of measurements

• Problems with the mean occur when there are one or two unusually high (or low) values in the data (outliers) which can make the mean too high (or too low) to reflect any patterns in the data

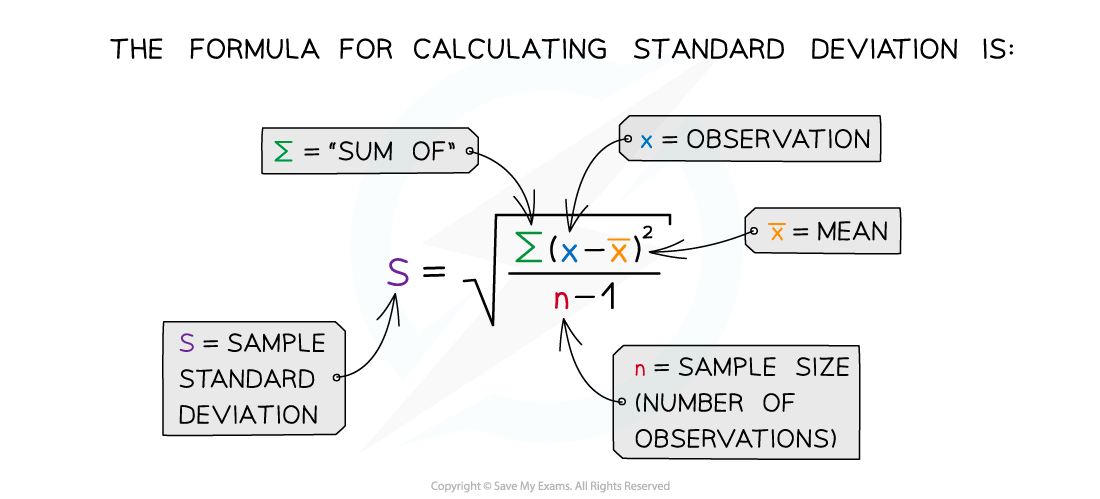
• The mean is sometimes referred to as X̄ in calculations

Standard Deviation

• The mean is a more informative statistic when it is provided alongside standard deviation

• Standard deviation measures the spread of data around the mean value

  • It is very useful when comparing consistency between different data sets

• The mean must be calculated before working out the standard deviation

Step 1: Calculate the mean

12 + 10 + 15 + 14 + 17 + 11 + 12 + 13 + 9 + 21 + 14 + 20 + 19 + 16 + 23 = 226

226 ÷ 15 = 15.067

Step 2: Round to 3 significant figures

Mean (X̄) = 15.1 seconds

Step 1: Calculate the mean

Mean = 885 ÷ 15 = 59 mm

Step 2: Find the difference between each value and the mean

Subtract the mean from each value to find the difference

Example: 62 - 59 = 3

Step 3: Square each difference

Square the difference for each value

Example: 3² = 9

Step 4: Total the differences

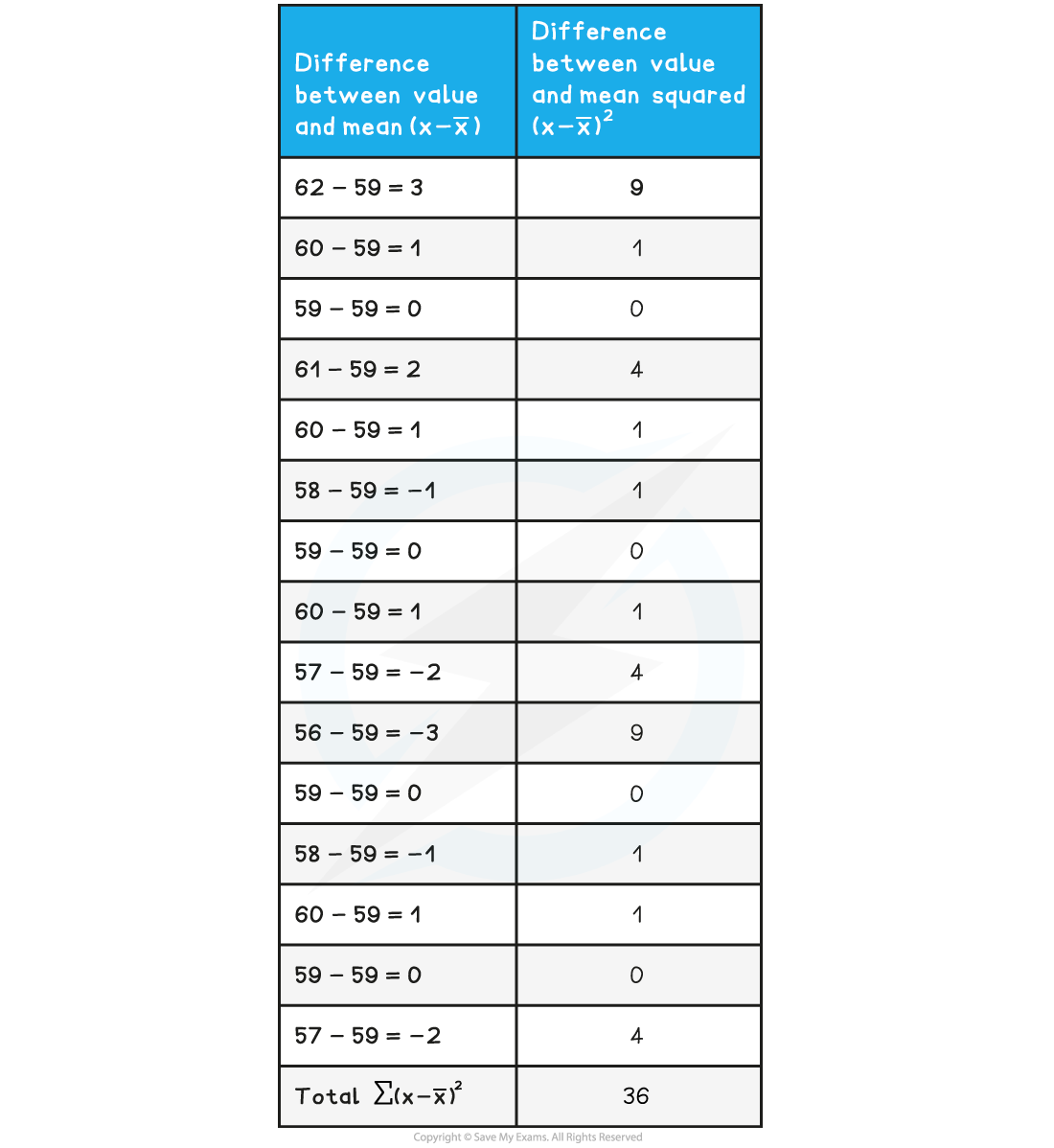
Step 5: Divide the total by (n-1) to get value A

36 ÷ (15 - 1) = 36 ÷ 14 = 2.571

Step 6: Get the square root of value A

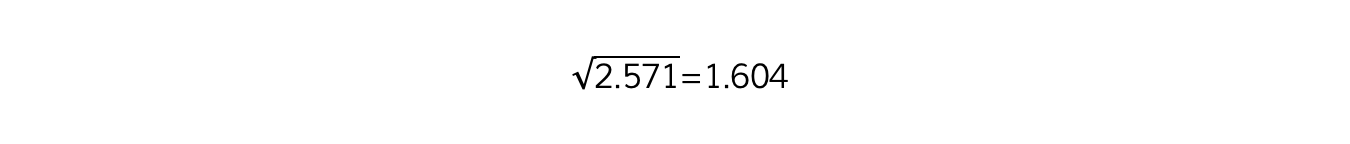
Standard Deviation = 1.60